Question
The sum of two digit number is 9, nine times of this number is twice the number obtained by reversing the order.find the number
Sir it's a humble request to u to upload videos and notes for soln of supplementary exercise of ncert textbook of maths .
This is because in supplementary part there are extra topics and important questions also
This is because in supplementary part there are extra topics and important questions also

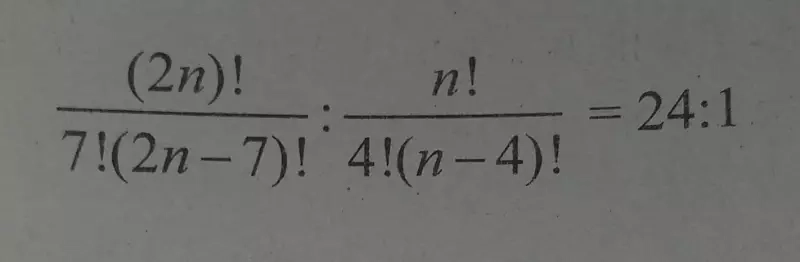

 Questions 1.
Questions 1.