Hi Jaswant,

The text version of your answer
Find the roots of the quadratic equation √3x2 – 2√2 x – 2√3 = 0
√3x2 – 2√2 x – 2√3 = 0
Comparing equation with ax2 + bx + c = 0
Here, a = √3, b = – 2√2 , c = –2√3
We know that
D = b2 – 4ac
= ("– 2" √2)^2– 4 ×(√3)×(-2√3)
= (4 ×2)+8(√3 ×√3)
= 8 + 8 × 3
= 8 + 24
= 32
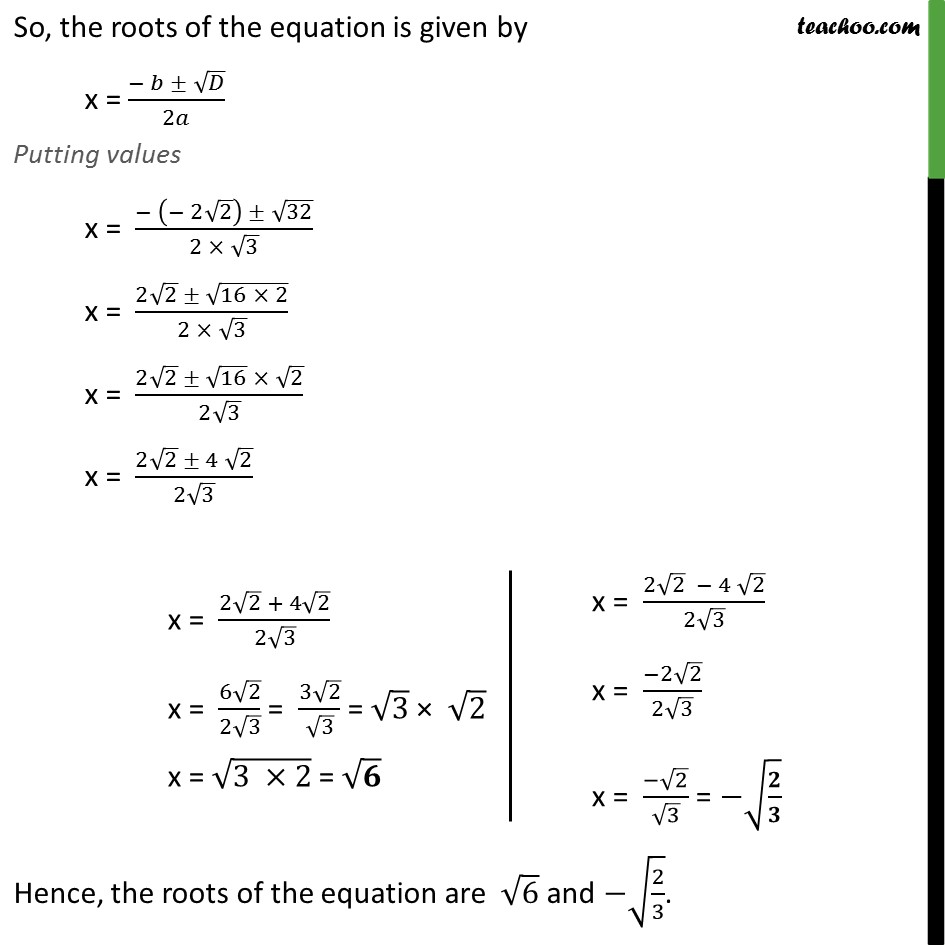
So, the roots of the equation is given by
x = (- b ± √D)/2a
Putting values
x = (- (- 2√2) ± √32)/(2 × √3)
x = (2√2 ± √(16 × 2))/(2 × √3)
x = (2√2 ± √16 × √2)/(2√3)
x = (2√2 ± 4 √2)/(2√3)
x = (2√2 + 4√2)/(2√3)
x = (6√2)/(2√3) = (3√2)/√3 = √3 × √2
x = √(3 ×2) = √6
x = (2√2 - 4 √2)/(2√3)
x = (-2√2)/(2√3)
x = (-√2)/√3 = -√(2/3)
Hence, the roots of the equation are √6 and -√(2/3).
Written on Jan. 22, 2017, 4:53 p.m.