Question 24 (Or 2nd) - CBSE Class 12 Sample Paper for 2019 Boards

Transcript

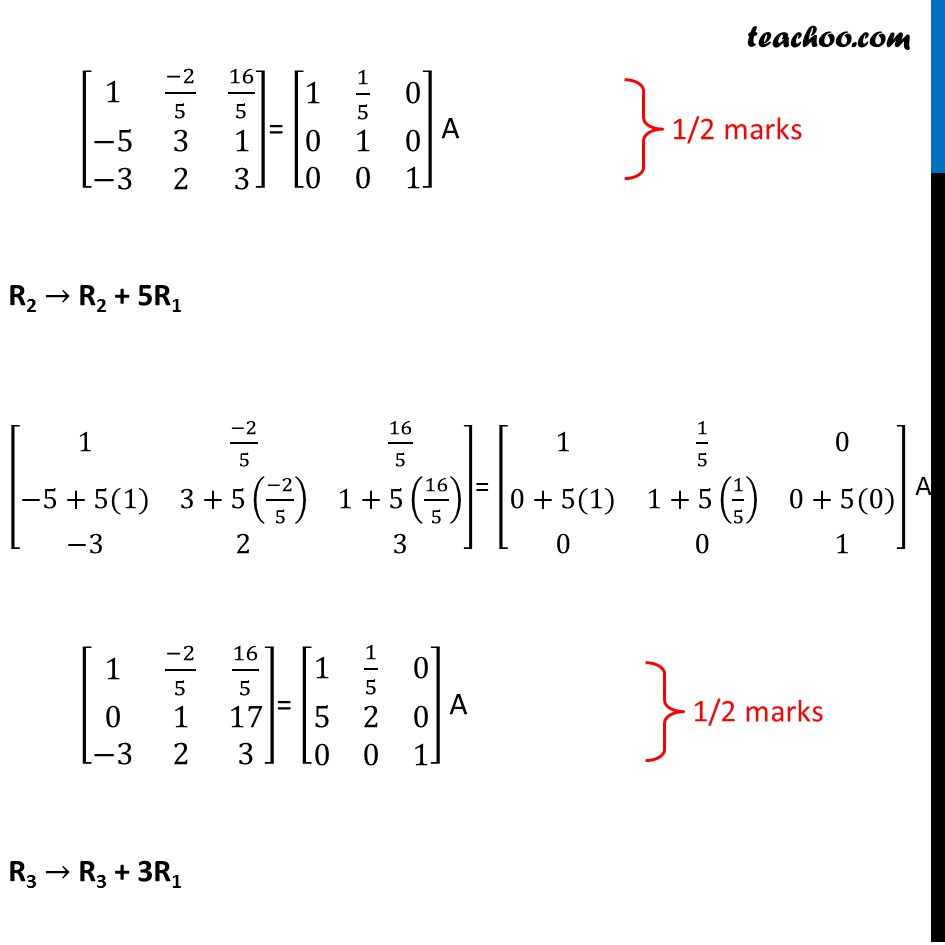
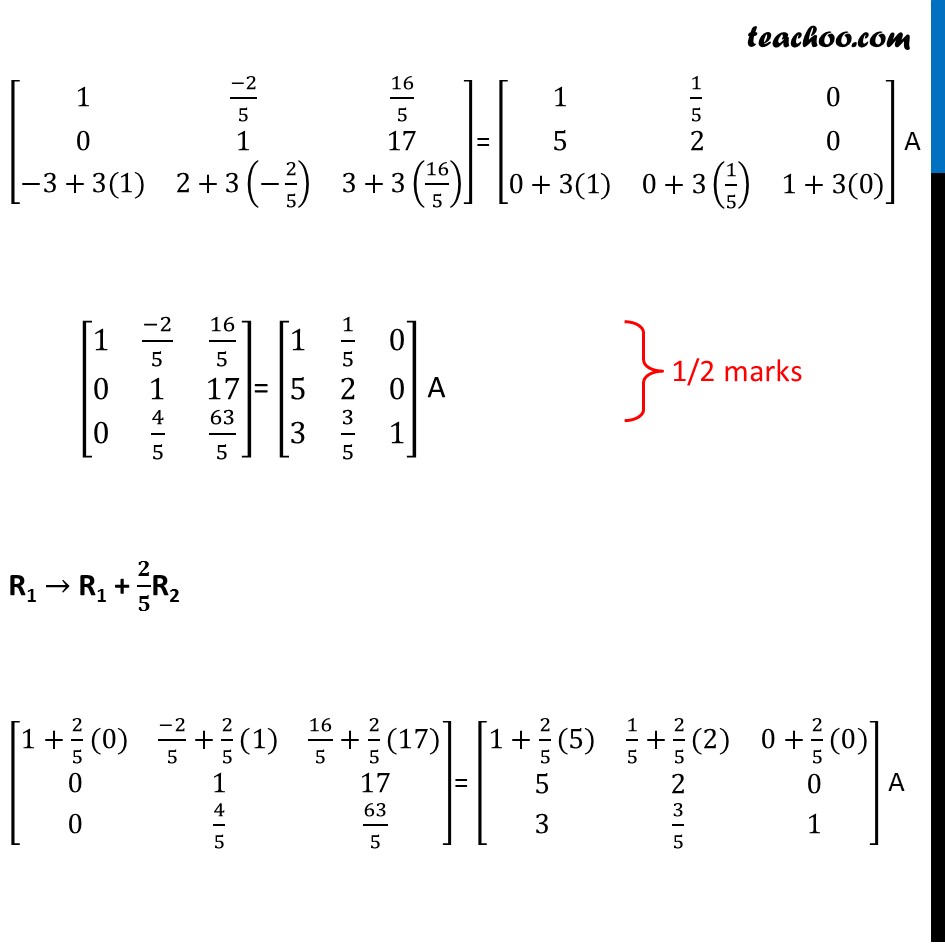
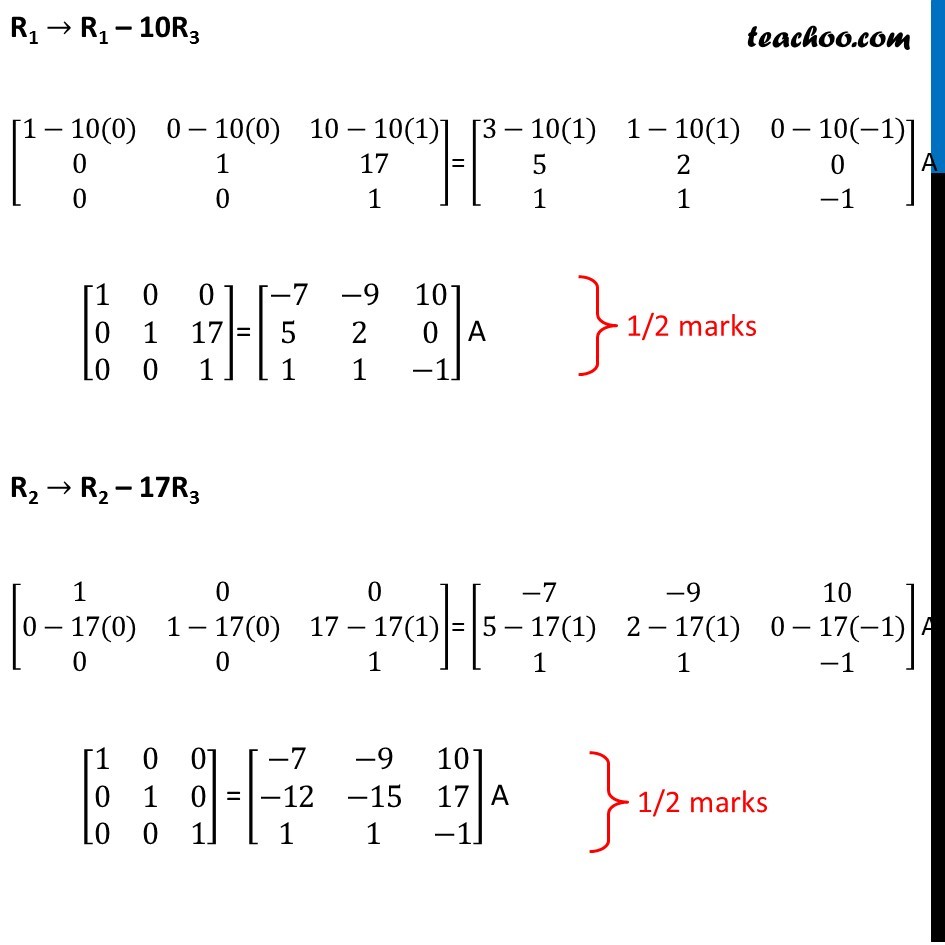
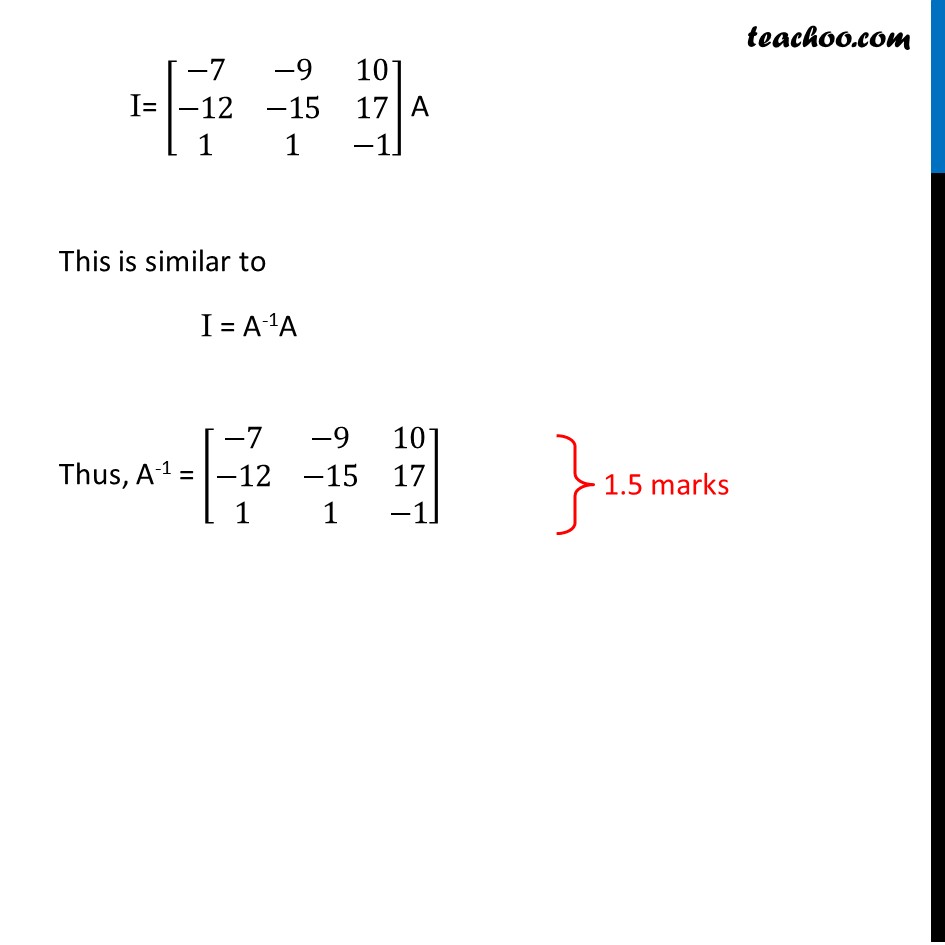
Question 24 (OR 2nd question) Find the inverse of the following matrix using elementary transformations [■8(2&−1&3@−5&3&1@−3&2&3)] Let A = [■8(2&−1&3@−5&3&1@−3&2&3)] We know that A = IA [■8(2&−1&3@−5&3&1@−3&2&3)]= [■8(1&0&0@0&1&0@0&0&1)] A R1 → R1 + 𝑹_𝟐/𝟓 [■8(1&(−2)/5&16/5@−5&3&1@−3&2&3)]= [■8(1&1/5&0@0&1&0@0&0&1)] A R2 → R2 + 5R1 [■8(1&(−2)/5&16/5@−5+5(1)&3+5((−2)/5)&1+5(16/5)@−3&2&3)]= [■8(1&1/5&0@0+5(1)&1+5(1/5)&0+5(0)@0&0&1)] A [■8(1&(−2)/5&16/5@0&1&17@−3&2&3)]= [■8(1&1/5&0@5&2&0@0&0&1)] A R3 → R3 + 3R1 [■8(1&(−2)/5&16/5@0&1&17@−3+3(1)&2+3(−2/5)&3+3(16/5) )]= [■8(1&1/5&0@5&2&0@0+3(1)&0+3(1/5)&1+3(0))] A [■8(1&(−2)/5&16/5@0&1&17@0&4/5&63/5)]= [■8(1&1/5&0@5&2&0@3&3/5&1)] A R1 → R1 + 𝟐/𝟓R2 [■8(1+2/5(0)&(−2)/5+2/5(1)&16/5+2/5(17)@0&1&17@0&4/5&63/5)]= [■8(1+2/5(5)&1/5+2/5(2)&0+2/5(0)@5&2&0@3&3/5&1)] A [■8(1&0&10@0&1&17@0&4/5&63/5)]= [■8(3&1&0@5&2&0@3&3/5&1)] A R3 → R3 – 𝟒/𝟓R2 [■8(1&0&10@0&1&17@0−4/5(0)&4/5−4/5(1)&63/5−4/5(17))]= [■8(3&1&0@5&2&0@3−4/5(5)&3/5−4/5(2)&1−4/5(0))] A [■8(1&0&10@0&1&17@0&0&−1)]= [■8(3&1&0@5&2&0@−1&−1&1)] A R3 → R3 × –1 [■8(1&0&10@0&1&17@0&0&1)]= [■8(3&1&0@5&2&0@1&1&−1)] A R1 → R1 – 10R3 [■8(1−10(0)&0−10(0)&10−10(1)@0&1&17@0&0&1)]= [■8(3−10(1)&1−10(1)&0−10(−1)@5&2&0@1&1&−1)] A [■8(1&0&0@0&1&17@0&0&1)]= [■8(−7&−9&10@5&2&0@1&1&−1)] A R2 → R2 – 17R3 [■8(1&0&0@0−17(0)&1−17(0)&17−17(1)@0&0&1)]= [■8(−7&−9&10@5−17(1)&2−17(1)&0−17(−1)@1&1&−1)] A [■8(1&0&0@0&1&0@0&0&1)] = [■8(−7&−9&10@−12&−15&17@1&1&−1)] A "I"= [■8(−7&−9&10@−12&−15&17@1&1&−1)] A This is similar to I = A-1A Thus, A-1 = [■8(−7&−9&10@−12&−15&17@1&1&−1)]