Question 14
Find the value of:
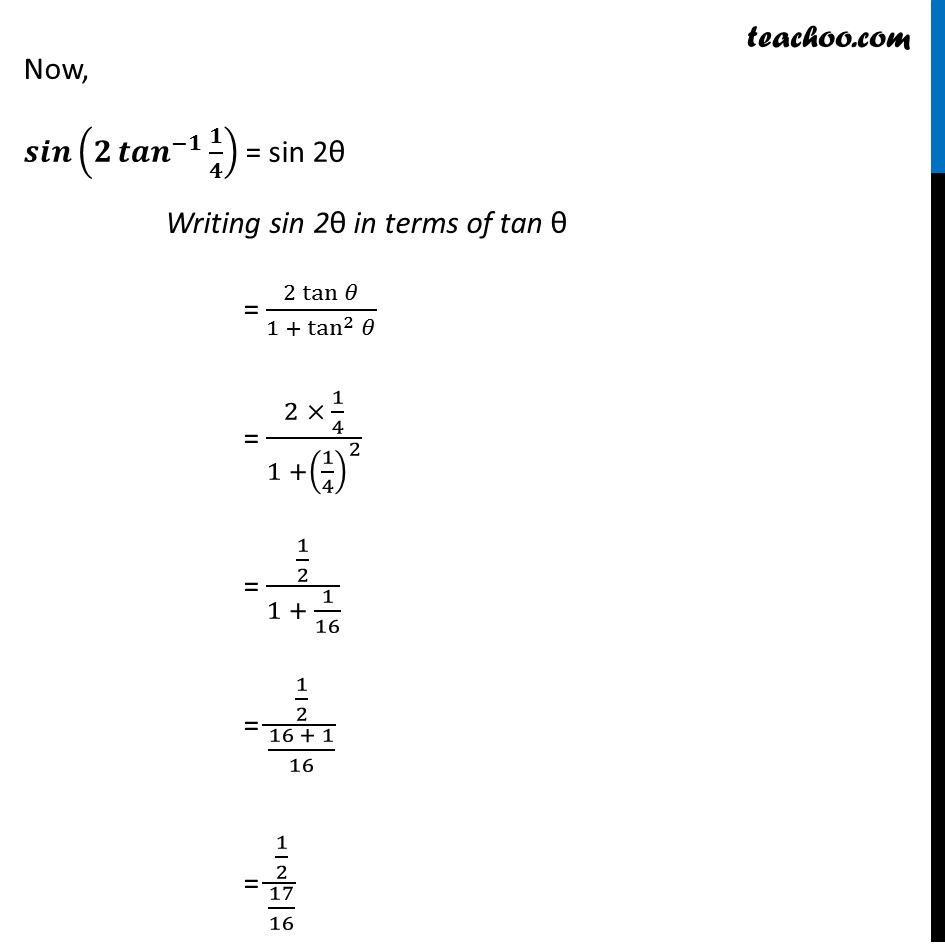
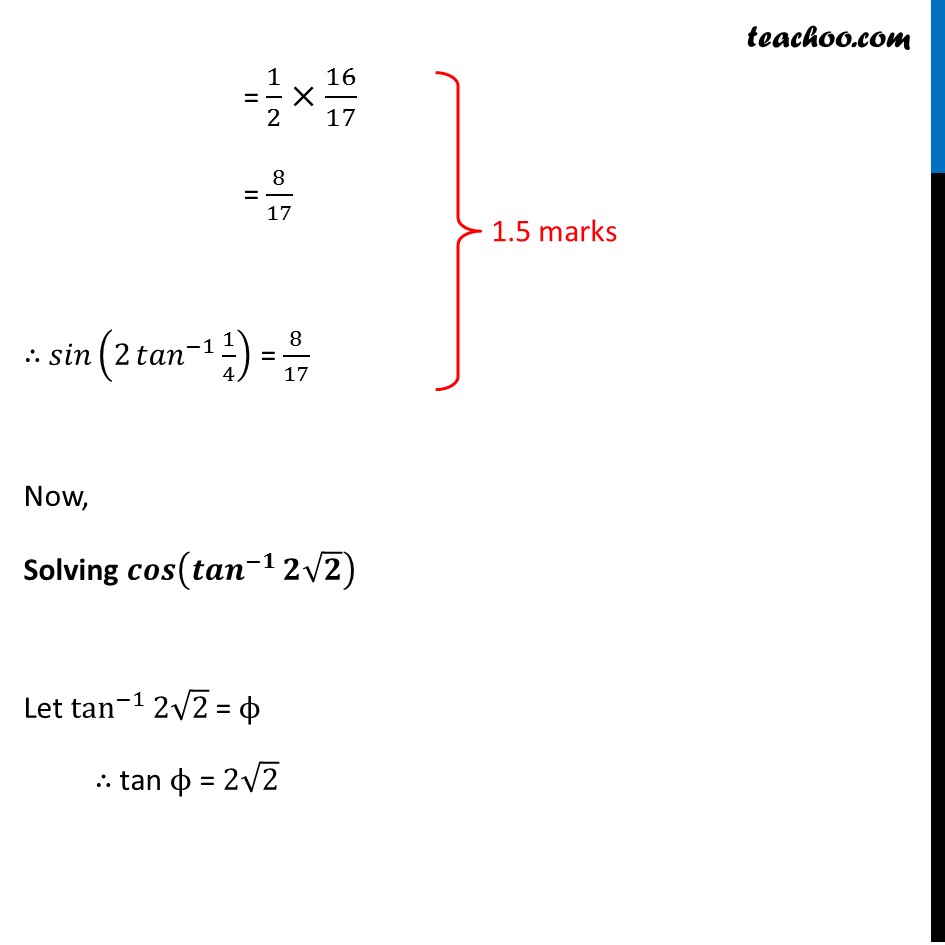
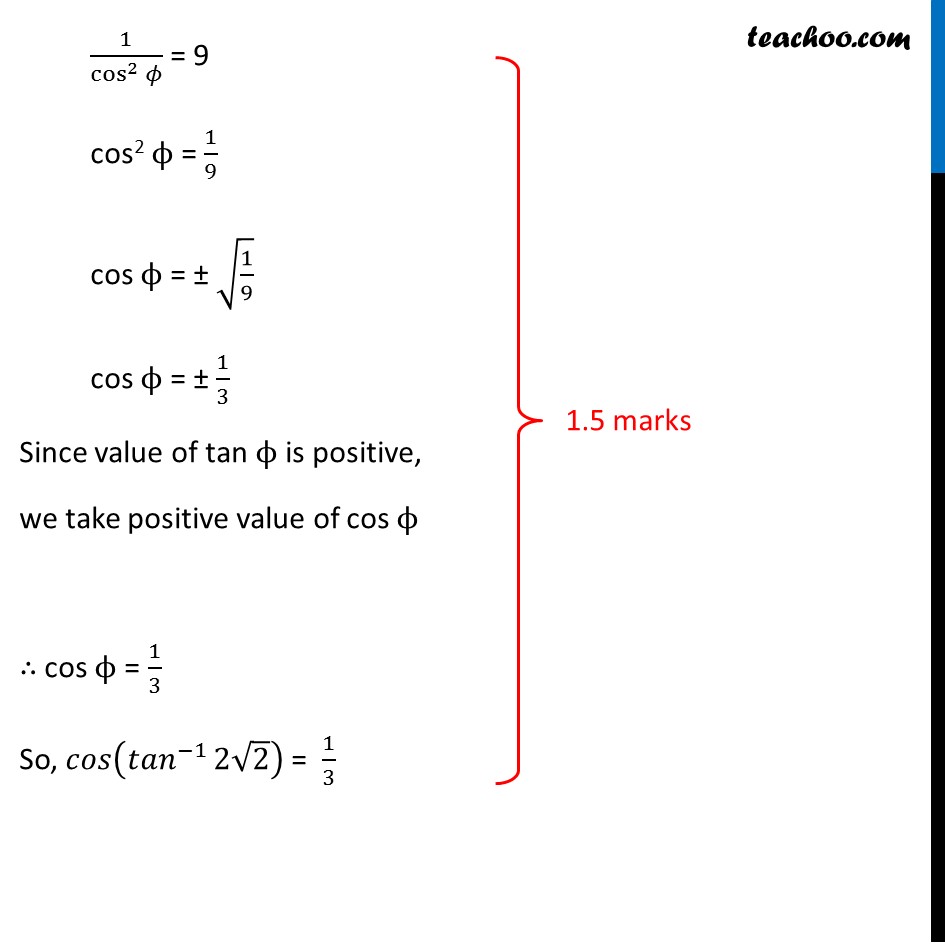
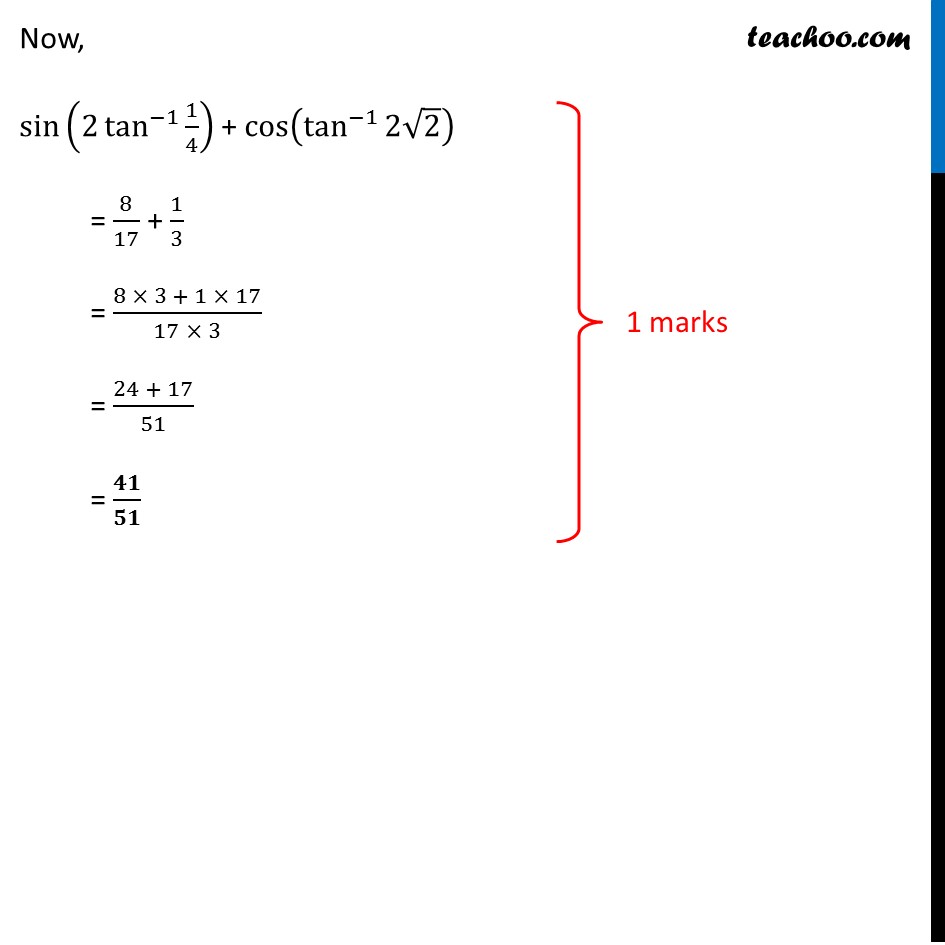
sin (2 tan -1 1/4) + cos (tan -1 2√2)


CBSE Class 12 Sample Paper for 2019 Boards
CBSE Class 12 Sample Paper for 2019 Boards
Last updated at Dec. 16, 2024 by Teachoo
Question 14
Find the value of:
sin (2 tan -1 1/4) + cos (tan -1 2√2)






Transcript
Question 14 Find the value of: sin(2 tan^(−1)〖1/4〗 ) + cos(tan^(−1)〖2√2〗 ) We solve sin(2 tan^(−1)〖1/4〗 ) & cos(tan^(−1)〖2√2〗 ) separately Solving 𝒔𝒊𝒏(𝟐 〖𝒕𝒂𝒏〗^(−𝟏)〖𝟏/𝟒〗 ) Let 〖𝑡𝑎𝑛〗^(−1)〖1/4〗=𝜃 1/4 = tan θ tan θ = 1/4 Now, 𝒔𝒊𝒏(𝟐 〖𝒕𝒂𝒏〗^(−𝟏)〖𝟏/𝟒〗 ) = sin 2θ Writing sin 2θ in terms of tan θ = (2 tan𝜃)/(1 + tan^2𝜃 ) = (2 × 1/4)/(1 +(1/4)^2 ) = (1/2)/(1 + 1/16) = (1/2)/((16 + 1)/16) = (1/2)/(17/16) = 1/2×16/17 = 8/17 ∴ 𝑠𝑖𝑛(2 〖𝑡𝑎𝑛〗^(−1)〖1/4〗 ) = 8/17 Now, Solving 𝒄𝒐𝒔(〖𝒕𝒂𝒏〗^(−𝟏)〖𝟐√𝟐〗 ) Let tan^(−1)〖2√2〗 = ϕ ∴ tan ϕ = 2√2 And, we need to find 𝑐𝑜𝑠(〖𝑡𝑎𝑛〗^(−1)〖2√2〗 ) = cos ϕ We know that 1 + tan2 ϕ = sec2 ϕ 1 + (2√2)^2 = sec2 ϕ 1 + 〖2^2 (√2)〗^2 = sec2 ϕ 1 + 4 × 2 = sec2 ϕ 1 + 8 = sec2 ϕ 9 = sec2 ϕ sec2 ϕ = 9 1/cos^2𝜙 = 9 And, we need to find 𝑐𝑜𝑠(〖𝑡𝑎𝑛〗^(−1)〖2√2〗 ) = cos ϕ We know that 1 + tan2 ϕ = sec2 ϕ 1 + (2√2)^2 = sec2 ϕ 1 + 〖2^2 (√2)〗^2 = sec2 ϕ 1 + 4 × 2 = sec2 ϕ 1 + 8 = sec2 ϕ 9 = sec2 ϕ sec2 ϕ = 9 1/cos^2𝜙 = 9 1/cos^2𝜙 = 9 cos2 ϕ = 1/9 cos ϕ = ± √(1/9) cos ϕ = ± 1/3 Since value of tan ϕ is positive, we take positive value of cos ϕ ∴ cos ϕ = 1/3 So, 𝑐𝑜𝑠(〖𝑡𝑎𝑛〗^(−1)〖2√2〗 ) = 1/3 Now, sin(2 tan^(−1)〖1/4〗 ) + cos(tan^(−1)〖2√2〗 ) = 8/17 + 1/3 = (8 × 3 + 1 × 17)/(17 × 3) = (24 + 17)/51 = 𝟒𝟏/𝟓𝟏