Question 10 (OR 1 st question)
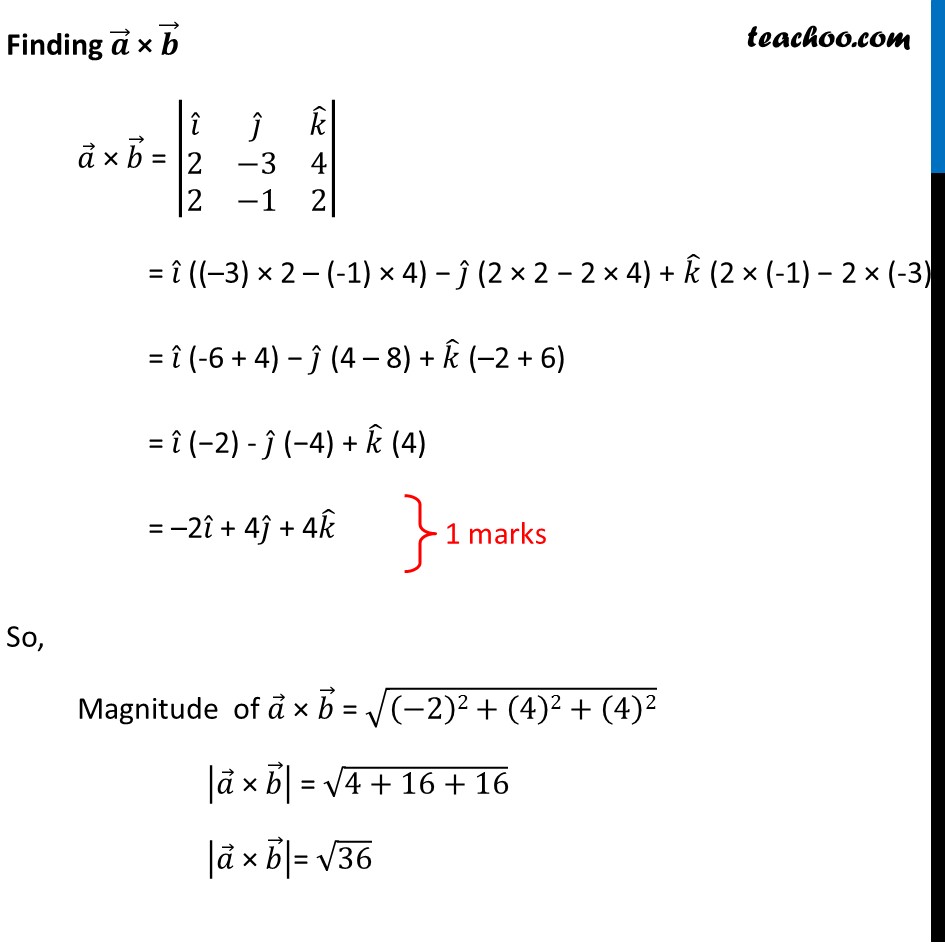
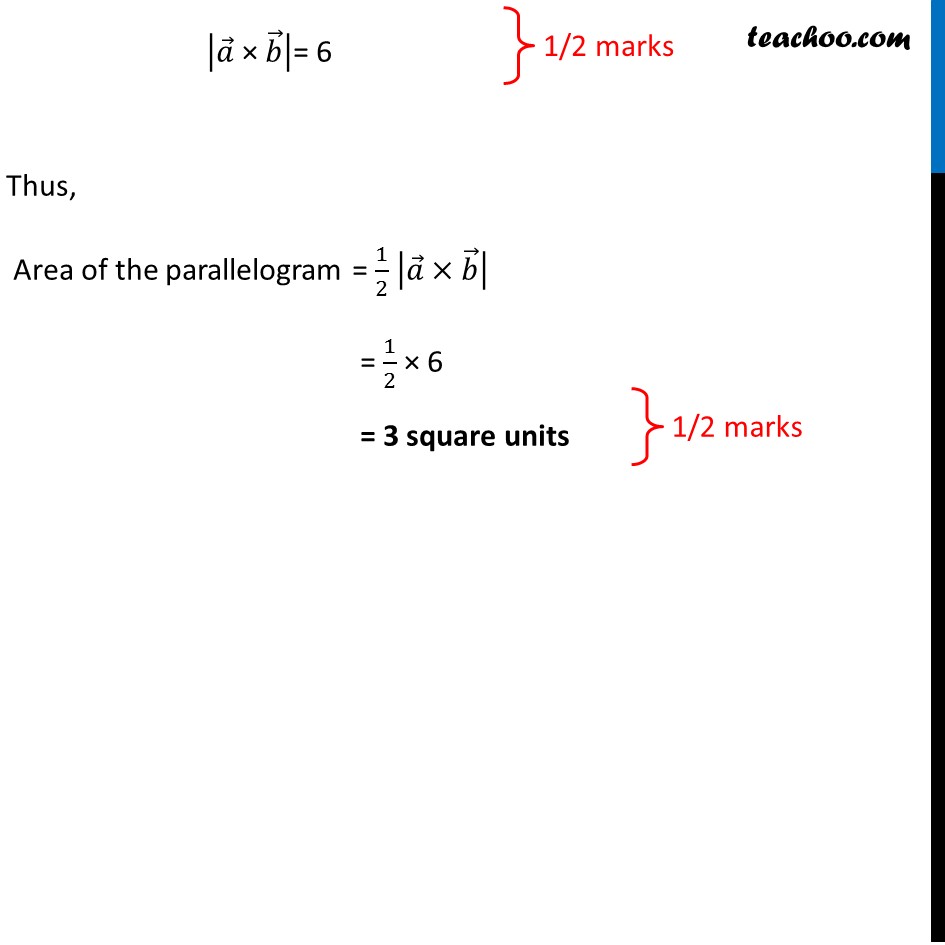
Find the area of the parallelogram whose diagonals are represented by the vectors a = 2i – 3j + 4k and b = 2i – j + 2k
We use the Area of Parallelogram formula with Diagonals

CBSE Class 12 Sample Paper for 2019 Boards
CBSE Class 12 Sample Paper for 2019 Boards
Last updated at Dec. 16, 2024 by Teachoo
Question 10 (OR 1 st question)
Find the area of the parallelogram whose diagonals are represented by the vectors a = 2i – 3j + 4k and b = 2i – j + 2k
We use the Area of Parallelogram formula with Diagonals



Transcript
Question 10 (OR 1st question) Find the area of the parallelogram whose diagonals are represented by the vectors 𝑎 ⃗ = 2𝑖 ̂ – 3𝑗 ̂ + 4𝑘 ̂ and 𝑏 ⃗ = 2𝑖 ̂ – 𝑗 ̂ + 2𝑘 ̂ Area of parallelogram with diagonals Area = 1/2 |(𝑑_1 ) ⃗×(𝑑_2 ) ⃗ | Given Diagonals of a parallelogram as 𝑎 ⃗ = 2𝑖 ̂ – 3𝑗 ̂ + 4𝑘 ̂ and 𝑏 ⃗ = 2𝑖 ̂ – 𝑗 ̂ + 2𝑘 ̂ Area of the parallelogram = 1/2 |𝑎 ⃗×𝑏 ⃗ | Finding 𝒂 ⃗ × 𝒃 ⃗ 𝑎 ⃗ × 𝑏 ⃗ = |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@2&−3&4@2&−1&2)| = 𝑖 ̂ ((–3) × 2 – (-1) × 4) − 𝑗 ̂ (2 × 2 − 2 × 4) + 𝑘 ̂ (2 × (-1) − 2 × (-3)) = 𝑖 ̂ (-6 + 4) − 𝑗 ̂ (4 – 8) + 𝑘 ̂ (–2 + 6) = 𝑖 ̂ (−2) - 𝑗 ̂ (−4) + 𝑘 ̂ (4) = –2𝑖 ̂ + 4𝑗 ̂ + 4𝑘 ̂ So, Magnitude of 𝑎 ⃗ × 𝑏 ⃗ = √((−2)2+(4)2+(4)2) |𝑎 ⃗" × " 𝑏 ⃗ | = √(4+16+16) |𝑎 ⃗" × " 𝑏 ⃗ |= √36 |𝑎 ⃗" × " 𝑏 ⃗ |= 6 Thus, Area of the parallelogram = 1/2 |𝑎 ⃗×𝑏 ⃗ | = 1/2 × 6 = 3 square units