Question 8 (OR 1 st question)
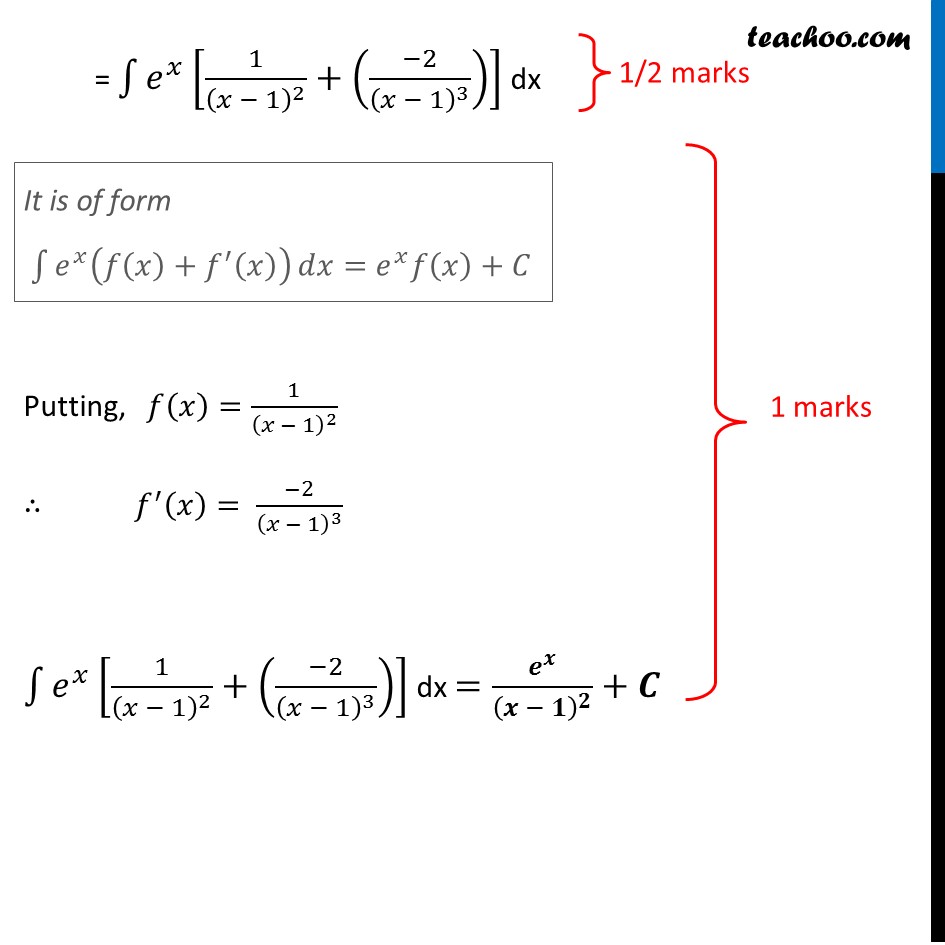
Find: ∫ e x (x - 3) / (x - 1) 3 dx

CBSE Class 12 Sample Paper for 2019 Boards
CBSE Class 12 Sample Paper for 2019 Boards
Last updated at Dec. 16, 2024 by Teachoo
Question 8 (OR 1 st question)
Find: ∫ e x (x - 3) / (x - 1) 3 dx


Transcript
Question 8 (OR 1st question) Find: ∫1▒(𝑒^𝑥 (𝑥 − 3))/(𝑥 − 1)^3 dx ∫1▒(𝑒^𝑥 (𝑥 − 3))/(𝑥 − 1)^3 dx = ∫1▒(𝑒^𝑥 (𝑥 − 1 − 2))/(𝑥 − 1)^3 dx = ∫1▒(𝑒^𝑥 [(𝑥 − 1)− 2])/(𝑥 − 1)^3 dx = ∫1▒〖𝑒^𝑥 [((𝑥 − 1) − 2)/(𝑥 − 1)^3 ] 〗 dx = ∫1▒〖𝑒^𝑥 [((𝑥 − 1))/(𝑥 − 1)^3 +((−2)/(𝑥 − 1)^3 )] 〗 dx = ∫1▒〖𝑒^𝑥 [1/(𝑥 − 1)^2 +((−2)/(𝑥 − 1)^3 )] 〗 dx It is of form ∫1▒〖𝑒^𝑥 (𝑓(𝑥)+𝑓^′ (𝑥)) 〗 𝑑𝑥=𝑒^𝑥 𝑓(𝑥)+𝐶 Putting, 𝑓(𝑥)=1/(𝑥 − 1)^2 " " ∴ 𝑓^′ (𝑥)= (−2)/(𝑥 − 1)^3 ∫1▒〖𝑒^𝑥 [1/(𝑥 − 1)^2 +((−2)/(𝑥 − 1)^3 )] 〗 dx =𝒆^𝒙/(𝒙 − 𝟏)^𝟐 +𝑪