Question 4 - Forming Linear Equations - Two digit number - Chapter 2 Class 8 Linear Equations in One Variable

Transcript

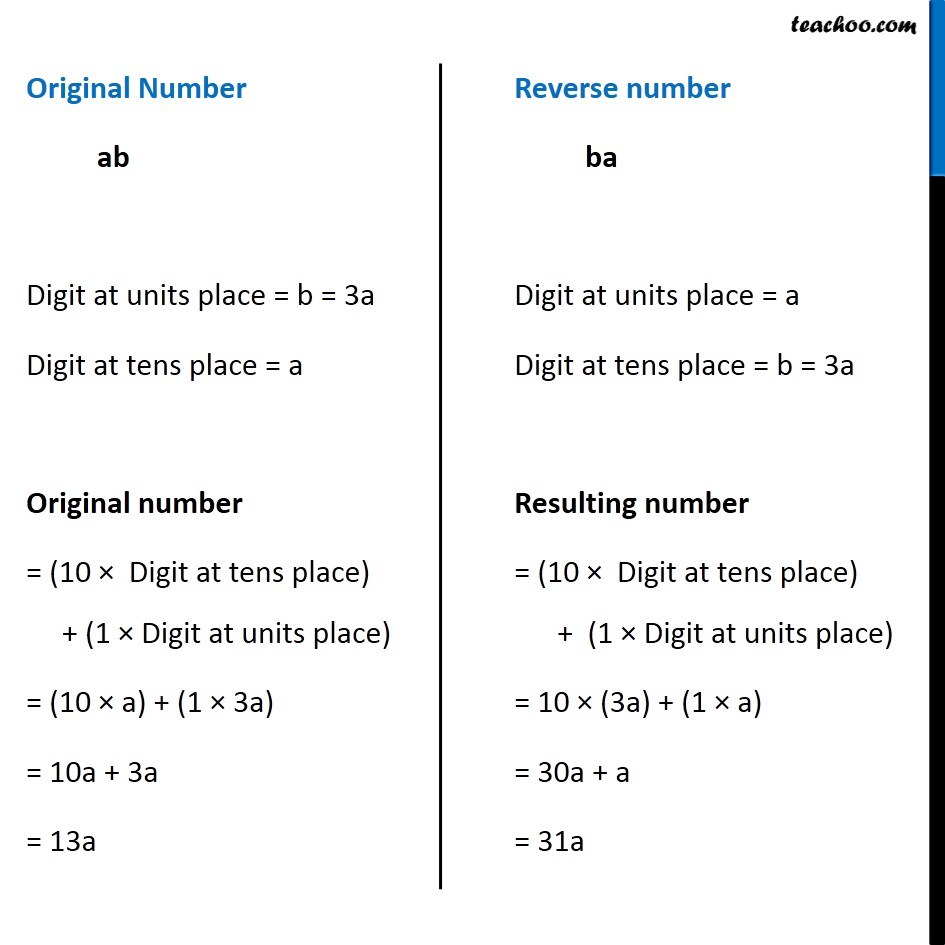
Question 4 One of the two digits of a two digit number is three times the other digit. If you interchange the digits of this two-digit number and add the resulting number to the original number, you get 88. What is the original number?Let original number be ab Let the digit at tens place = a & digit at units place = b Given that One of the two digits of a two digit number is three times the other digit. b = 3a Original Number ab Digit at units place = b = 3a Digit at tens place = a Original number = (10 × Digit at tens place) + (1 × Digit at units place) = (10 × a) + (1 × 3a) = 10a + 3a = 13a Reverse number ba Digit at units place = a Digit at tens place = b = 3a Resulting number = (10 × Digit at tens place) + (1 × Digit at units place) = 10 × (3a) + (1 × a) = 30a + a = 31a Hence, Original number = 13a Resulting number = 31a Given that, If we add resulting number to original number, we get 88. Resulting number + Original number = 88 13a + 31a = 88 44a = 88 a = 88/44 a = 2 Therefore, Digit at tens place = a = 2 & Digit at units place = 3a = 3 × 2 = 6 Hence, the original number = 26 Note: While forming the equation, We are told One of the two digits of a two digit number is three times the other digit. b = 3a We could have also taken a = 3b If we would have taken that, answer will be 62 Both the answers are correct.