For general solutions
We must learn
For sin x = sin y,
x = nπ + (–1) n y, where n ∈ Z
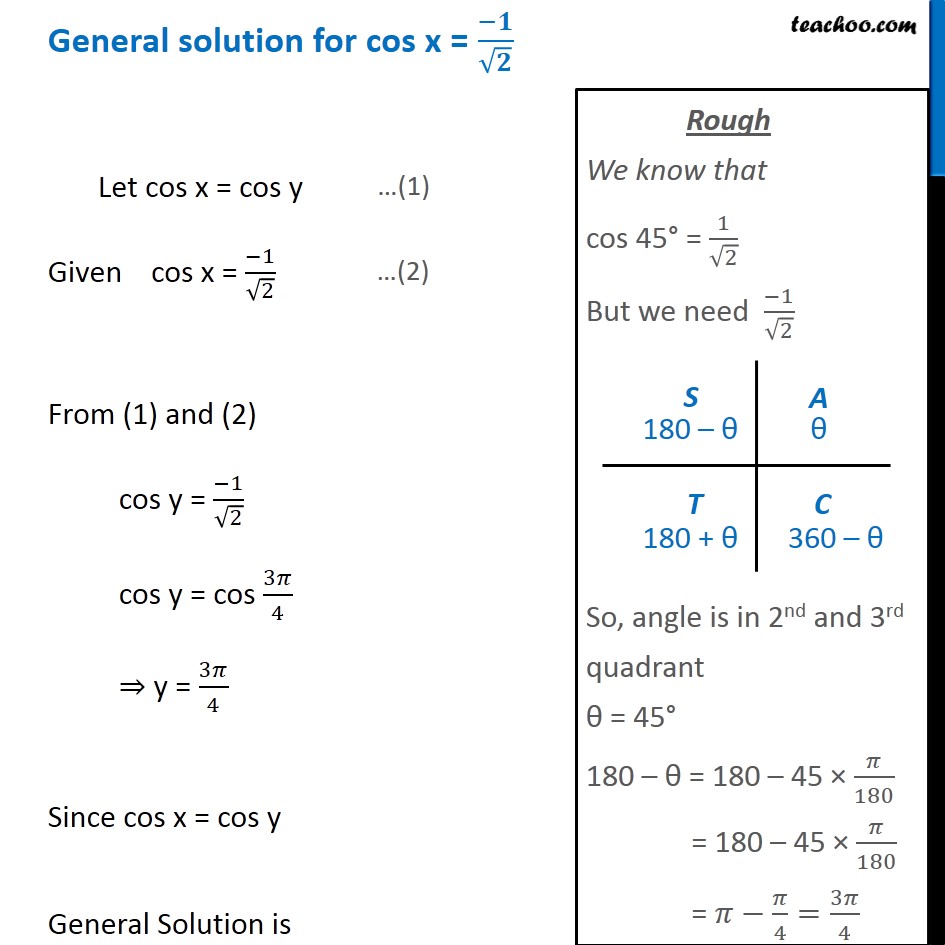
For cos x = cos y ,
x = 2nπ ± y, where n ∈ Z
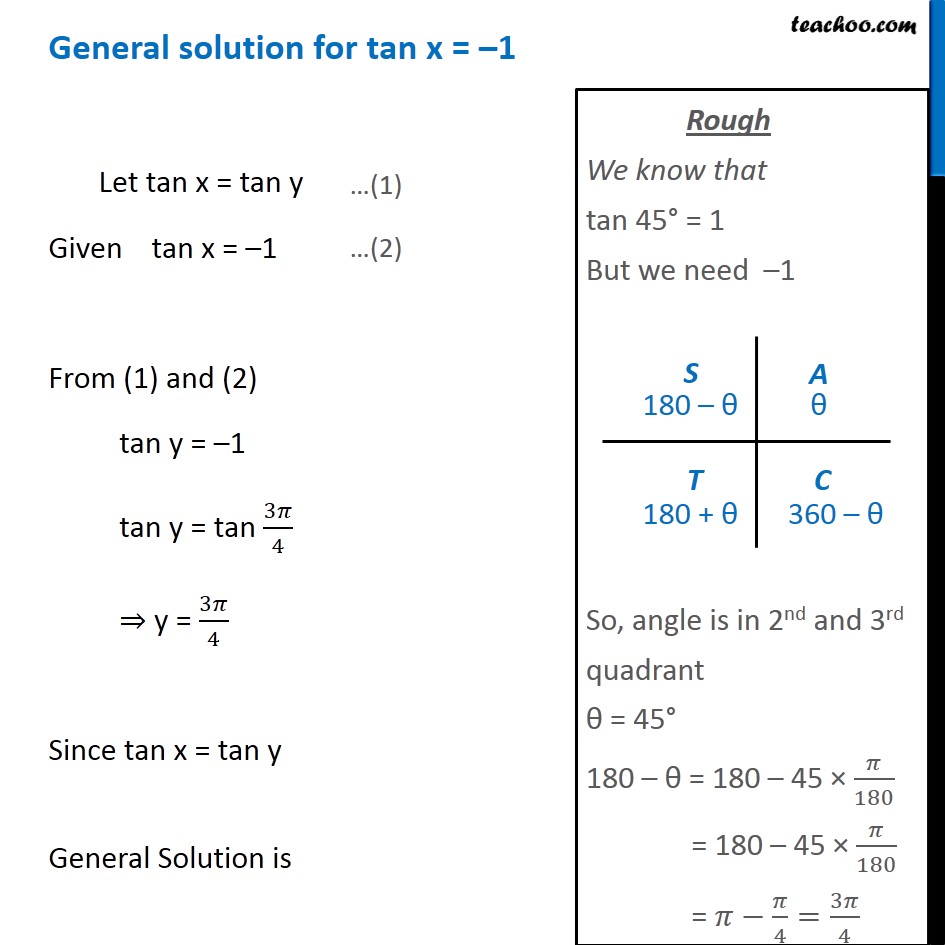
For tan x = tan y,
x = nπ + y, where n ∈ Z
Note : Here n ∈ Z means n is an integer




Finding General Solutions
Last updated at Dec. 16, 2024 by Teachoo
For general solutions
We must learn
For sin x = sin y,
x = nπ + (–1) n y, where n ∈ Z
For cos x = cos y ,
x = 2nπ ± y, where n ∈ Z
For tan x = tan y,
x = nπ + y, where n ∈ Z
Note : Here n ∈ Z means n is an integer






Transcript
For general solutions We must learn For sin x = sin y, x = nπ + (–1)n y, where n ∈ Z For cos x = cos y, x = 2nπ ± y, where n ∈ Z For tan x = tan y, x = nπ + y, where n ∈ Z Note: Here n ∈ Z means n is an integer