Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Dec. 16, 2024 by

If y = log (√x + 1 / √x) 2  then prove that x (x + 1) 2 y 2 + (x + 1) 2 y 1 = 2

This is a question of CBSE Sample Paper - Class 12 - 2017/18.

You can download the question paper here  https://www.teachoo.com/cbse/sample-papers/

If y = log (root x + 1/root)^2, then prove x (x+1)^2 y2 + (x+1)^2 y1 =

Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Part 2
Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Part 3
Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Part 4
Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Part 5 Question 15 - CBSE Class 12 Sample Paper for 2018 Boards - Part 6

Go Ad-free

Transcript

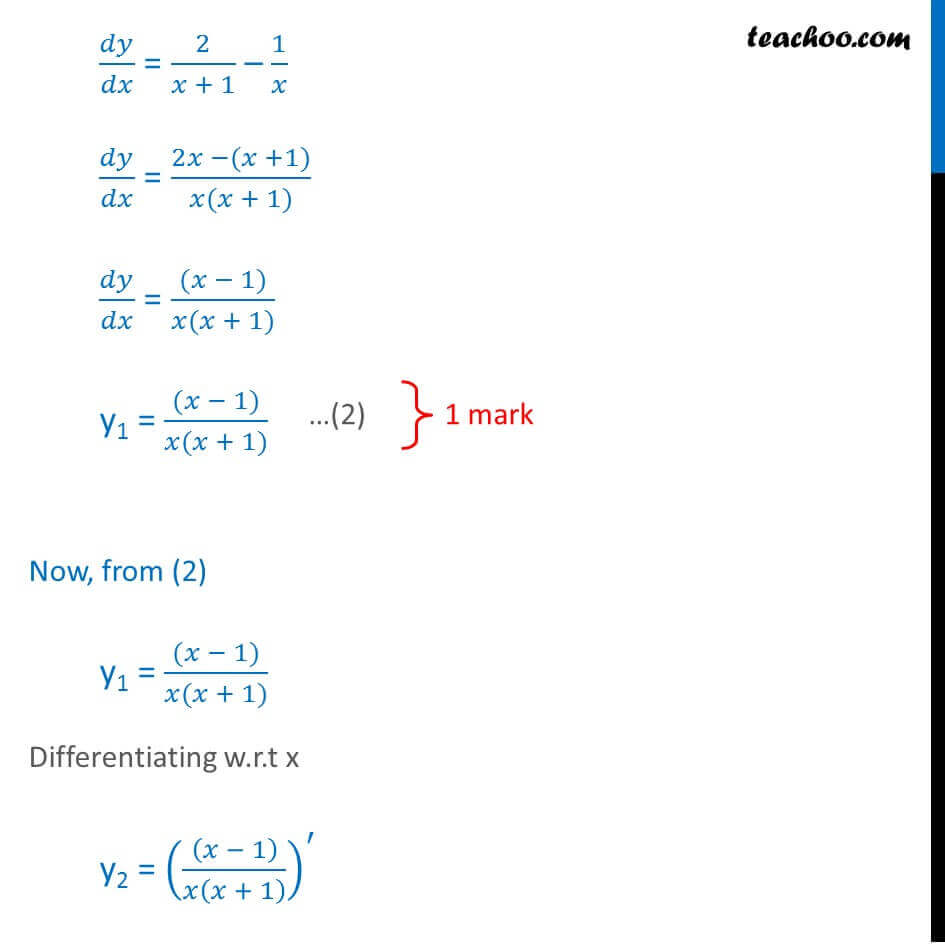
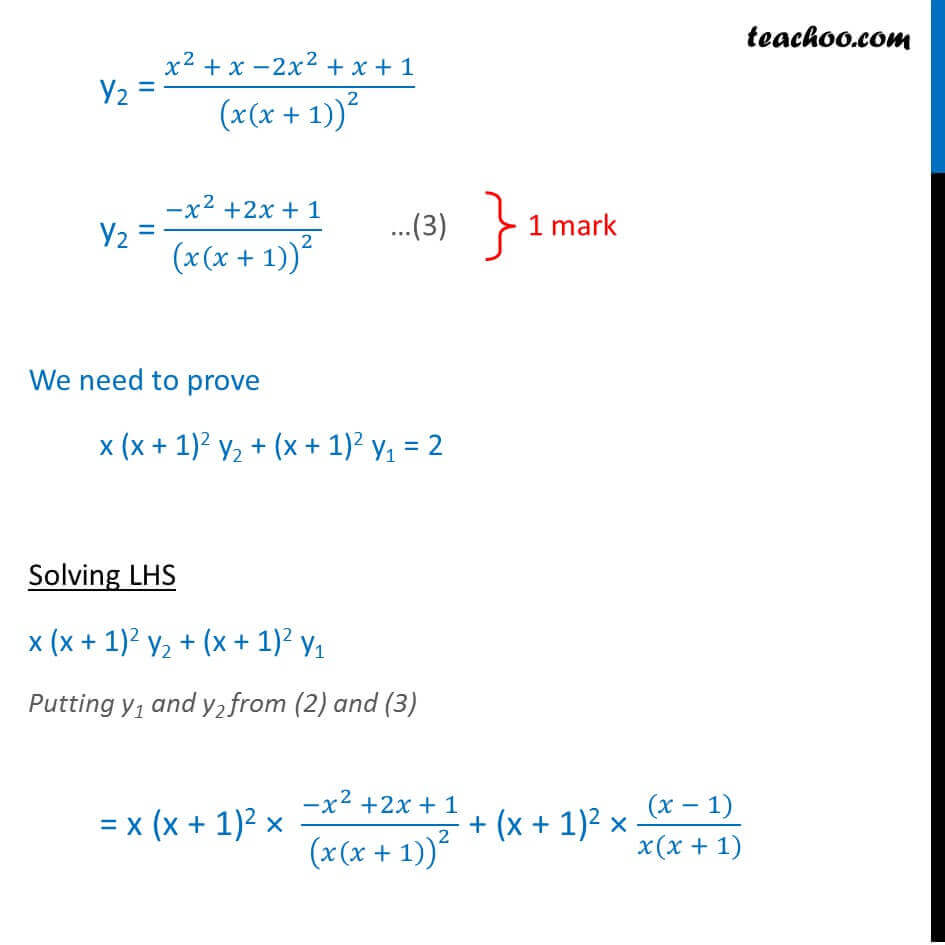
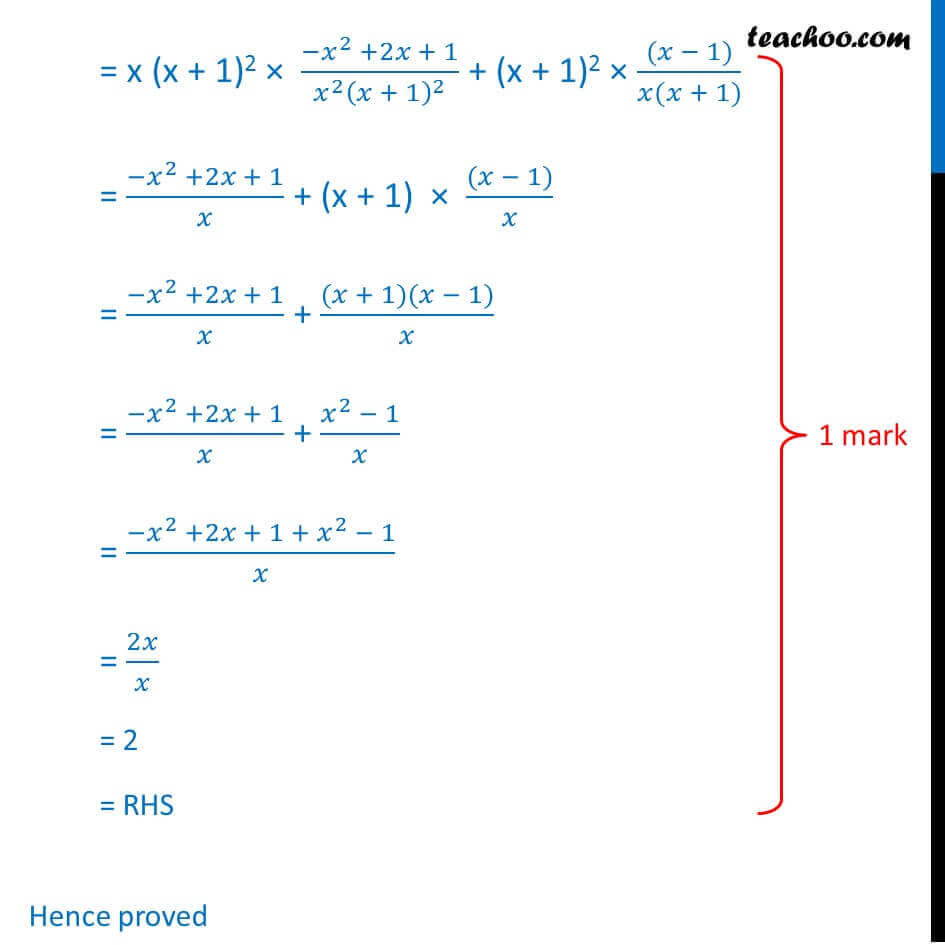
Question 15 If y = log ( +1/ )^2then prove that x (x + 1)2 y2 + (x + 1)2 y1 = 2 Given y = log ( +1/ )^2 Using log a2 = 2 log a y = 2 log ( +1/ ) y = 2 log (( + 1)/ ) y = 2 log (( + 1)/ ) Using log / = log a log b y = 2 log ( +1) 2 log y = 2 log ( +1) 2 log ( )^(1/2) Using log a2 = 2 log a y = 2 log ( +1) 2 1/2 log y = 2 log ( +1) log Now finding y1 and y2 From (1) y = 2 log ( +1) log Differentiating w.r.t x / = 2 (log ( + 1) )/ (log )/ / = 2 1/( + 1) 1/ / = 2/( + 1) 1/ / = (2 ( +1))/( ( + 1)) / = (( 1))/( ( + 1)) y1 = (( 1))/( ( + 1)) Now, from (2) y1 = (( 1))/( ( + 1)) Differentiating w.r.t x y2 = ((( 1))/ ( + 1) )^ = x (x + 1)2 ( ^2 +2 + 1)/( ^2 ( + 1)^2 ) + (x + 1)2 (( 1))/( ( + 1)) = ( ^2 +2 + 1)/ + (x + 1) (( 1))/ = ( ^2 +2 + 1)/ + (( + 1)( 1))/ = ( ^2 +2 + 1)/ + ( ^2 1)/ = ( ^2 +2 + 1 + ^2 1)/ = 2 / = 2 = RHS Hence proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo