CBSE Class 10 Sample Paper for 2018 Boards
You are here
CBSE Class 10 Sample Paper for 2018 Boards
Last updated at Dec. 16, 2024 by Teachoo
This is a question of CBSE Sample Paper - Class 10 - 2017/18.
You can download the question paper here https://www.teachoo.com/cbse/sample-papers/
Transcript
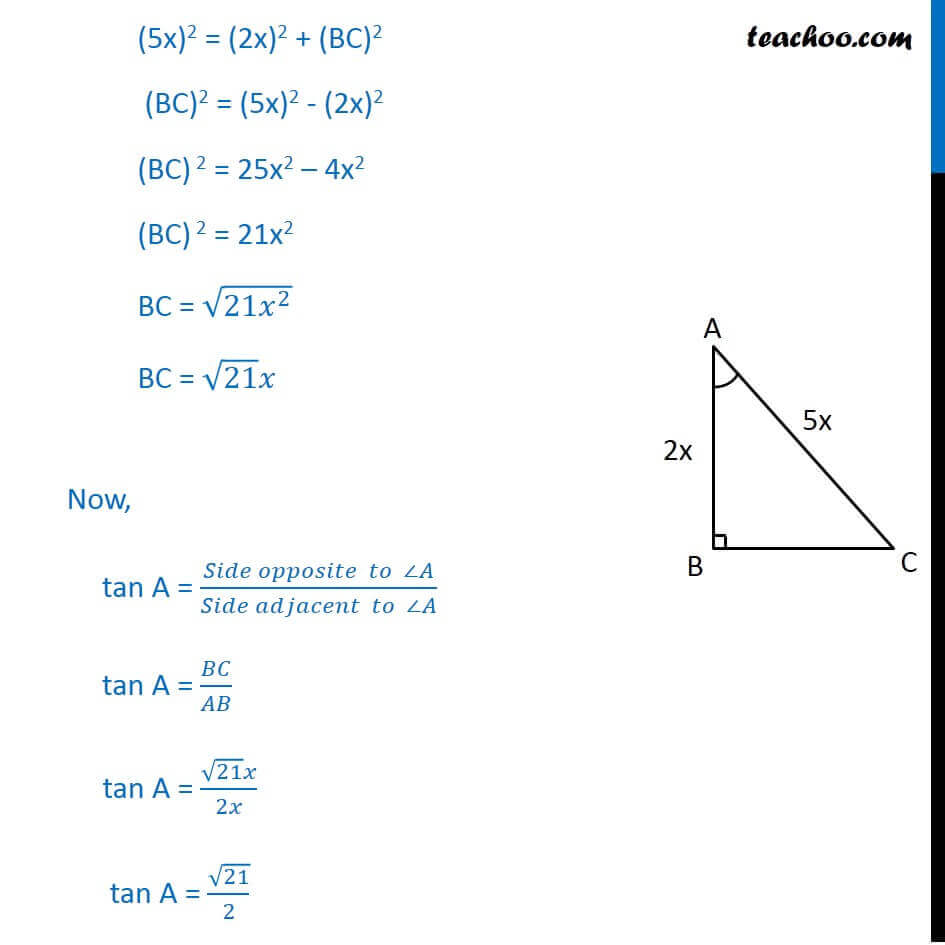
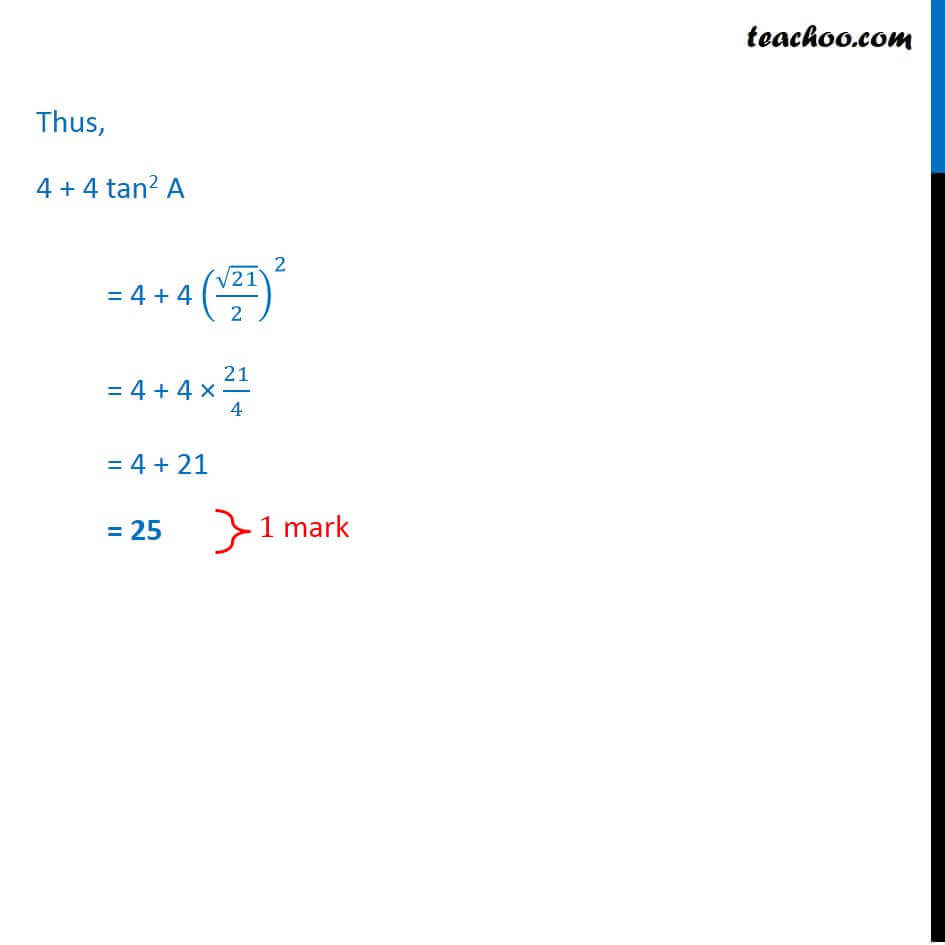
Question 6 If cos A = 2/5 , find the value of 4 + 4 tan2 A Given cos A = 2/5 (𝑆𝑖𝑑𝑒 𝑎𝑑𝑗𝑎𝑐𝑒𝑛𝑡 𝑡𝑜 ∠𝐴)/𝐻𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒 = 2/5 𝐴𝐵/𝐴𝐶=2/5 Let AB = 2x & AC = 5x Using Pythagoras theorem to find BC (Hypotenuse)2 = (Height)2 + (Base)2 AC2 = AB2 + BC2 (5x)2 = (2x)2 + (BC)2 (BC)2 = (5x)2 - (2x)2 (BC) 2 = 25x2 – 4x2 (BC) 2 = 21x2 BC = √(21𝑥^2 ) BC = √21 𝑥 Now, tan A = (𝑆𝑖𝑑𝑒 𝑜𝑝𝑝𝑜𝑠𝑖𝑡𝑒 𝑡𝑜 ∠𝐴)/(𝑆𝑖𝑑𝑒 𝑎𝑑𝑗𝑎𝑐𝑒𝑛𝑡 𝑡𝑜 ∠𝐴) tan A = 𝐵𝐶/𝐴𝐵 tan A = (√21 𝑥)/2𝑥 tan A = √21/2 Thus, 4 + 4 tan2 A = 4 + 4 (√21/2)^2 = 4 + 4 × 21/4 = 4 + 21 = 25
