Question 3 (Supplementary NCERT) - Examples - Chapter 7 Class 12 Integrals

Transcript

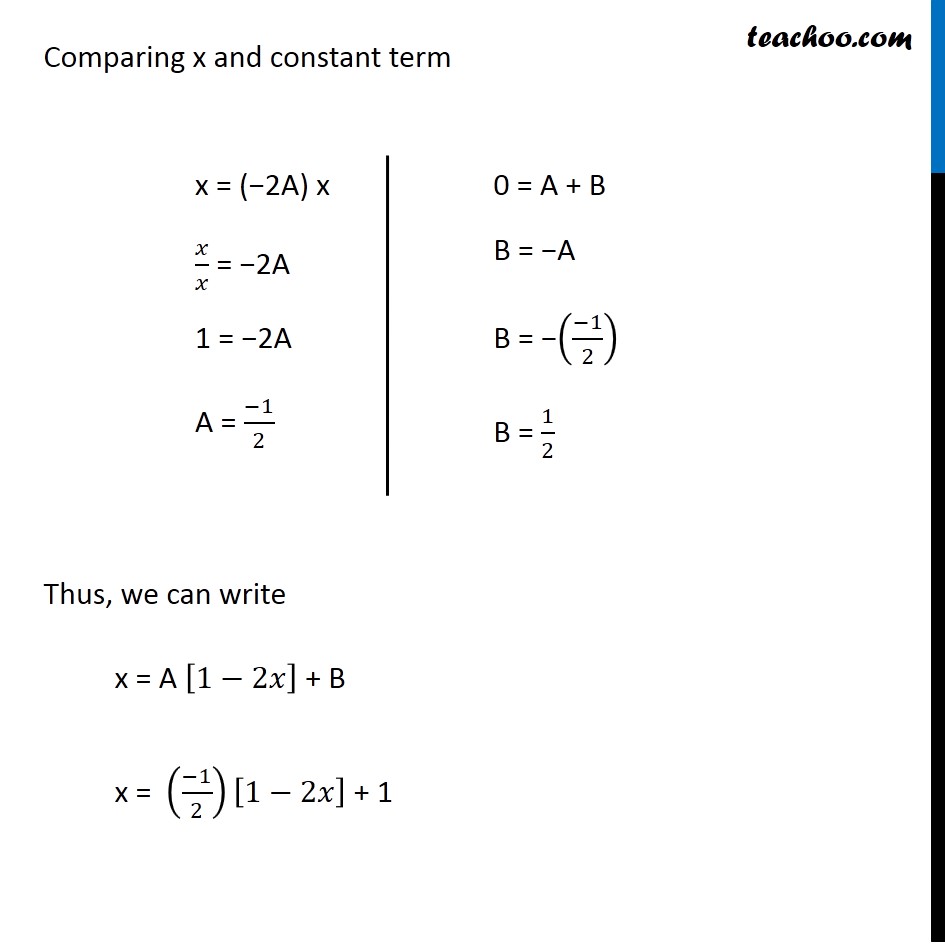
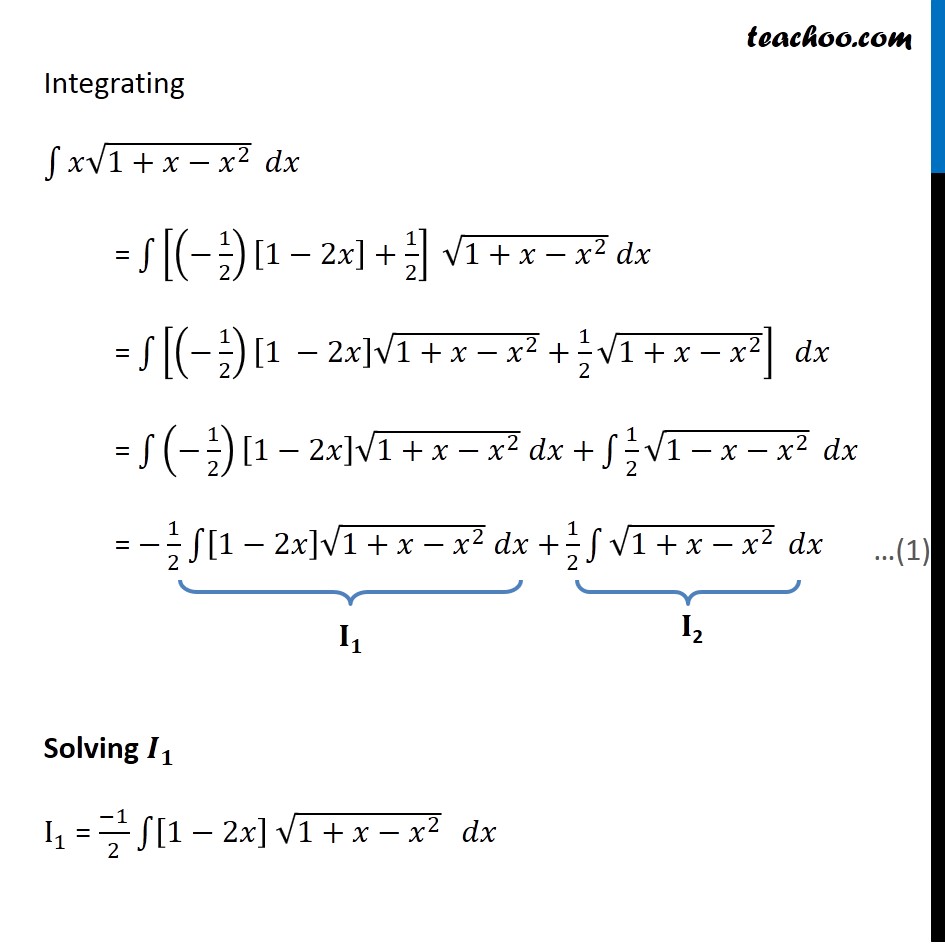
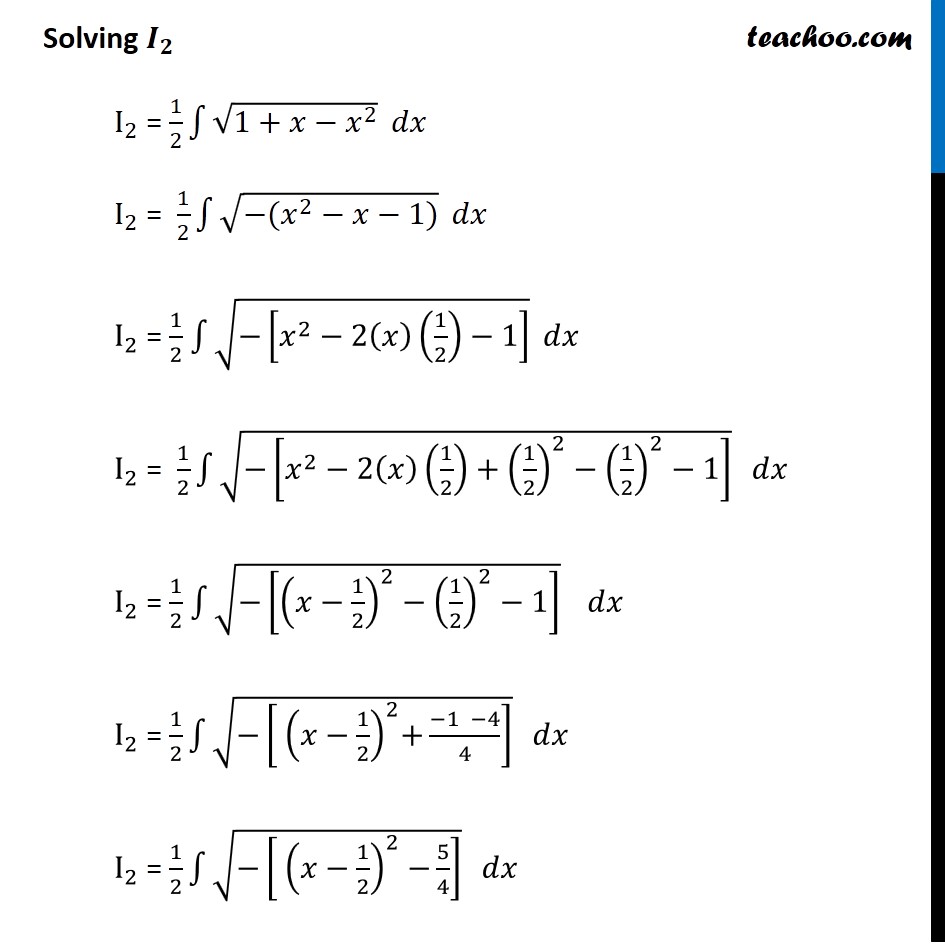
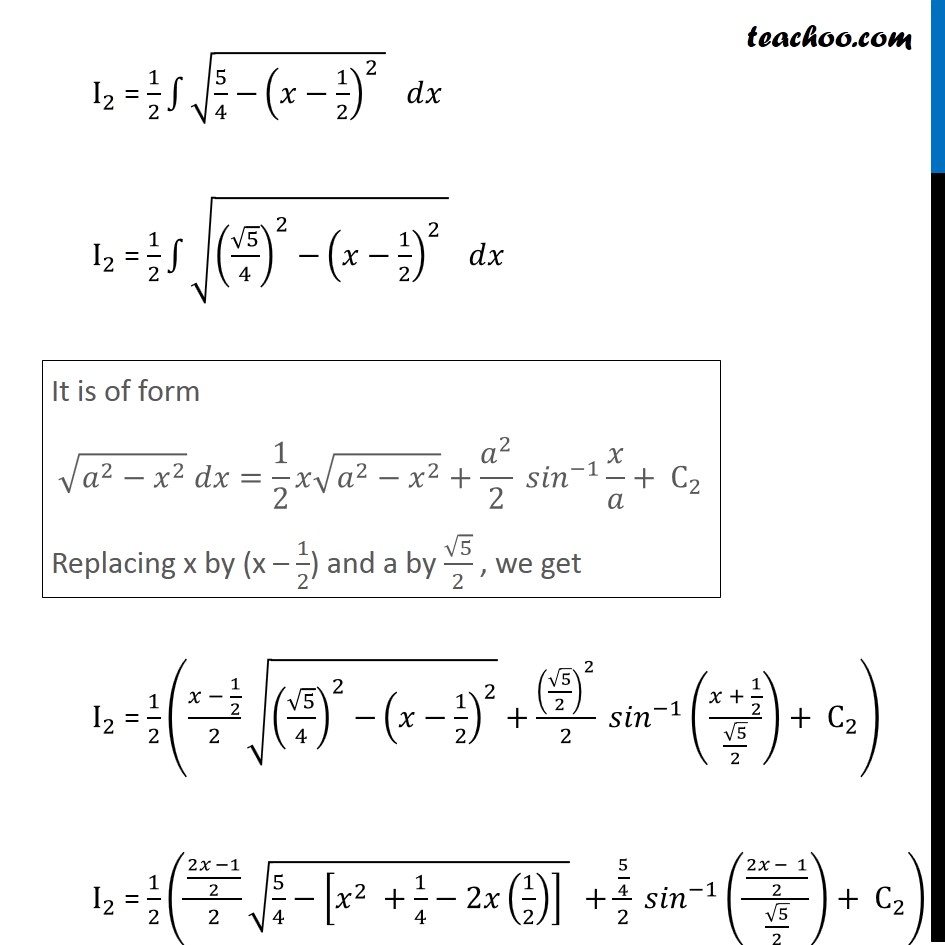
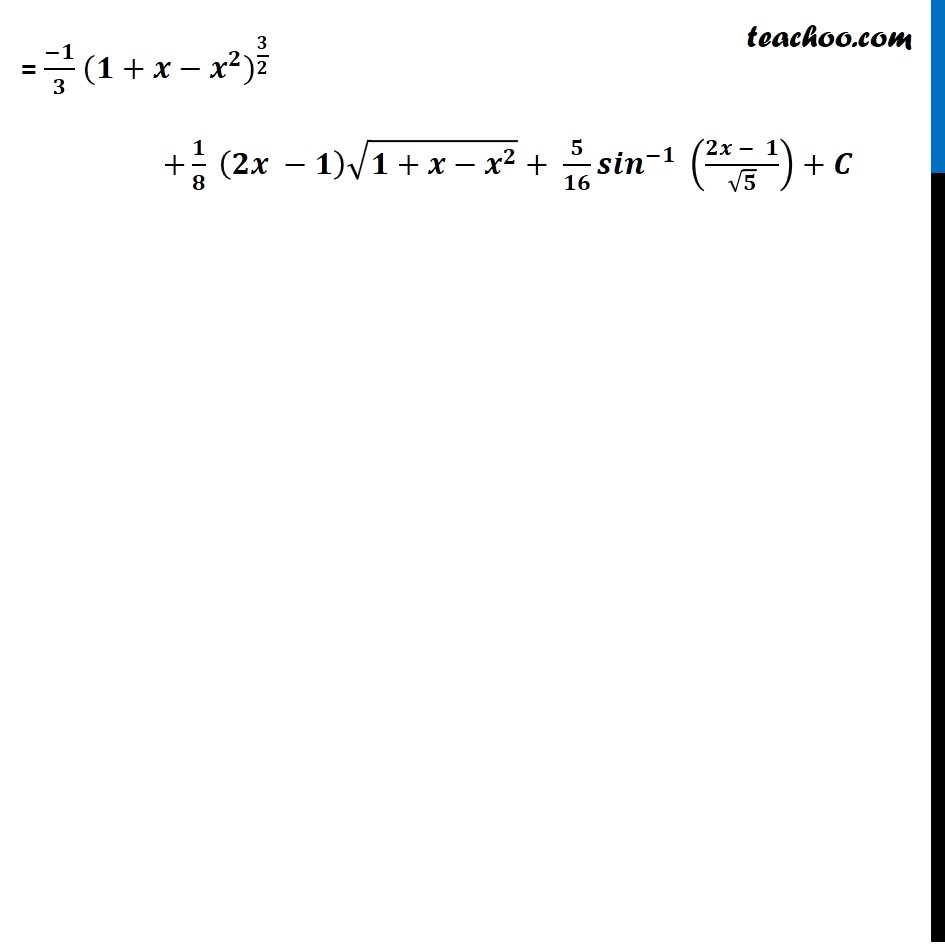
Question 3 (Supplementary NCERT) ∫1▒𝑥 √(1+𝑥−𝑥^2 ) 𝑑𝑥 ∫1▒𝑥 √(1+𝑥−𝑥^2 ) 𝑑𝑥 We can write it as:- x = A [𝑑/𝑑𝑥 (1+𝑥−𝑥^2 )]+ B x = A [0+1−2𝑥]+ B x = A [1−2𝑥]+ B x = "A"−2"A" 𝑥+ B x = −2"A" 𝑥+(𝐴+𝐵) Comparing x and constant term Thus, we can write x = A [1−2𝑥] + B x = ((−1)/2)[1−2𝑥] + 1 x = (−2A) x 𝑥/𝑥 = −2A 1 = −2A A = (−1)/2 0 = A + B B = −A B = −((−1)/2) B = 1/2 Integrating ∫1▒〖𝑥√(1+𝑥−𝑥^2 ) 〗 𝑑𝑥 = ∫1▒〖[(−1/2)[1−2𝑥]+1/2] 〗 √(1+𝑥−𝑥^2 ) 𝑑𝑥 = ∫1▒〖[(−1/2)[1 −2𝑥] √(1+𝑥−𝑥^2 )+1/2 √(1+𝑥−𝑥^2 )] 〗 𝑑𝑥 = ∫1▒〖(−1/2)[1−2𝑥] √(1+𝑥−𝑥^2 ) 𝑑𝑥+〗 ∫1▒〖1/2 √(1−𝑥−𝑥^2 )〗 𝑑𝑥 = −1/2 ∫1▒〖[1−2𝑥] √(1+𝑥−𝑥^2 ) 𝑑𝑥+〗 1/2 ∫1▒√(1+𝑥−𝑥^2 ) 𝑑𝑥 Solving 𝑰_𝟏 I_1 = (−1)/2 ∫1▒〖[1−2𝑥] √(1+𝑥−𝑥^2 )〗 𝑑𝑥 Let 1 + 𝑥 − 𝑥^2 = t Diff. both sides w.r.t.x 0 + 1 −2x = 𝑑𝑡/𝑑𝑥 (1 − 2x) dx = dt dx = 𝑑𝑡/(1 − 2𝑥) Thus, our equation becomes I_1 = (−1)/2 ∫1▒〖[1−2𝑥] √(1+𝑥−𝑥^2 )〗 𝑑𝑥 Putting the value if (1+𝑥−𝑥^2) and dx, we get I_1 = (−1)/2 ∫1▒〖[1−2𝑥] √𝑡〗. 𝑑𝑥 I_1 = (−1)/2 ∫1▒〖[1−2𝑥] √𝑡〗. 𝑑𝑡/[1 − 2𝑥] 𝐼_1 = (−1)/2 ∫1▒√𝑡. 𝑑𝑡 I_1 = (−1)/2 ∫1▒〖(𝑡)〗^(1/2) 𝑑𝑡 I_1 = (−1)/2 〖𝑡 〗^(1/2 + 1)/((1/2 + 1) )+ C_1 I_1 = (−1)/2 〖𝑡 〗^(3/2 )/((3/2) )+ C_1 I_1 = (−1)/3 〖𝑡 〗^(3/2 )+ C_1 I_1 = (−1)/3 〖(1−𝑥 −𝑥^2 ) 〗^(3/2 )+ C_1 ("Using t = " 1−𝑥 −𝑥^2 ) Solving 𝑰_𝟐 I_2 = 1/2 ∫1▒√(1+𝑥−𝑥^2 ) 𝑑𝑥 I_2 = 1/2 ∫1▒√(−(𝑥^2−𝑥−1)) 𝑑𝑥 I_2 = 1/2 ∫1▒√(−[𝑥^2−2(𝑥)(1/2)−1] ) 𝑑𝑥 I_2 = 1/2 ∫1▒〖√(−[𝑥^2−2(𝑥)(1/2)+(1/2)^2−(1/2)^2−1] ) 〗 𝑑𝑥 I_2 = 1/2 ∫1▒〖√(−[(𝑥−1/2)^2−(1/2)^2−1] ) 〗 𝑑𝑥 I_2 = 1/2 ∫1▒〖√(−[〖 (𝑥−1/2)〗^2+(−1 −4)/4] ) 〗 𝑑𝑥 I_2 = 1/2 ∫1▒〖√(−[〖 (𝑥−1/2)〗^2 −5/4] ) 〗 𝑑𝑥 I_2 = 1/2 ∫1▒〖√(5/4 〖 −(𝑥−1/2)〗^2 ) 〗 𝑑𝑥 I_2 = 1/2 ∫1▒〖√((√5/4)^2 〖 −(𝑥−1/2)〗^2 ) 〗 𝑑𝑥 I_2 = 1/2 ((𝑥 − 1/2)/2 √((√5/4)^2 〖 −(𝑥−1/2)〗^2 )+(√5/2)^2/2 〖𝑠𝑖𝑛〗^(−1) ((𝑥 + 1/2)/(√5/2))+ C_2 ) I_2 = 1/2 (((2𝑥 −1)/2)/2 √(5/4−[𝑥^2 +1/4−2𝑥(1/2)] ) +(5/4)/2 〖𝑠𝑖𝑛〗^(−1) (((2𝑥 − 1)/2)/(√5/2))+ C_2 ) It is of form √(𝑎^2−𝑥^2 ) 𝑑𝑥=1/2 𝑥√(𝑎^2−𝑥^2 )+𝑎^2/2 〖𝑠𝑖𝑛〗^(−1) 𝑥/𝑎+ C_2 Replacing x by (x – 1/2) and a by √5/2 , we get I_2 = 1/2 ((2𝑥 −1)/4 √(5/4−[𝑥^2 +1/4 −𝑥] ) +5/8 〖𝑠𝑖𝑛〗^(−1) ((2𝑥 − 1)/√5)+ C_2 ) I_2 " = " (2𝑥 −1)/8 √(1+𝑥+𝑥^2 ) +5/16 〖𝑠𝑖𝑛〗^(−1) ((2𝑥 − 1)/√5)+C_3 Putting the value of I_1 and I_2 in (1) ∫1▒𝑥 √(1+ 𝑥−𝑥^2 ) d𝑥 = (−1)/2 ∫1▒〖[1−2𝑥] √(1+𝑥−𝑥^2 )〗 𝑑𝑥+∫1▒√(1+𝑥−𝑥^2 ) 𝑑𝑥 = (−1)/3 〖(1+𝑥−𝑥^2)〗^(3/2) + C_1 + ((2𝑥 −1))/8 √(1+𝑥−𝑥^2 )+5/16 〖𝑠𝑖𝑛〗^(−1) ((2𝑥 − 1)/√5)+ C_3 = (−𝟏)/𝟑 〖(𝟏+𝒙−𝒙^𝟐)〗^(𝟑/𝟐) +𝟏/𝟖 (𝟐𝒙 −𝟏) √(𝟏+𝒙−𝒙^𝟐 )+ 𝟓/𝟏𝟔 〖𝒔𝒊𝒏〗^(−𝟏) ((𝟐𝒙 − 𝟏)/√𝟓)+ 𝑪