Question 1 (i) - Difficult Polynomial Questions - Chapter 2 Class 10 Polynomials

Transcript

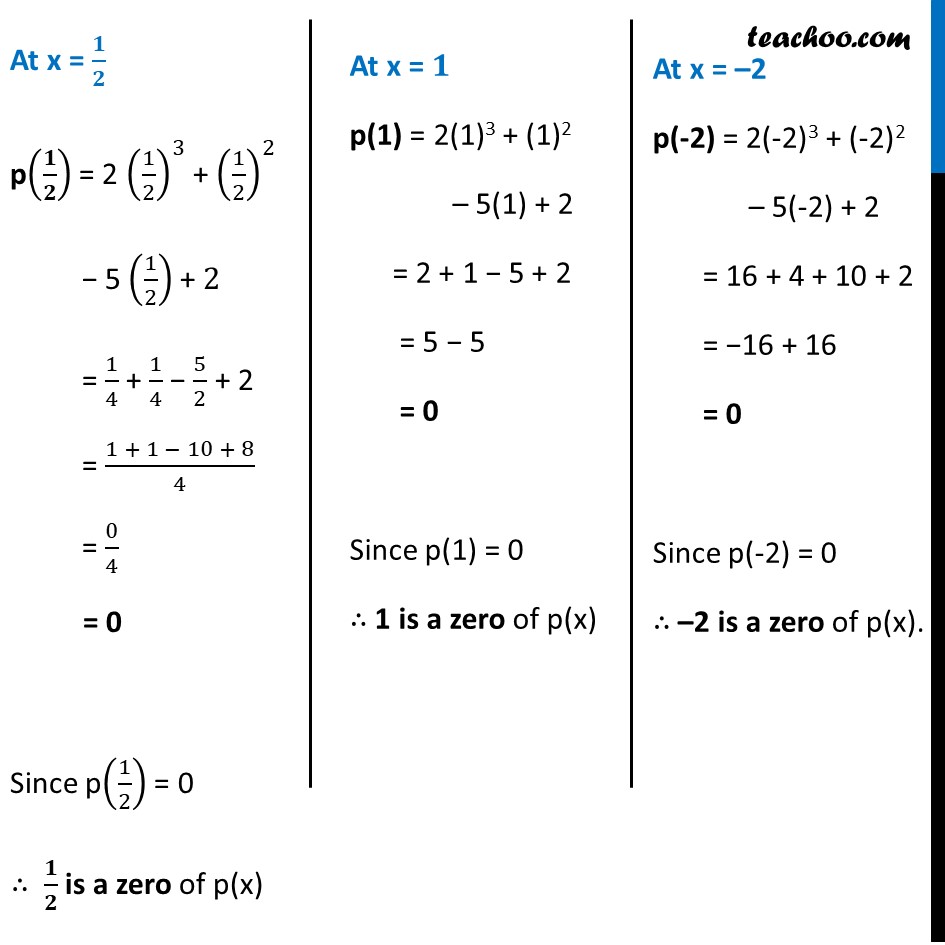
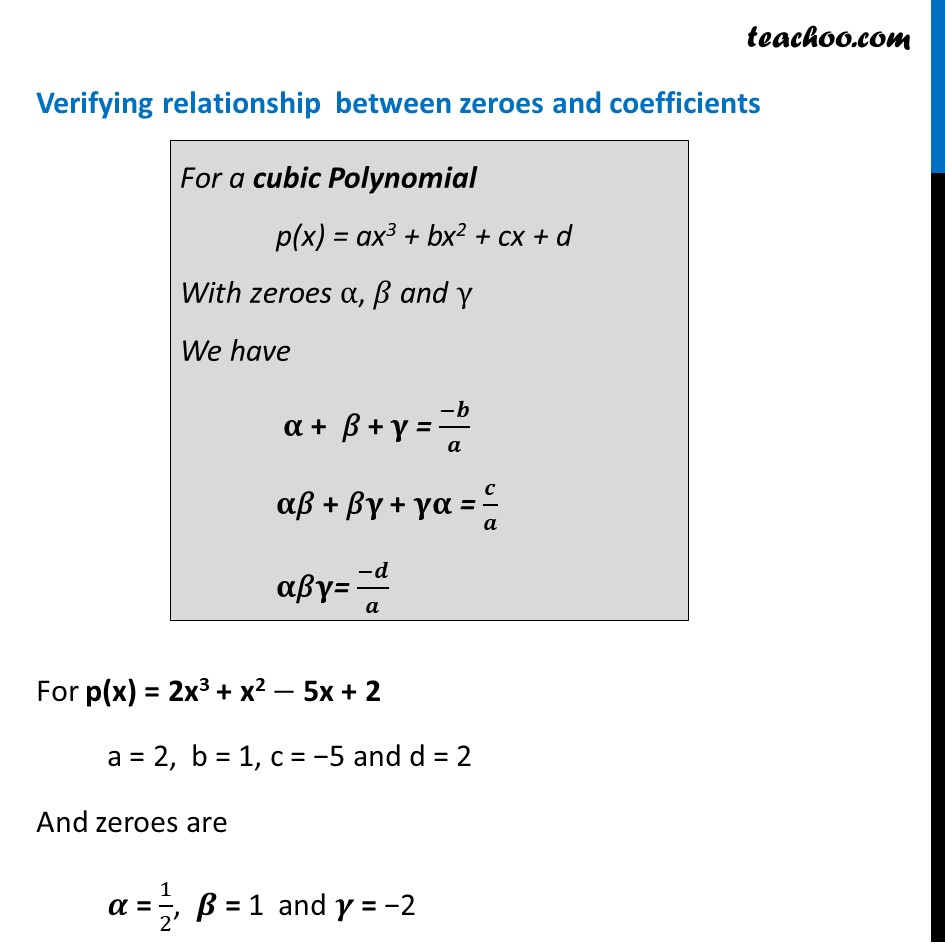
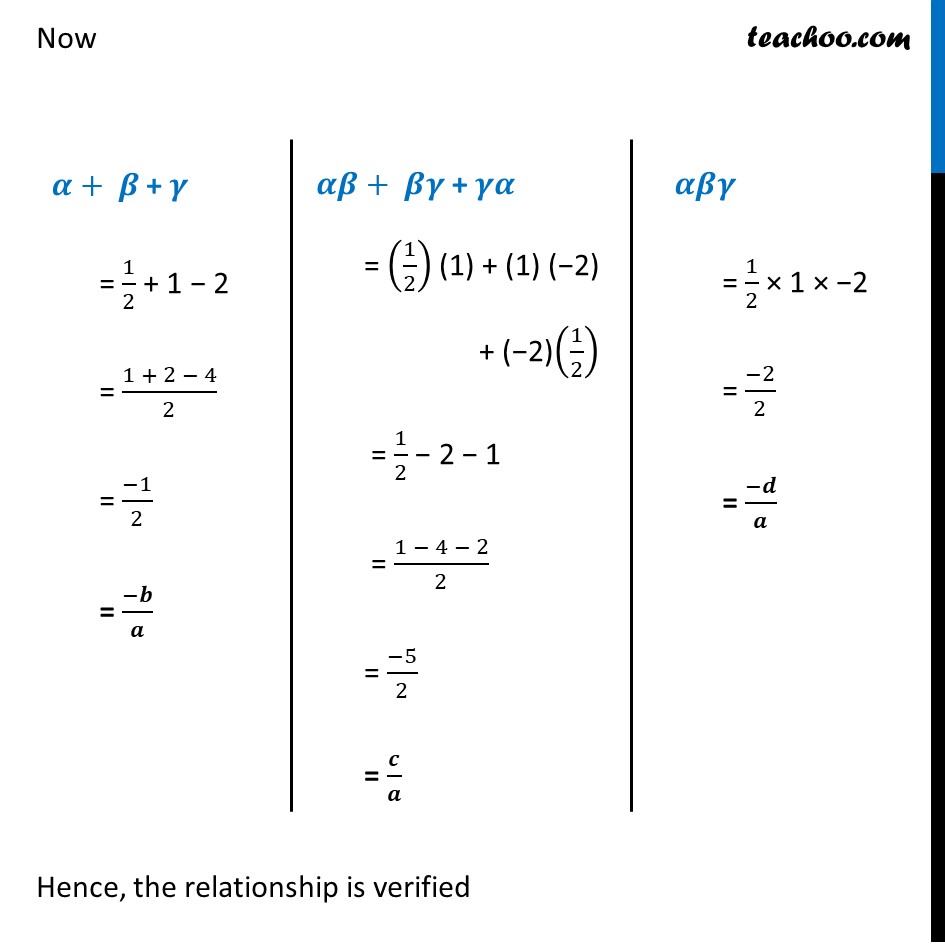
Question 1 - Polynomials Class 10 Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeros and the coefficients in each case: (i) 2x^3 + x^2 - 5x + 2; 1/2, 1, -2 At x = 𝟏/𝟐 p(𝟏/𝟐) = 2 (1/2)^3 + (1/2)^2 − 5 (1/2) + 2 = 1/4 + 1/4 − 5/2 + 2 = (1 + 1 − 10 + 8)/4 = 0/4 = 0 Since p(1/2) = 0 ∴ 𝟏/𝟐 is a zero of p(x) At x = 𝟏 p(1) = 2(1)3 + (1)2 – 5(1) + 2 = 2 + 1 − 5 + 2 = 5 − 5 = 0 Since p(1) = 0 ∴ 1 is a zero of p(x) At x = –2 p(-2) = 2(-2)3 + (-2)2 – 5(-2) + 2 = 16 + 4 + 10 + 2 = −16 + 16 = 0 Since p(-2) = 0 ∴ –2 is a zero of p(x). At x = 𝟏/𝟐 p(𝟏/𝟐) = 2 (1/2)^3 + (1/2)^2 − 5 (1/2) + 2 = 1/4 + 1/4 − 5/2 + 2 = (1 + 1 − 10 + 8)/4 = 0/4 = 0 Since p(1/2) = 0 ∴ 𝟏/𝟐 is a zero of p(x) Verifying relationship between zeroes and coefficients For p(x) = 2x3 + x2 − 5x + 2 a = 2, b = 1, c = −5 and d = 2 And zeroes are 𝜶 = 1/2, 𝜷 = 1 and 𝜸 = −2 For a cubic Polynomial p(x) = ax3 + bx2 + cx + d With zeroes α, 𝛽 and γ We have 𝛂 + 𝛽 + 𝛄 = (−𝒃)/𝒂 𝛂"𝛽" + 𝛽𝛄 + 𝛄𝛂 = 𝒄/𝒂 𝛂"𝛽" 𝛄= (−𝒅)/𝒂 Now 𝜶+ 𝜷 + 𝜸 = 1/2 + 1 − 2 = (1 + 2 − 4)/2 = (−1)/2 = (−𝒃)/𝒂