Construction 11.5 - Constructions

Transcript

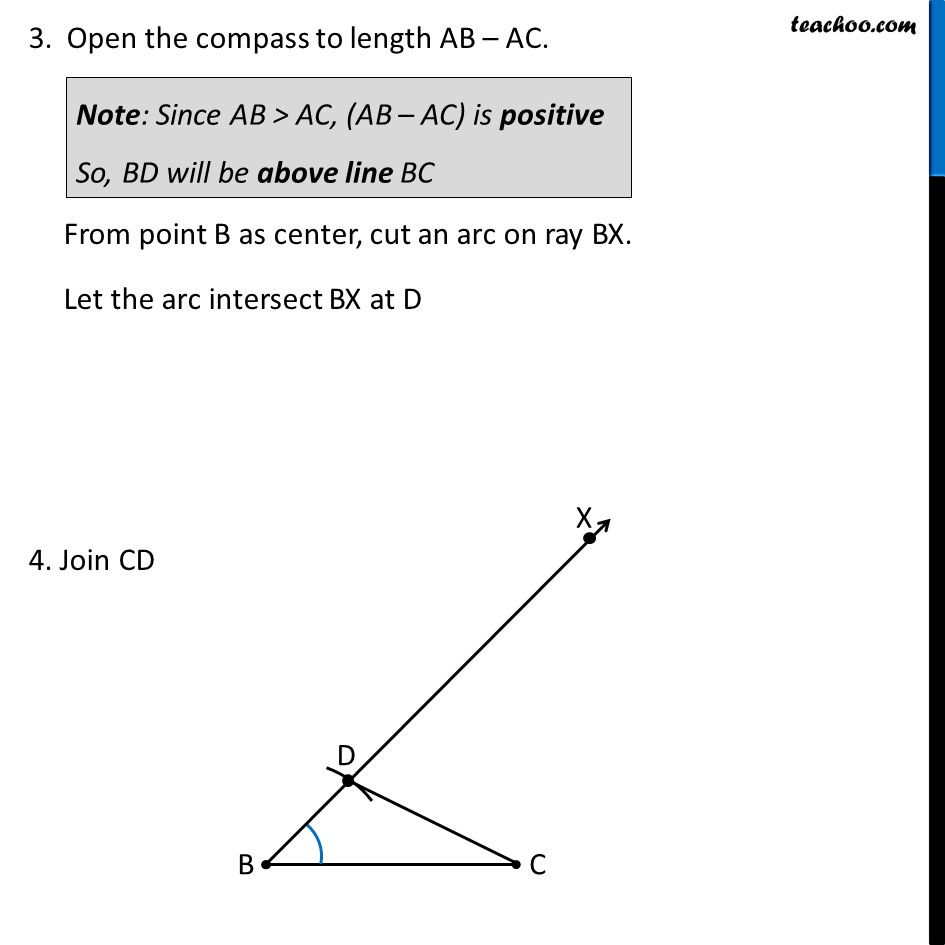
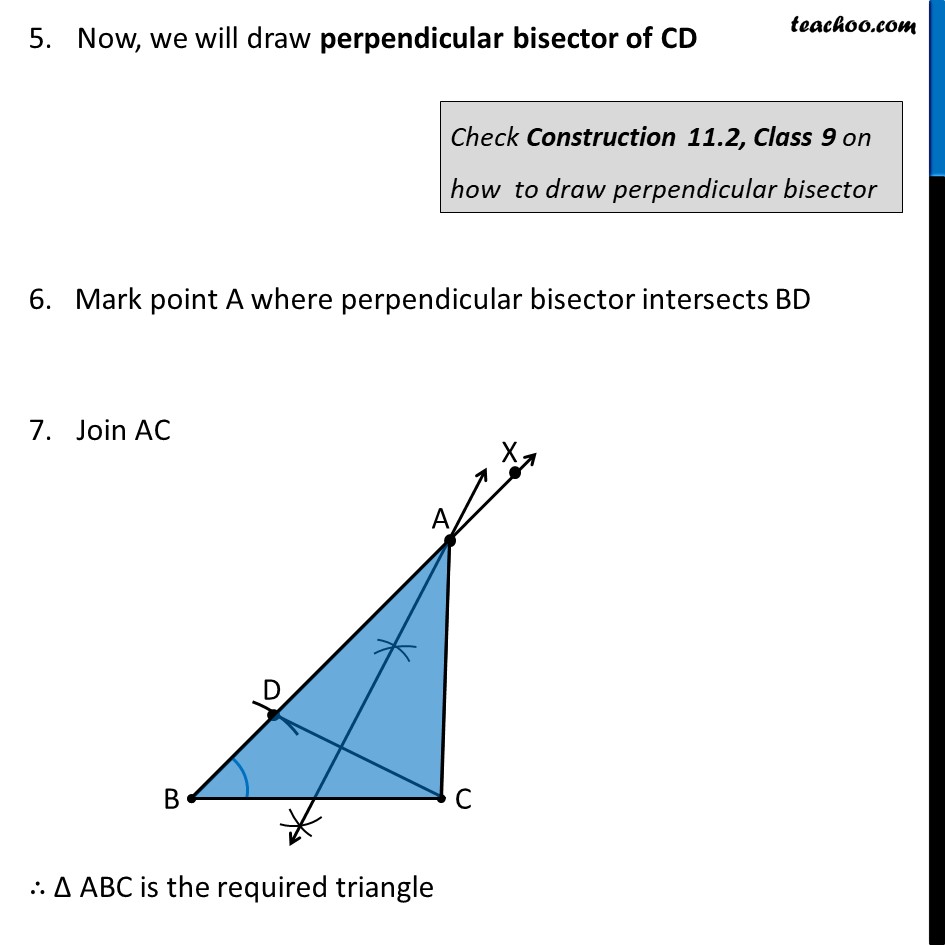
Construction 11.5 : To construct a triangle, given its base, a base angle and the difference of the other two sides. Let Δ ABC with ∠ B and base BC be given Now, we are also given difference of other two sides So, There can be two cases AB > AC and difference AB – AC is given AC > AB and difference AC – AB is given Let’s do both these cases separately Case 1: AB > AC Given the base BC, a base angle ∠B and the difference AB – AC, we need to construct Δ ABC Steps of Construction: Draw base BC 2. Now, let’s draw ∠ B Construct angle B from point B. Let the ray be BX 3. Open the compass to length AB – AC. From point B as center, cut an arc on ray BX. Let the arc intersect BX at D 4. Join CD Note: Since AB > AC, (AB – AC) is positive So, BD will be above line BC Now, we will draw perpendicular bisector of CD 6. Mark point A where perpendicular bisector intersects BD Join AC ∴ Δ ABC is the required triangle Check Construction 11.2, Class 9 on how to draw perpendicular bisector Justification – Case 1 We need to prove that AB – AC = BD. Let perpendicular bisector intersect CD at point R Thus, AR is the perpendicular bisector of CD ∴ CR = DR & ∠ ARC = ∠ ARD = 90° Now, In Δ ADR and Δ ACR AR = AR ∠ ARD = ∠ ARC DR = CR ∴ Δ ADR ≅ Δ ACR ⇒ AC = AD Now, BD = AB – AD BD = AB – AC Thus, our construction is justified (Common) (From (2)) (From (1)) (SAS Congruency) (CPCT) (From (3)) Case 2: AC > AB Given the base BC, a base angle ∠B and the difference AC – AB, we need to construct Δ ABC Steps of Construction: Draw base BC 2. Now, let’s draw ∠ B Construct angle B from point B. Let the ray be BX Open the compass to length AC – AB. From point B as center, cut an arc on ray BX (opposite side of BC). Let the arc intersect BX at D 4. Join CD Note: Since AC > AB, (AB – AC) is negative So, BD will be below line BC Now, we will draw perpendicular bisector of CD 6. Mark point A where perpendicular bisector intersects BD Join AC ∴ Δ ABC is the required triangle Check Construction 11.2, Class 9 on how to draw perpendicular bisector Justification - Case 2 We need to prove that AC – AB = BD. Let perpendicular bisector intersect CD at point R Thus, AR is the perpendicular bisector of CD ∴ CR = DR & ∠ ARC = ∠ ARD = 90° Now, In Δ ADR and Δ ACR AR = AR ∠ ARD = ∠ ARC DR = CR ∴ Δ ADR ≅ Δ ACR ⇒ AC = AD Now, BD = AD – AB BD = AC – AB Thus, our construction is justified (Common) (From (2)) (From (1)) (SAS Congruency) (CPCT)