Integration Formula Sheet

Check the formula sheet of integration. 

Topics include

1. Basic Integration Formulas
2. Integral of special functions
3. Integral by Partial Fractions
4. Integration by Parts
5. Other Special Integrals
6. Area as a sum
7. Properties of definite integration 

 Integration of Trigonometric Functions, Properties of Definite Integration are all mentioned here.

Basic Formula

1. ∫x ⁿ   = x ⁿ⁺¹ /n+1  + C
2. ∫cos x    = sin x  + C
3. ∫sin x    = -cos x  + C
4. ∫sec ² x    = tan x  + C
5. ∫cosec ² x    = -cot x  + C
6. ∫sec x tan x    = sec x  + C
7. ∫cosec  x cot x    = -cosec x  + C
8. ∫dx/√ 1- x ²  = sin ^-1  x  + C
9. ∫dx/√ 1- x ²  = -cos ^-1  x  + C
10. ∫dx/√ 1+ x ²  = tan ^-1  x  + C
11. ∫dx/√ 1+ x ²  = -cot ^-1  x  + C
12. ∫e ^x   = e ^x  + C
13. ∫a ^x   = a ^x / log a + C
14. ∫dx/x √ x ²   - 1= sec ^-1  x  + C
15. ∫dx/x √ x ²   - 1= cosec ^-1  x  + C
16. ∫1/x    = log |x| + c
17. ∫tan x    = log |sec x| + c
18. ∫cot x    = log |sin x| + c
19. ∫sec x    = log |sec x + tan x| + c
20. ∫cosec x    = log |cosec x - cot x| + c

Practice Basic Formula questions - Part 1 and Basic Formula questions - Part 2.

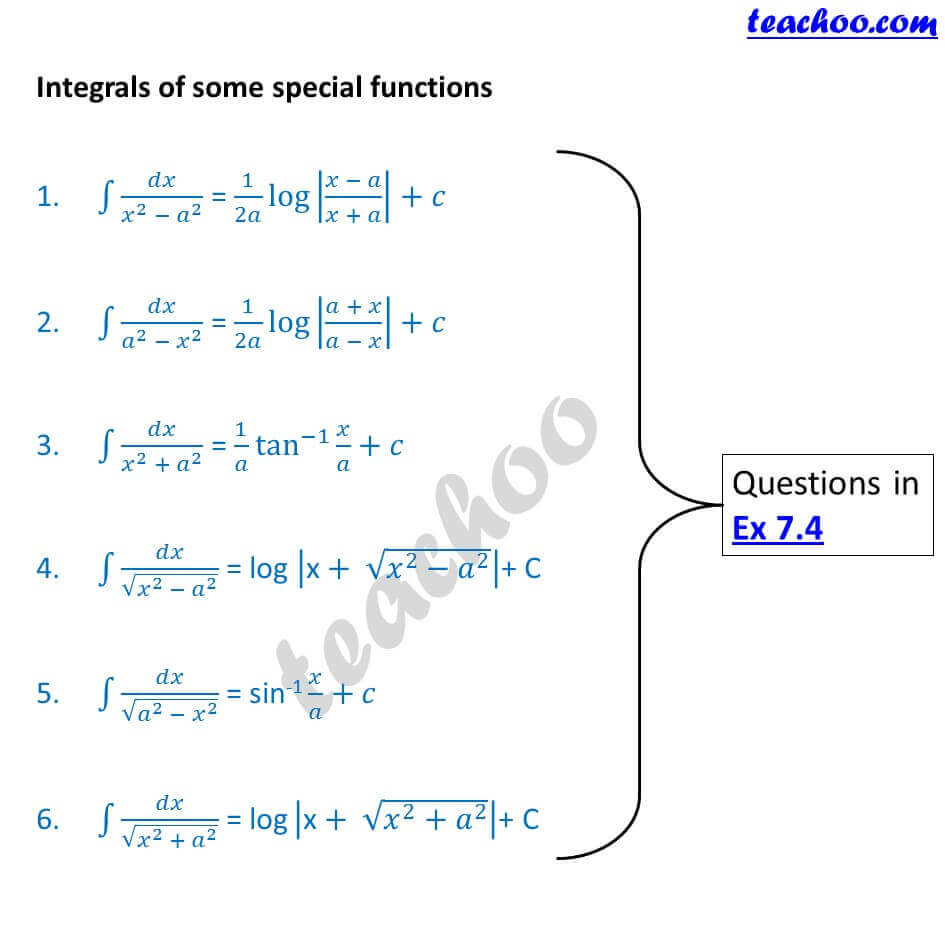
Integrals of some special function s

1.  ∫dx/(x ²   - a ² ) = 1/2a  log⁡ |(x - a) / (x + a)| + c
2.  ∫dx/(a ²   - x ² ) = 1/2a  log⁡ |(a + x) / (a - x)| + c
3. ∫dx / (x ²   + a ² ) = 1/a  tan ^(-1) ⁡ x / a + c

4. ∫dx / √(x ²   - a ² ) = log |"x" + √(x ² -a ² )| + C

5. 1.∫dx / √(a ²   - x ² ) = sin-1 x / a + c

6. ∫dx / √(x ² + a ² ) = log |"x" + √(x ² + a ² )| + C

Check Practice Questions

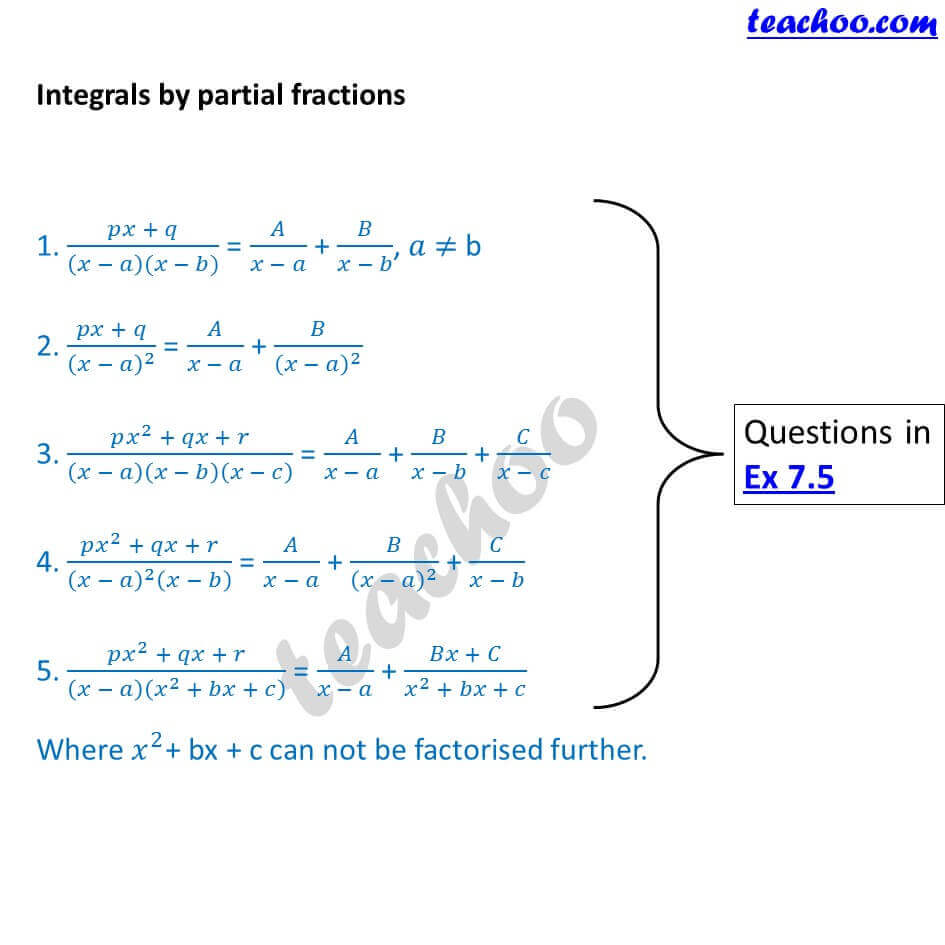
Integrals by partial fractions

1. (px + q) / ((x - a) (x - b)) = A/(x - a) + B / (x - b)

2. (px + q) / (x - a) ²  = A/(x - a) + B / (x - a) ²   

3. (px ²   + qx + r) / (x - a) (x - b) (x - c)  = A / (x - a) + B / (x - b) + C / (x - c)
4. (px ² + qx + r) / ((x - a) ² (x - b) ) = A / (x - a) + B / (x - a) ² + C / (x - b)
5. (px ² + qx + r) / (x - a) (x ² + bx + c)  = A / (x - a) + (Bx + C) / (x ² + bx + c)
   

   Where x ² + bx + c can not be factorised further.

Check Practice Questions

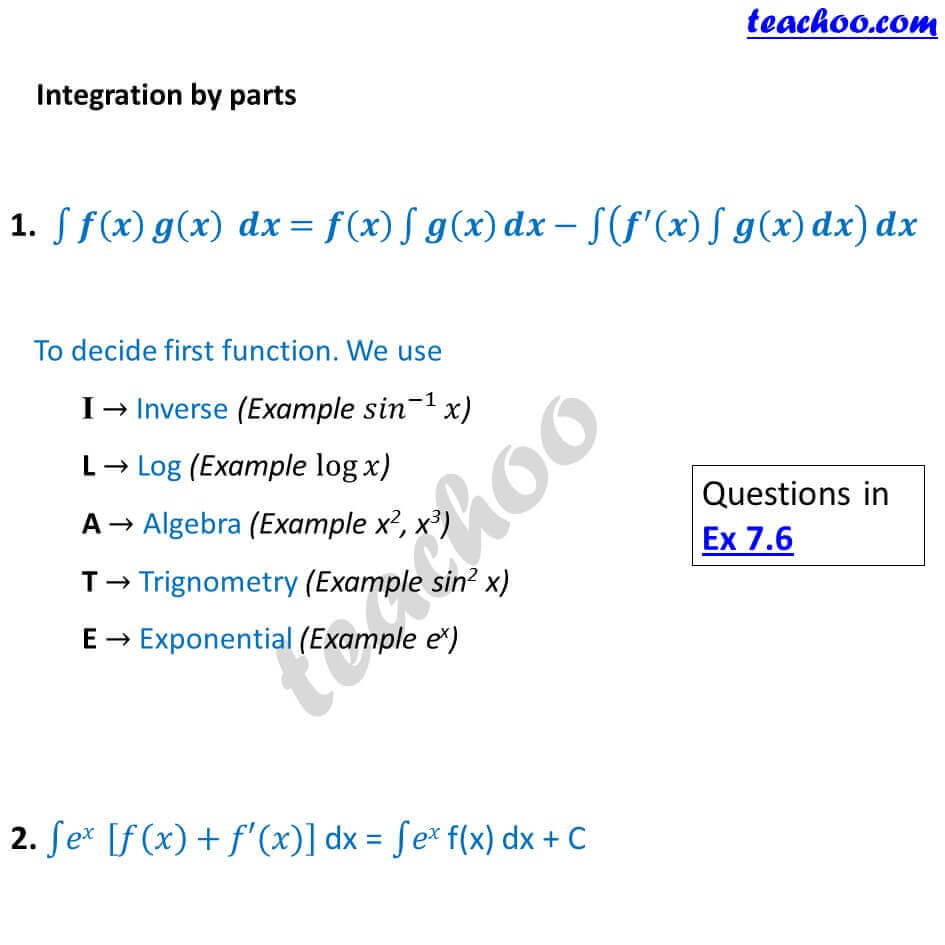
Integration by parts

1. ∫𝒇(𝒙) 𝒈⁡(𝒙)  𝒅𝒙 = 𝒇(𝒙) ∫𝒈 (𝒙) 𝒅𝒙− ∫(𝒇 ' (𝒙) ∫𝒈(𝒙) 𝒅𝒙) 𝒅𝒙
   
   

   To decide first function. We use

   I → Inverse (Example sin ^(-1)  ⁡x)

   L → Log (Example log ⁡x)

   A → Algebra (Example x ² , x ³ )

   T → Trigonometry (Example sin ² x)

   E → Exponential (Example e ^x )
   
   

2. ∫ex [f (x) + f ′(x)] dx = ∫ex f(x) dx + C

Check Practice Questions

Other Special Integrals

1.  ∫√ (x ²  - a ² ) dx = x / 2 √(x ²  - a ² ) − a ²  / 2 log |x + √(x ²  - a ² )| + C 

2. ∫ √( x ²  + a ² ) dx = x / 2 √(x ²  + a ² ) + a ²  / 2 log |x +√(x ²  + a ² )| + C 

3. ∫ √( a ²  - x ² ) dx = x / 2 √(a ²   - x ² ) + a ²  / 2 sin ¹ x / a + C

Check Practice Questions

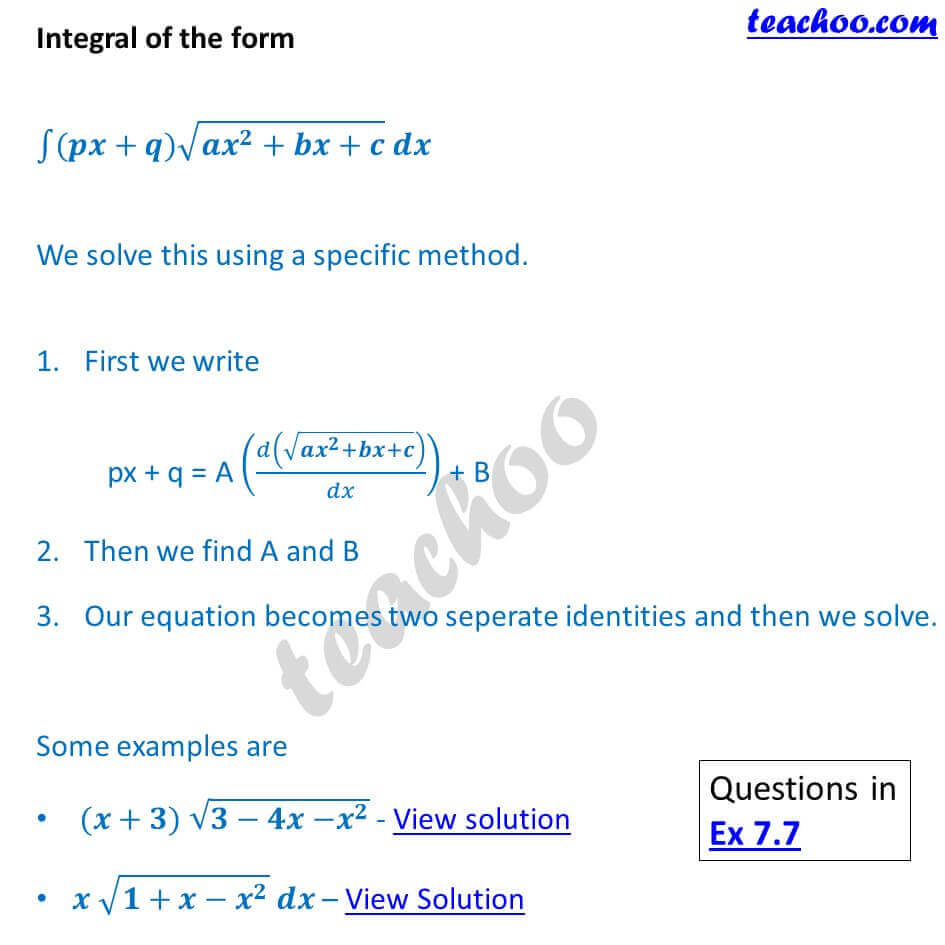
Integral of the form  ∫ (px+q) √( ax ²  + bx + c )  dx

We solve this using a specific method.

1. First we write
        px + q = A (d(√(ax ²  + bx + c))/dx) + B
2. Then we find A and B
3. Our equation becomes two seperate identities and then we solve.

Some examples are

• (x + 3) √( 3 - 4x - x ² ) - View solution
• x √(1 + x - x ² ) dx – View Solution

Check Practice Questions

Area as a sum

∫a→b f (x)  dx = (b - a)  (lim) (n→∞)  1 / n (f (a) + f (a + h) + f (a + 2h)…+ f (a + (n - 1) h))

Check Practice Questions

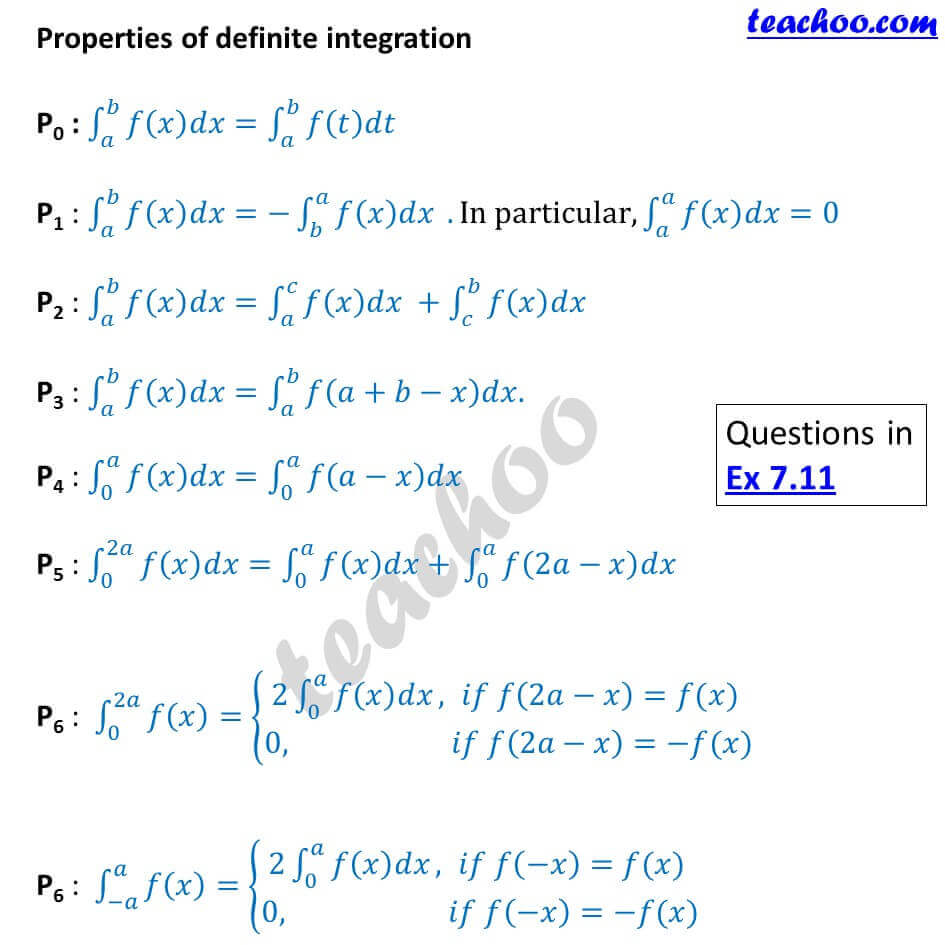
Properties of definite integration

1. P ₀ : ∫a→b   f(x) dx = ∫a→b   f(t) dt
2. P ₁ : ∫a→b   f(x) dx = -∫b→a   f(x) dx .In particular, ∫a→a   f(x) dx = 0
3. P ₂ : ∫a→b   f(x) dx = ∫a→c f(x) dx + ∫c→b f(x) dx
4. P ₃ : ∫a→b f(x) dx= ∫a→b   f(a + b - x) dx.
5. P ₄ : ∫0→a f(x)dx = ∫0→a   f(a - x) dx
6. P ₅ : ∫0→2a   f(x) dx = ∫0→a   f(x) dx + ∫0→a f(2a - x) dx
7. P ₆ :  ∫0→2a f(x) = {(2∫0→a   f(x) dx,  if f (2a - x) = f (x) , if f (2a - x) = -f(x))
8. P ₇ :  ∫(-a)→a f(x) = {(2∫0→a f(x) dx,  if f(-x) = f(x), if f ( -x) = -f(x)

Check Practice Questions

You can also download the pdf here