

Standard deviation and variance - Ungrouped data
Standard deviation and variance - Ungrouped data
Last updated at Dec. 16, 2024 by Teachoo



Transcript
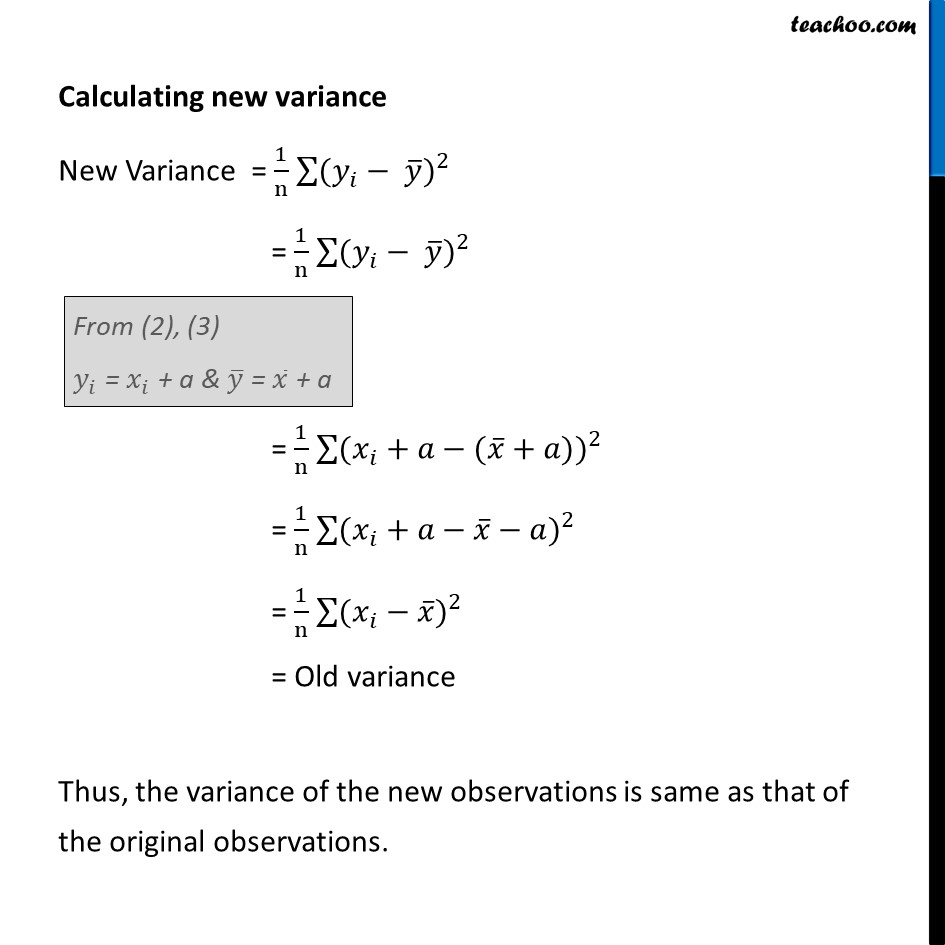
Example 15 If each observation 𝑥1, 𝑥2, 𝑥3, ..., 𝑥𝑛 is increased by a, where a is a negative or positive number, show that the variance remains unchanged. Let the mean of the observations 𝑥1, 𝑥2, 𝑥3, ..., 𝑥𝑛 be 𝑥 Variance of these observations is given by Old Variance = 1n ( 𝑥𝑖− 𝑥)2 If each observation is increased by a , we get new observations, Let the new observations be 𝑦1, 𝑦2, 𝑦3, ..., 𝑦𝑛 where 𝑦𝑖 = 𝑥𝑖 + a We need to find variance of the new observations i.e. New Variance = 1n ( 𝑦𝑖− 𝑦)2 Now, We know 𝑦𝑖 = 𝑥𝑖 + a Calculating 𝑦 in terms of 𝑥, 𝑦 = 1𝑛 𝑦𝑖 𝑦 = 1𝑛 ( 𝑥𝑖 + a) 𝑦 = 1𝑛 𝑥𝑖 + 𝑎 𝑦 = 1𝑛 𝑥𝑖 + 1𝑛 𝑎 𝑦 = 𝑥 + 1𝑛 × n(a) 𝑦 = 𝑥 + a Calculating new variance New Variance = 1n ( 𝑦𝑖− 𝑦)2 = 1n ( 𝑦𝑖− 𝑦)2 = 1n ( 𝑥𝑖+𝑎−( 𝑥+𝑎))2 = 1n ( 𝑥𝑖+𝑎− 𝑥−𝑎)2 = 1n ( 𝑥𝑖− 𝑥)2 = Old variance Thus, the variance of the new observations is same as that of the original observations.