

Integration by parts
Integration by parts
Last updated at December 16, 2024 by Teachoo



Transcript
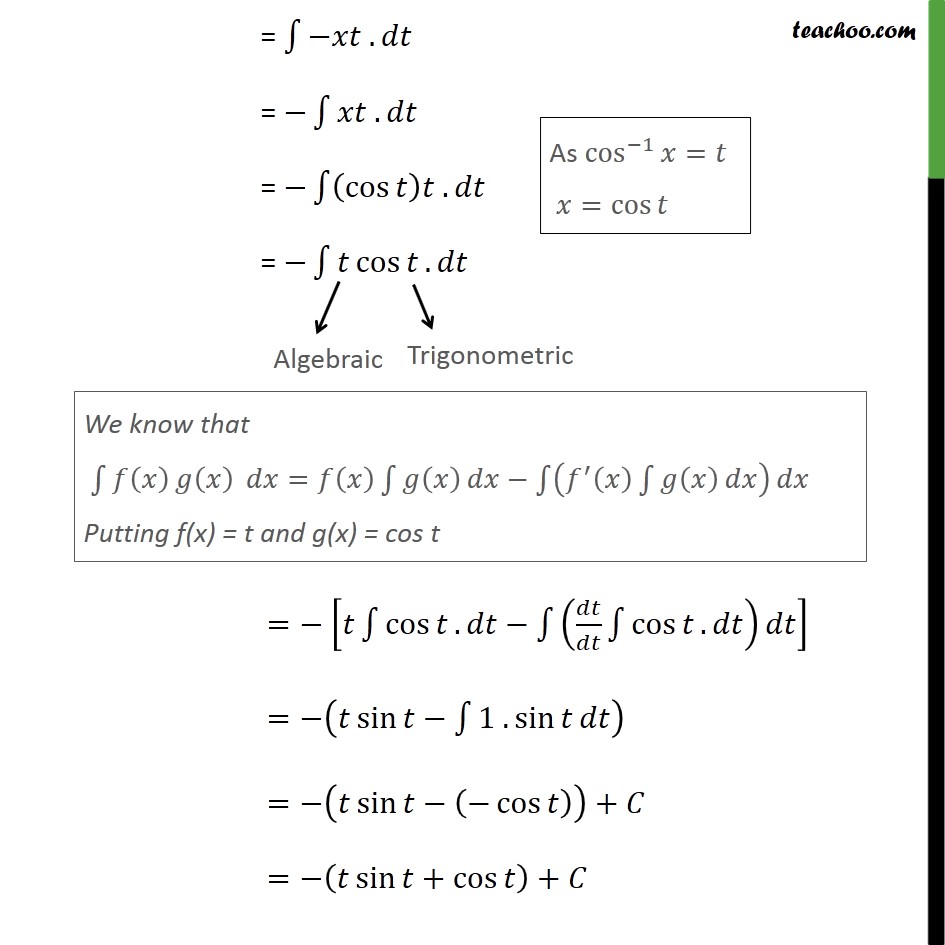
Ex 7.6, 11 𝑥 cos−1𝑥 1 − 𝑥2 Let cos−1𝑥=𝑡 Differentiating both sides 𝑤.𝑟.𝑡.𝑥 −1 1 − 𝑥2 = 𝑑𝑡𝑑𝑥 𝑑𝑥=− 1 − 𝑥2 𝑑𝑡 Putting the value of cos−1x = t and dx = − 1− x2 dt , we get 𝑥 cos−1𝑥 1 − 𝑥2 .𝑑𝑥 = 𝑥 . 𝑡 1 − 𝑥2 .𝑑𝑥 = 𝑥 . 𝑡 1 − 𝑥2 . − 1 − 𝑥2𝑑𝑡 = −𝑥𝑡 . 𝑑𝑡 = − 𝑥𝑡 . 𝑑𝑡 = − cos𝑡𝑡 . 𝑑𝑡 = − 𝑡 cos𝑡. 𝑑𝑡 =− 𝑡 cos𝑡. 𝑑𝑡− 𝑑𝑡𝑑𝑡 cos𝑡. 𝑑𝑡𝑑𝑡 =− 𝑡 sin𝑡− 1 . sin𝑡𝑑𝑡 =− 𝑡 sin𝑡− − cos𝑡+𝐶 =− 𝑡 sin𝑡+ cos𝑡+𝐶 =−𝑡 sin𝑡− cos𝑡+𝐶 =−𝑡 1− 𝑐𝑜𝑠2𝑡 −𝑐𝑜𝑠𝑡+𝐶 = − cos−1𝑥 1− 𝑥2−𝑥+𝐶 = − 1− 𝑥2 cos−1𝑥+𝑥+𝐶