
Integration by partial fraction - Type 1
Integration by partial fraction - Type 1
Last updated at Dec. 16, 2024 by Teachoo




Transcript
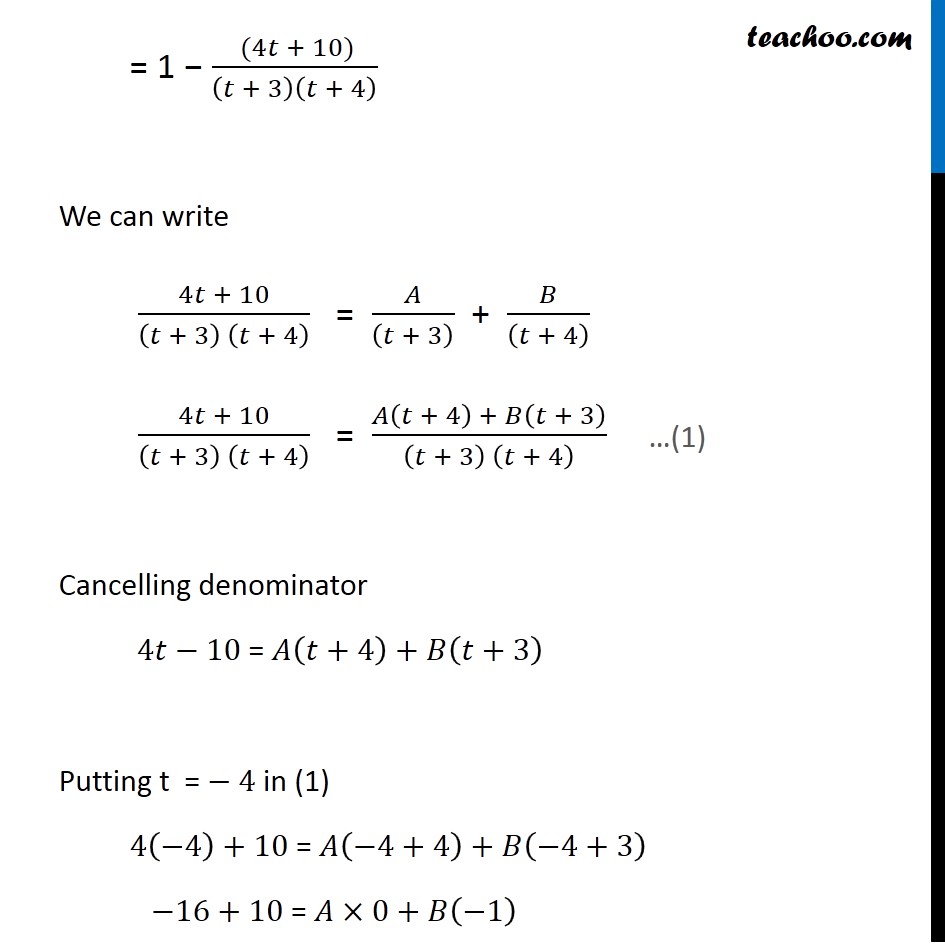
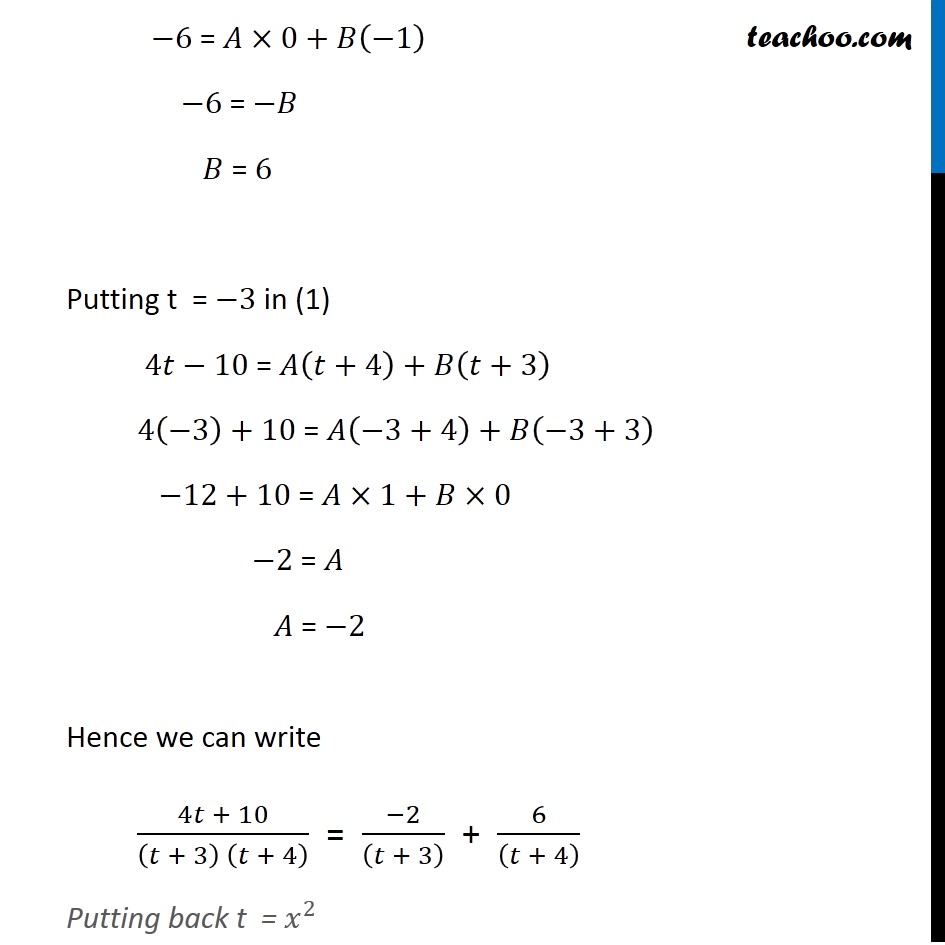
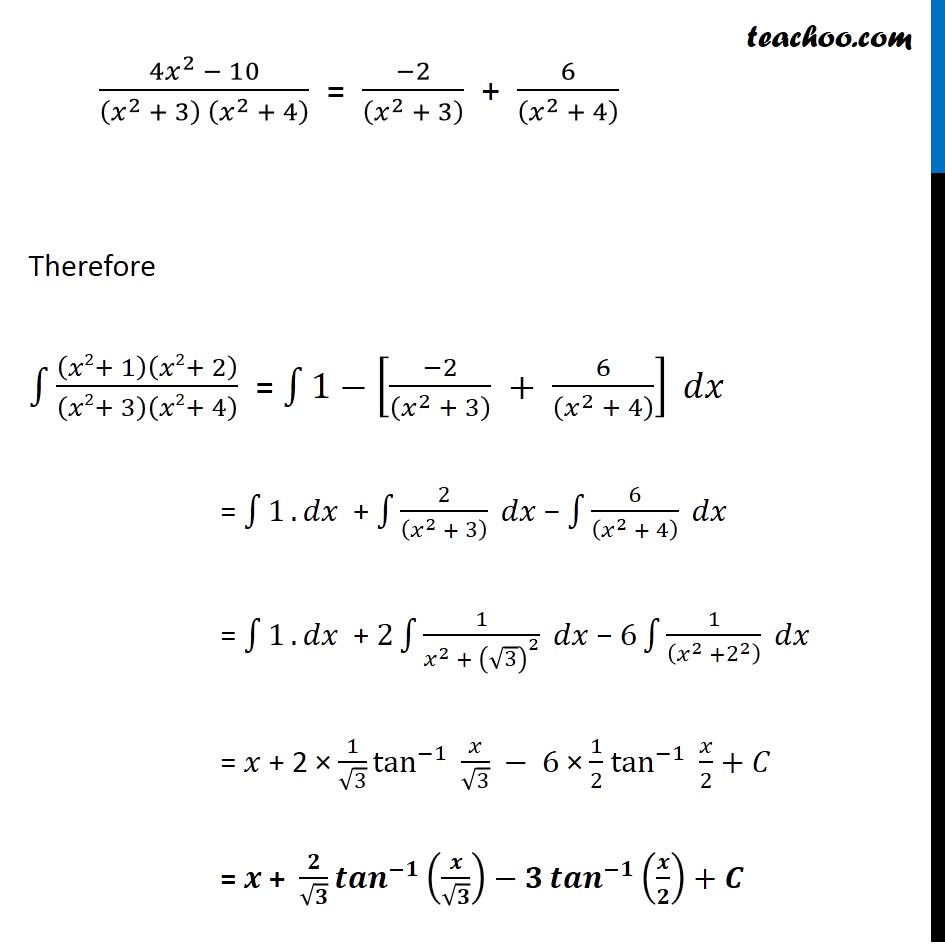
Ex 7.5, 18 Integrate the function (𝑥2+ 1)(𝑥2+ 2)/(𝑥2+ 3)(𝑥2+ 4) (𝑥^2 + 1)(𝑥^2 + 2)/(𝑥^2 + 3)(𝑥^2 + 4) " " Let t = 𝑥^2 = (𝑡 + 1)(𝑡 + 2)/(𝑡 + 3)(𝑡 + 4) = (𝑡^2 + 3𝑡 + 2)/(𝑡^2 + 7𝑡 + 12) = 1 + (−4𝑡 −10)/(𝑡^2 + 7𝑡 + 12) = 1 + (−(4𝑡 + 10))/(𝑡 + 3)(𝑡 + 4) Rough = 1 − ( (4𝑡 + 10))/(𝑡 + 3)(𝑡 + 4) We can write (4𝑡 + 10)/((𝑡 + 3) (𝑡 + 4) ) = 𝐴/((𝑡 + 3) ) + 𝐵/((𝑡 + 4) ) (4𝑡 + 10)/((𝑡 + 3) (𝑡 + 4) ) = (𝐴(𝑡 + 4) + 𝐵(𝑡 + 3))/((𝑡 + 3) (𝑡 + 4) ) Cancelling denominator 4𝑡−10 = 𝐴(𝑡+4)+𝐵(𝑡+3) Putting t = − 4 in (1) 4(−4)+10 = 𝐴(−4+4)+𝐵(−4+3) −16+10 = 𝐴×0+𝐵(−1) …(1) −6 = 𝐴×0+𝐵(−1) −6 = −𝐵 𝐵 = 6 Putting t = −3 in (1) 4𝑡−10 = 𝐴(𝑡+4)+𝐵(𝑡+3) 4(−3)+10 = 𝐴(−3+4)+𝐵(−3+3) −12+10 = 𝐴×1+𝐵×0 −2 = 𝐴 𝐴 = −2 Hence we can write (4𝑡 + 10)/((𝑡 + 3) (𝑡 + 4) ) = (−2)/((𝑡 + 3) ) + 6/((𝑡 + 4) ) Putting back t = 𝑥^2 (4𝑥^2 − 10)/((𝑥^2 + 3) (𝑥^2 + 4) ) = (−2)/((𝑥^2 + 3) ) + 6/((𝑥^2 + 4) ) Therefore ∫1▒(𝑥2+ 1)(𝑥2+ 2)/(𝑥2+ 3)(𝑥2+ 4) = ∫1▒〖1−[(−2)/((𝑥^2 + 3) ) + 6/((𝑥^2 + 4) )] 〗 𝑑𝑥 = ∫1▒1. 𝑑𝑥 + ∫1▒2/((𝑥^2 + 3) ) 𝑑𝑥 − ∫1▒6/((𝑥^2 + 4) ) 𝑑𝑥 = ∫1▒1. 𝑑𝑥 + 2∫1▒1/(𝑥^2 + (√3)^2 ) 𝑑𝑥 − 6∫1▒1/((𝑥^2 +2^2 ) ) 𝑑𝑥 = 𝑥 + 2 × 1/√3 tan^(−1)〖 𝑥/√3〗 − 6 × 1/2 tan^(−1)〖 𝑥/2〗+𝐶 = 𝒙 + 𝟐/√𝟑 〖𝒕𝒂𝒏〗^(−𝟏)(𝒙/√𝟑)−𝟑 〖𝒕𝒂𝒏〗^(−𝟏)(𝒙/𝟐)+𝑪