Ex 7.5, 17 - Chapter 7 Class 12 Integrals (Important Question)

Last updated at Dec. 16, 2024 by Teachoo

Ex 7.5, 17 - Integrate cos x / (1 - sin x) (2 - sin x) - Ex 7.5

Ex 7.5, 17 - Chapter 7 Class 12 Integrals - Part 2

Ex 7.5, 17 - Chapter 7 Class 12 Integrals - Part 3

Ex 7.5, 17 - Chapter 7 Class 12 Integrals - Part 4

Next: Ex 7.5, 18 Important → Go Ad-free

Transcript

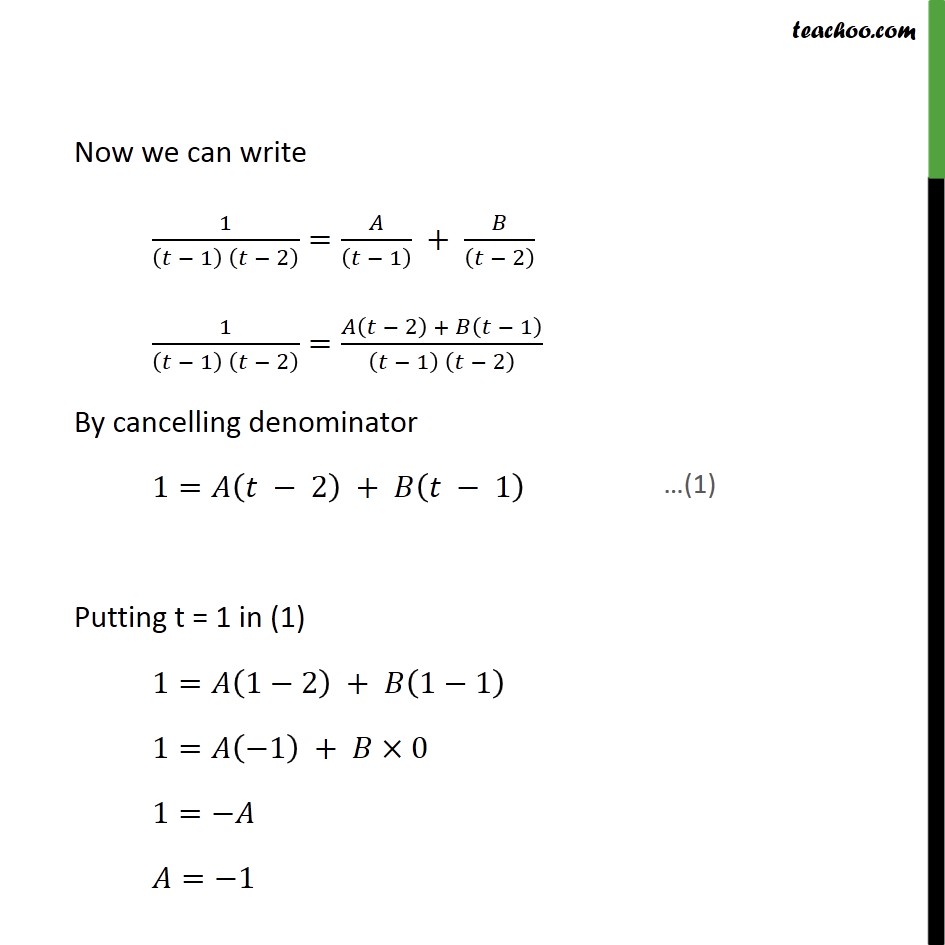
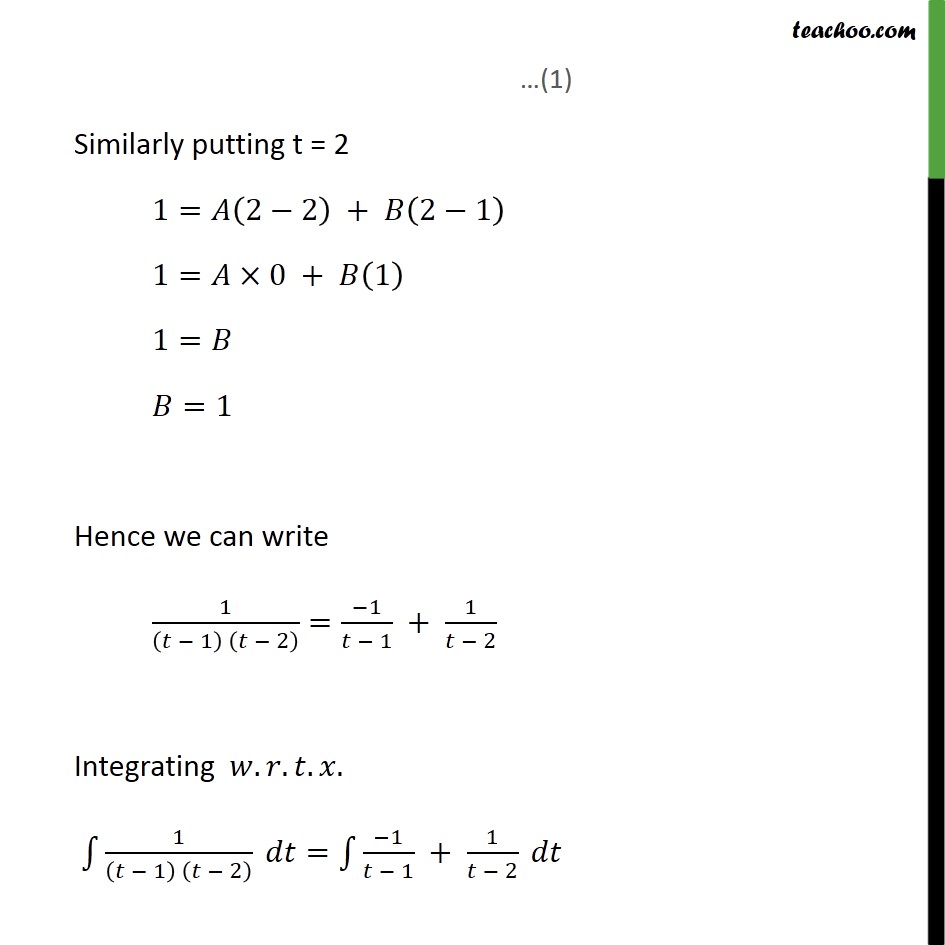
Ex 7.5, 17 cos (1 sin )(2 sin ) [Hint: Put sin x = t] Let sin = Diff. . . . = = Now we can write cos (1 sin )(2 sin ) = cos (1 )(2 ) = (1 )(2 ) Now we can write 1 1 2 = 1 + 2 1 1 2 = 2 + 1 1 2 By cancelling denominator 1= 2 + 1 Putting t = 1 in (1) 1= 1 2 + 1 1 1= 1 + 0 1= = 1 Similarly putting t = 2 1= 2 2 + 2 1 1= 0 + 1 1= =1 Hence we can write 1 1 2 = 1 1 + 1 2 Integrating . . . . 1 1 2 = 1 1 + 1 2 = log 1 + log 2 + Putting back t = = log sin 1 + log sin 2 + = log sin 2 log sin 1 + = log sin 2 sin 1 + = log 1 2 sin 1 1 sin + = +

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular