Chapter 7 Class 12 Integrals
Concept wise

Ex 7.5, 10 - Chapter 7 Class 12 Integrals

Last updated at Dec. 16, 2024 by

Ex 7.5, 10 - Integrate 2x - 3 / (x2 - 1) (2x + 3) - Integration by partial fraction - Type 3

Ex 7.5, 10 - Chapter 7 Class 12 Integrals - Part 2
Ex 7.5, 10 - Chapter 7 Class 12 Integrals - Part 3
Ex 7.5, 10 - Chapter 7 Class 12 Integrals - Part 4

Go Ad-free

Transcript

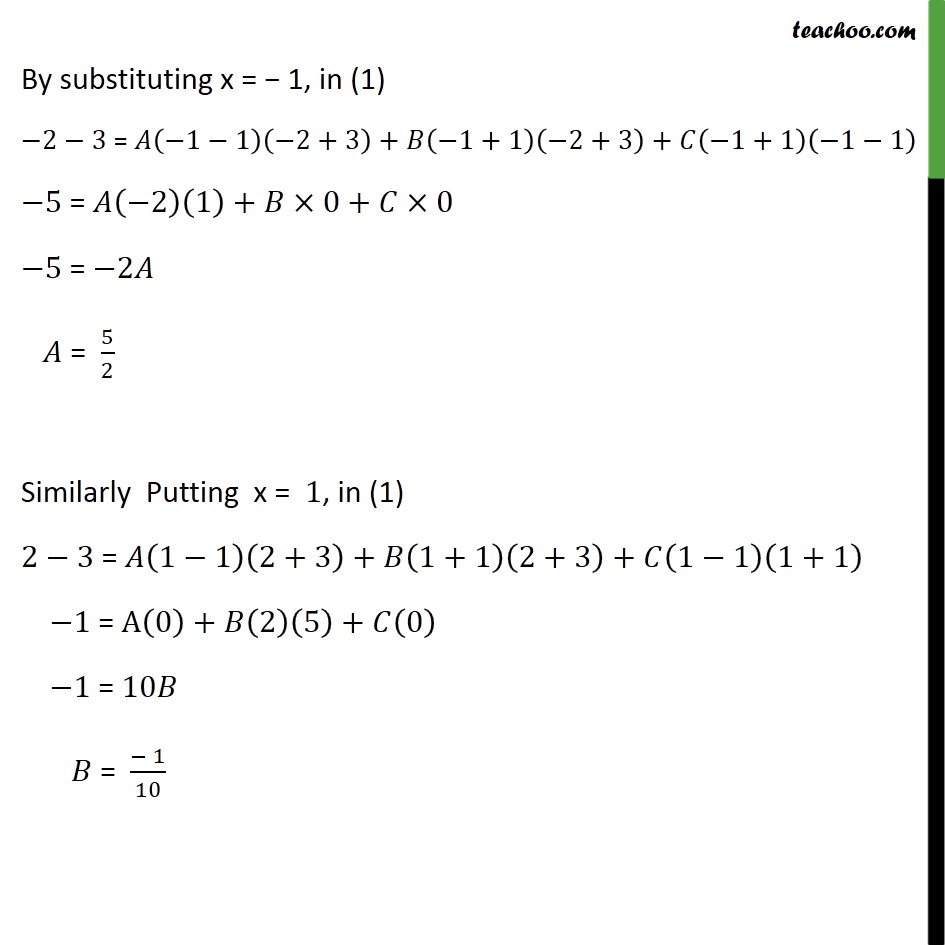
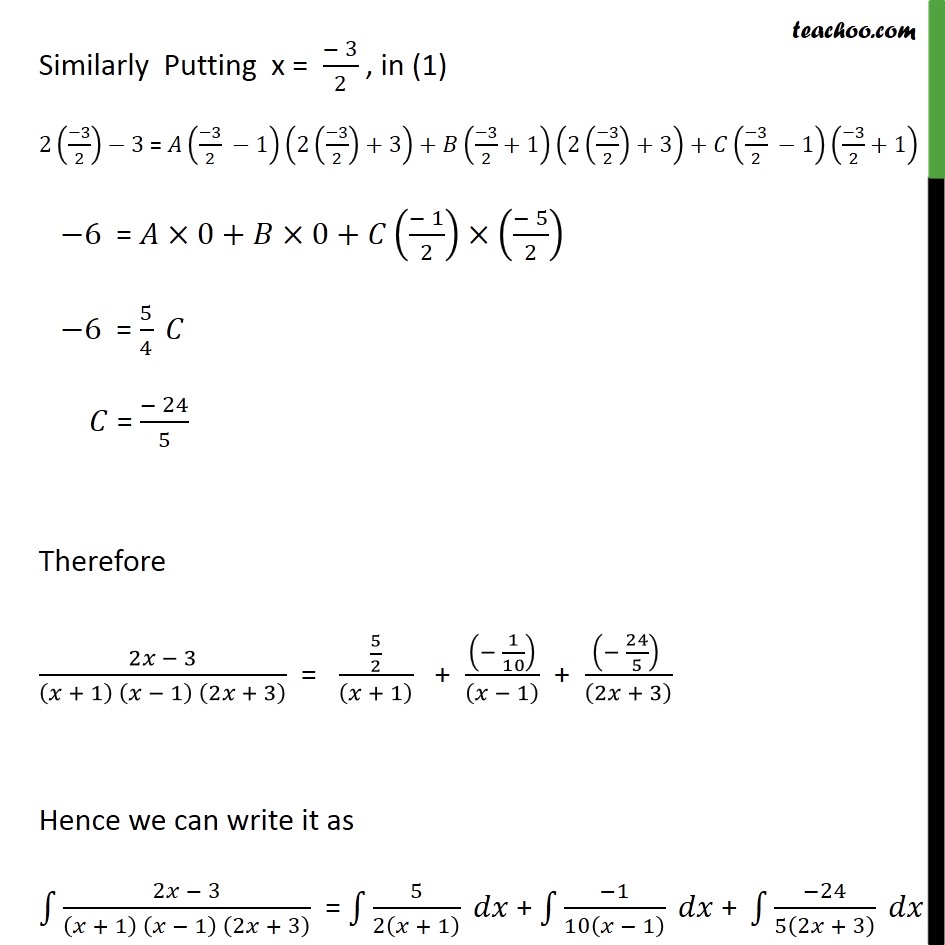
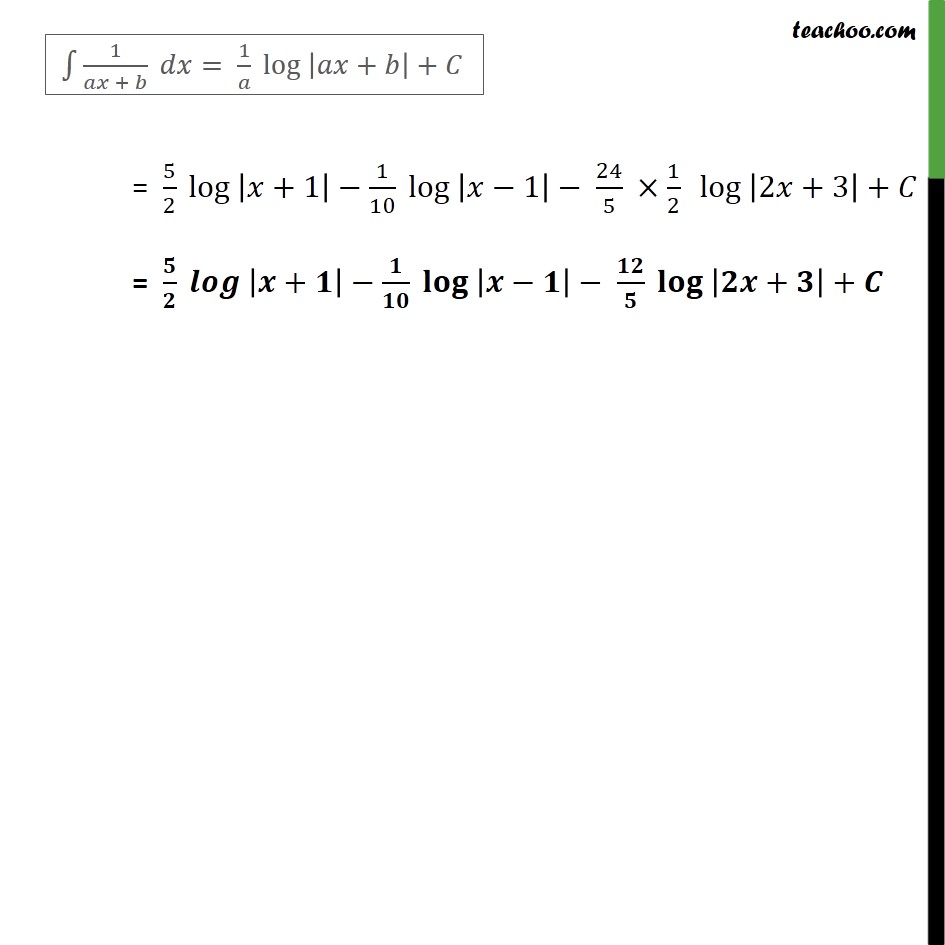
Ex 7.5, 10 2 3 2 1 2 + 3 We can write the integrand as 2 3 2 1 2 + 3 = 2 3 1 + 1 2 + 3 = + 1 + 1 + 2 + 3 = 1 2 + 3 + + 1 2 + 3 + 1 + 3 1 + 1 2 + 3 By cancelling denominator 2 3 = 1 2 +3 + +1 2 +3 + 1 +1 By substituting x = 1, in (1) 2 3 = 1 1 2+3 + 1+1 2+3 + 1+1 1 1 5 = 2 1 + 0+ 0 5 = 2 = 5 2 Similarly Putting x = 1, in (1) 2 3 = 1 1 2+3 + 1+1 2+3 + 1 1 1+1 1 = A 0 + 2 5 + 0 1 = 10 = 1 10 Similarly Putting x = 3 2 , in (1) 2 3 2 3 = 3 2 1 2 3 2 +3 + 3 2 +1 2 3 2 +3 + 3 2 1 3 2 +1 6 = 0+ 0+ 1 2 5 2 6 = 5 4 = 24 5 Therefore 2 3 + 1 1 2 + 3 = 5 2 + 1 + 1 10 1 + 24 5 2 + 3 Hence we can write it as 2 3 + 1 1 2 + 3 = 5 2 + 1 + 1 10 1 + 24 5 2 + 3 = 5 2 log +1 1 10 log 1 24 5 1 2 log 2 +3 + = + + +

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo