Ex 7.4, 18 - Chapter 7 Class 12 Integrals

Transcript

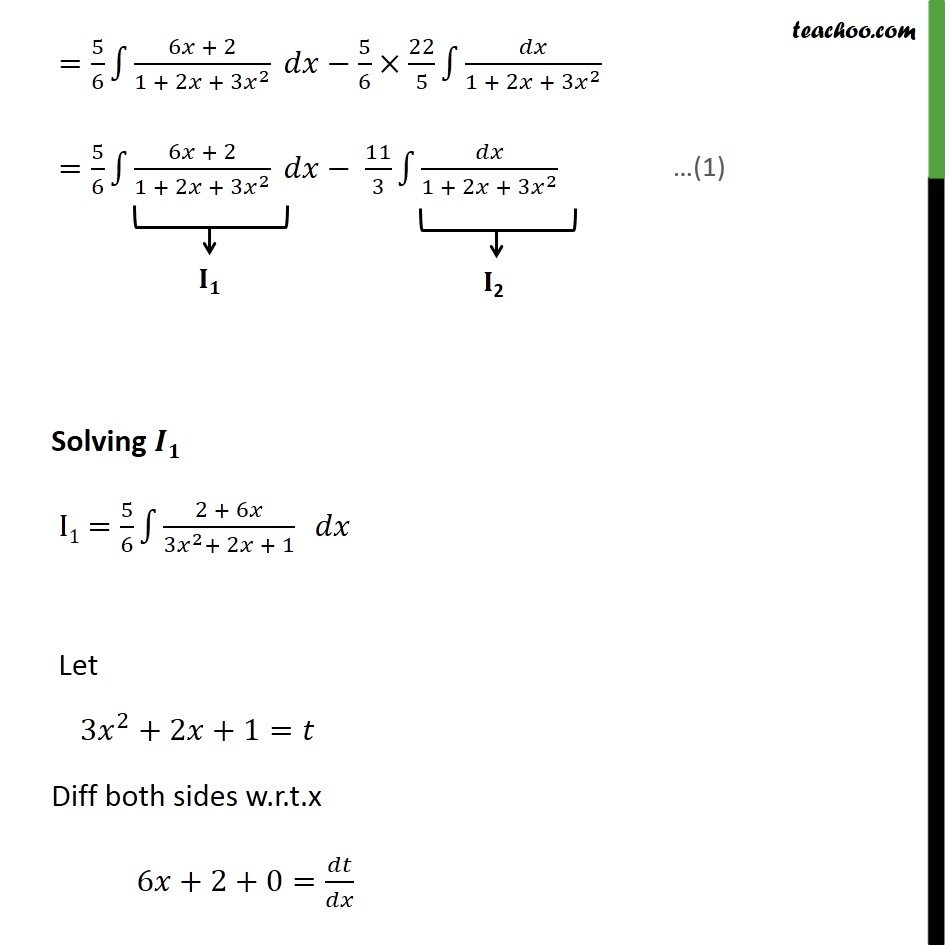
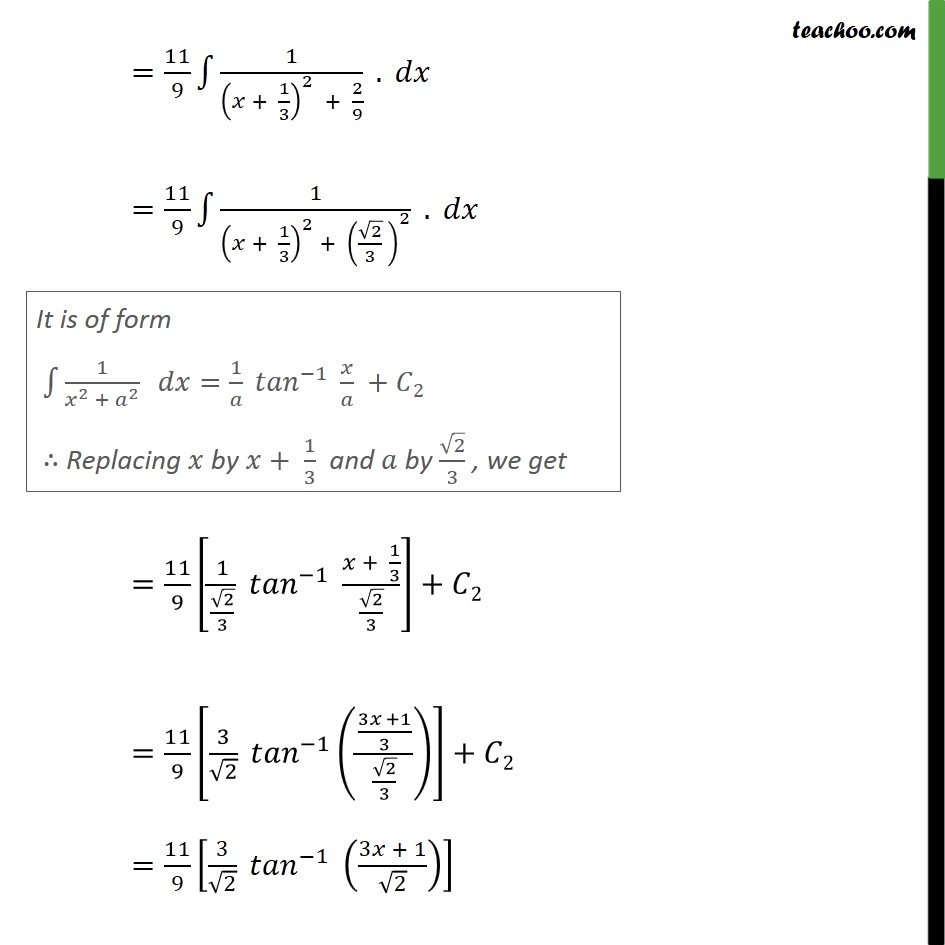
Ex 7.4, 18 5𝑥 − 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯ ﷮﷮ 5𝑥 − 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥 =5 ﷮﷮ 𝑥 − 2﷮5﷯﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥 = 5﷮6﷯ ﷮﷮ 6𝑥 − 12﷮5﷯﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥 = 5﷮6﷯ ﷮﷮ 6𝑥 + 2 − 12﷮5﷯ − 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥 = 5﷮6﷯ ﷮﷮ 6𝑥 + 2 − 22﷮5﷯﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥 = 5﷮6﷯ ﷮﷮ 6𝑥 + 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥− 5﷮6﷯× 22﷮5﷯ ﷮﷮ 𝑑𝑥﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ = 5﷮6﷯ ﷮﷮ 6𝑥 + 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ 𝑑𝑥− 11﷮3﷯ ﷮﷮ 𝑑𝑥﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ Solving 𝑰𝟏 I1 = 5﷮6﷯ ﷮﷮ 2 + 6𝑥﷮3 𝑥﷮2﷯+ 2𝑥 + 1﷯﷯ 𝑑𝑥 Let 3 𝑥﷮2﷯+2𝑥+1=𝑡 Diff both sides w.r.t.x 6𝑥+2+0= 𝑑𝑡﷮𝑑𝑥﷯ 𝑑𝑥= 𝑑𝑡﷮6𝑥 + 2﷯ Thus, our equation becomes I1= 5﷮6﷯ ﷮﷮ 2 + 6𝑥﷮3 𝑥﷮2﷯+ 2𝑥 + 1﷯﷯ 𝑑𝑥 Put the values of 3 𝑥﷮2﷯+2𝑥+1﷯ and 𝑑𝑥, we get I1= 5﷮6﷯ ﷮﷮ 6𝑥 + 2﷮𝑡﷯﷯ 𝑑𝑥 I1= 5﷮6﷯ ﷮﷮ 6𝑥 + 2﷮𝑡﷯﷯ × 𝑑𝑡﷮6𝑥 + 2﷯ I1= 5﷮6﷯ ﷮﷮ 1﷮𝑡﷯﷯ . 𝑑𝑡 I1= 5﷮6﷯ log﷮ 𝑡﷯﷯+𝐶1 I1= 5﷮6﷯ log﷮ 3 𝑥﷮2﷯+2𝑥+1﷯﷯+𝐶1 Solving 𝑰𝟐 I2= 11﷮3﷯ ﷮﷮ 1﷮3 𝑥﷮2﷯+ 2𝑥 + 1﷯﷯ . 𝑑𝑥 = 11﷮3﷯ ﷮﷮ 1﷮3 𝑥﷮2﷯+ 2𝑥﷮3﷯ + 1﷮3﷯﷯﷯﷯ . 𝑑𝑥 = 11﷮3 . 3﷯ ﷮﷮ 1﷮ 𝑥﷮2﷯+ 2𝑥﷮3﷯ + 1﷮3﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥﷮2﷯+ 2 𝑥﷯ 1﷮3﷯﷯ + 1﷮3﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥﷮2﷯+ 2 𝑥﷯ 1﷮3﷯﷯ + 1﷮3﷯﷯﷮2﷯− 1﷮3﷯﷯﷮2﷯+ 1﷮3﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥 + 1﷮3﷯﷯﷮2﷯− 1﷮3﷯﷯﷮2﷯+ 1﷮3﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥 + 1﷮3﷯﷯﷮2﷯− 1﷮9﷯ + 1﷮3﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥 + 1﷮3﷯﷯﷮2﷯ + 2﷮9﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ ﷮﷮ 1﷮ 𝑥 + 1﷮3﷯﷯﷮2﷯ + ﷮2﷯﷮3﷯ ﷯﷮2﷯﷯﷯ . 𝑑𝑥 = 11﷮9﷯ 1﷮ ﷮2﷯﷮3﷯﷯ 𝑡𝑎𝑛﷮−1﷯ ﷮ 𝑥 + 1﷮3﷯﷮ ﷮2﷯﷮3﷯﷯﷯﷯+𝐶2 = 11﷮9﷯ 3﷮ ﷮2﷯﷯ 𝑡𝑎𝑛﷮−1﷯﷮ 3𝑥 +1﷮3﷯﷮ ﷮2﷯﷮3﷯﷯﷯﷯﷯+𝐶2 = 11﷮9﷯ 3﷮ ﷮2﷯﷯ 𝑡𝑎𝑛﷮−1﷯ ﷮ 3𝑥 + 1﷮ ﷮2﷯﷯﷯﷯﷯ = 11﷮9﷯ . 3﷮ ﷮2﷯﷯ 𝑡𝑎𝑛﷮−1﷯ ﷮ 3𝑥 + 1﷮ ﷮2﷯﷯﷯﷯ + 11﷮9﷯ 𝐶2 = 11﷮3 ﷮2﷯﷯ 𝑡𝑎𝑛﷮−1﷯ ﷮ 3𝑥 + 1﷮ ﷮2﷯﷯﷯﷯ + 𝐶3 Now, putting the values of I1 and I2 in (1) ﷮﷮ 5𝑥 − 2﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ . 𝑑𝑥 = 5﷮6﷯ ﷮﷮ 2 + 6𝑥﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ . 𝑑𝑥− 11﷮3﷯ ﷮﷮ 1﷮1 + 2𝑥 + 3 𝑥﷮2﷯﷯﷯ . 𝑑𝑥 = 𝟓﷮𝟔﷯ 𝒍𝒐𝒈﷮ 𝟑 𝒙﷮𝟐﷯+𝟐𝒙+𝟏﷯﷯− 𝟏𝟏﷮𝟑 ﷮𝟐﷯﷯ 𝒕𝒂𝒏﷮−𝟏﷯ ﷮ 𝟑𝒙 + 𝟏﷮ ﷮𝟐﷯﷯﷯﷯ + 𝑪