Ex 7.7, 11 - Integrate root x2 - 8x + 7 dx - Class 12

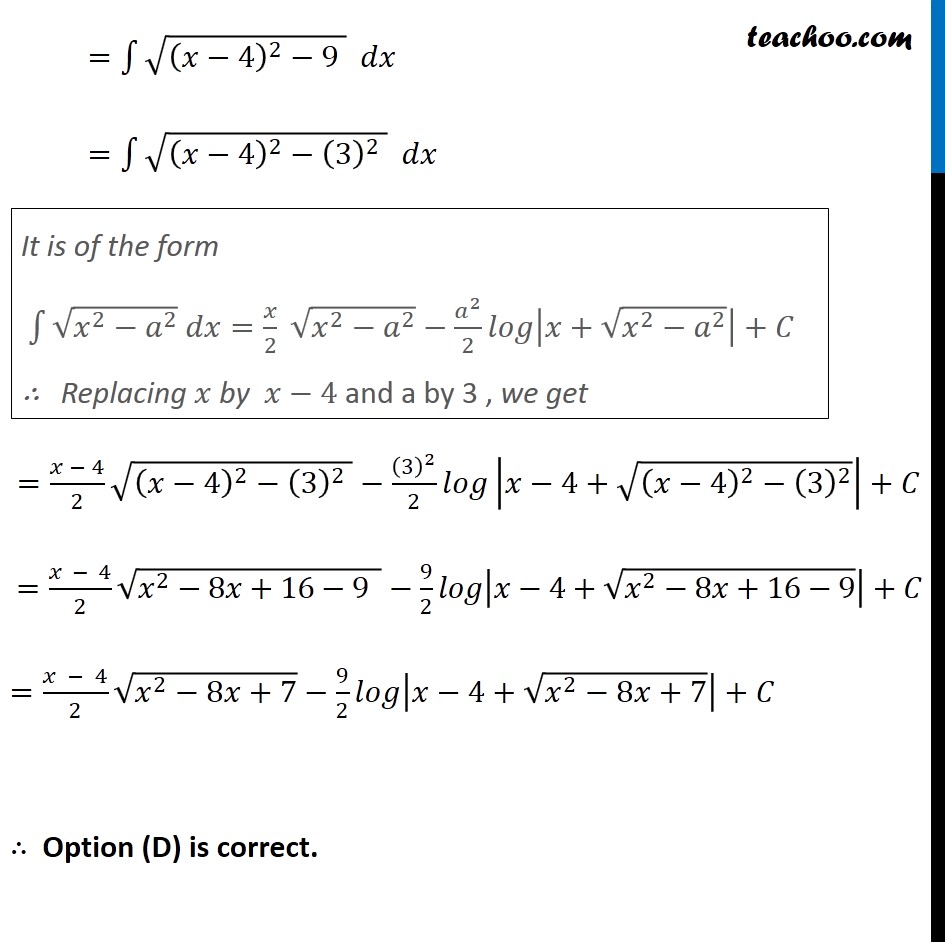
Ex 7.7, 11 ∫1▒〖√(𝑥2 −8𝑥+7) " " 〗 𝑑𝑥 1/2 (x - 4)√(𝑥2 −8𝑥+7) + 9 log |𝑥−4+√(𝑥2−8𝑥+7)| + C 1/2 (x + 4)√(𝑥2 −8𝑥+7) + 9 log |𝑥+4+√(𝑥2−8𝑥+7)| + C 1/2 (x - 4)√(𝑥2 −8𝑥+7) - 3√2 log |𝑥−4+√(𝑥2−8𝑥+7)| + C 1/2 (x - 4)√(𝑥2 −8𝑥+7) + 9/2 log |𝑥−4+√(𝑥2−8𝑥+7)| + C ∫1▒〖√(𝑥^2−8𝑥+7) 𝑑𝑥〗 =∫1▒〖√(𝑥^2−2(4)(𝑥)+7) 𝑑𝑥〗 =∫1▒〖√(𝑥^2−2(4)(𝑥) 〖+(4)〗^2−(4)^2+7) 𝑑𝑥〗 =∫1▒〖√((𝑥−4)^2−16+7) 𝑑𝑥〗 =∫1▒〖√((𝑥−4)^2−9 ) 𝑑𝑥〗 =∫1▒〖√((𝑥−4)^2−(3)^2 ) 𝑑𝑥〗 =(𝑥 − 4)/2 √((𝑥−4)^2−(3)^2 )−(3)^2/2 𝑙𝑜𝑔|𝑥−4+√((𝑥−4)^2−(3)^2 )|+𝐶 =(𝑥 − 4)/2 √(𝑥^2−8𝑥+16−9 )−9/2 𝑙𝑜𝑔|𝑥−4+√(𝑥^2−8𝑥+16−9)|+𝐶 =(𝑥 − 4)/2 √(𝑥^2−8𝑥+7)−9/2 𝑙𝑜𝑔|𝑥−4+√(𝑥^2−8𝑥+7)|+𝐶 ∴ Option (D) is correct. It is of the form ∫1▒〖√(𝑥^2−𝑎^2 ) 𝑑𝑥=𝑥/2 √(𝑥^2−𝑎^2 )−𝑎^2/2 𝑙𝑜𝑔|𝑥+√(𝑥^2−𝑎^2 )|+𝐶〗 ∴ Replacing 𝑥 by 𝑥−4 and a by 3 , we get

Ex 7.7, 11 - Chapter 7 Class 12 Integrals (Important Question)

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular