
Definite Integration - By Formulae
Definite Integration - By Formulae
Last updated at Dec. 16, 2024 by Teachoo


Transcript
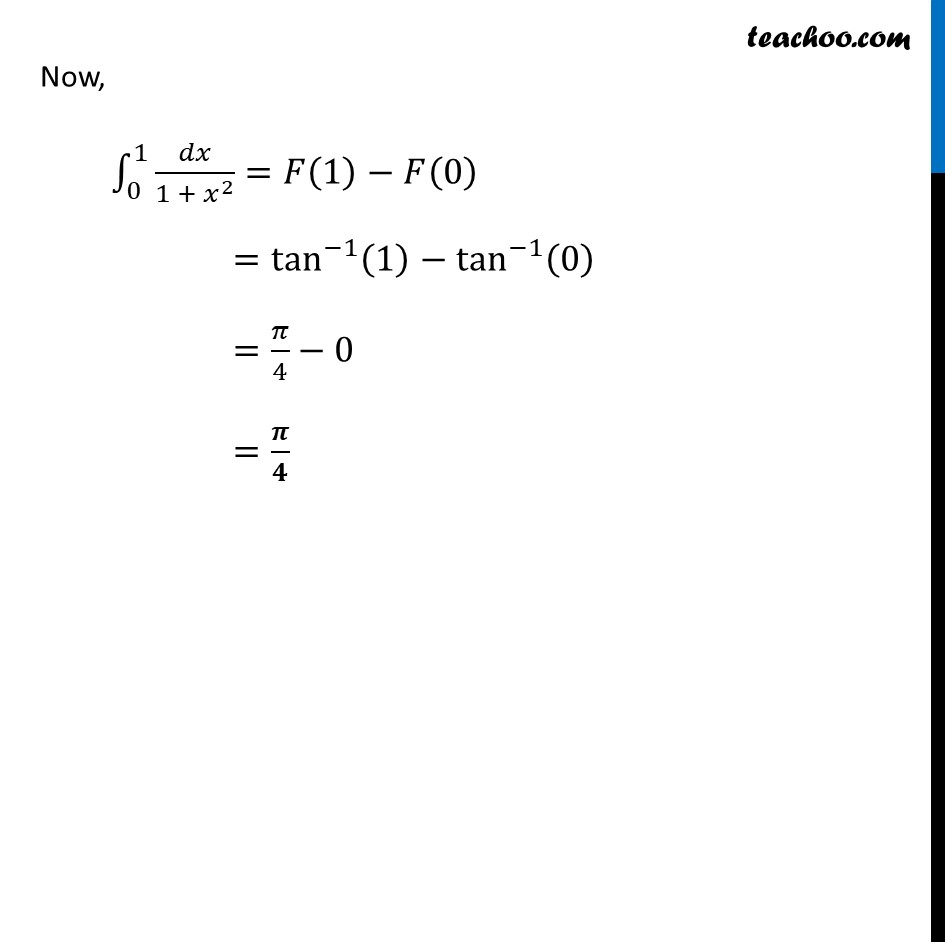
Ex 7.8, 10 ∫_0^1▒𝑑𝑥/(1 + 𝑥2) Let F(𝑥)=∫1▒𝑑𝑥/(1 + 𝑥^2 ) =∫1▒1/(1^2 + 𝑥^2 ) 𝑑𝑥 =1/1 .tan^(−1)(𝑥/1) =tan^(−1) 𝑥 Hence F(𝑥)=tan^(−1) 𝑥 Now, ∫_0^1▒〖𝑑𝑥/(1 + 𝑥^2 )=𝐹(1)−𝐹(0) 〗 =tan^(−1)〖(1)−tan^(−1)(0) 〗 =𝜋/4−0 =𝝅/𝟒