If f(x) = Integration t sin t from 0 to x, then f'(x) is [MCQ]

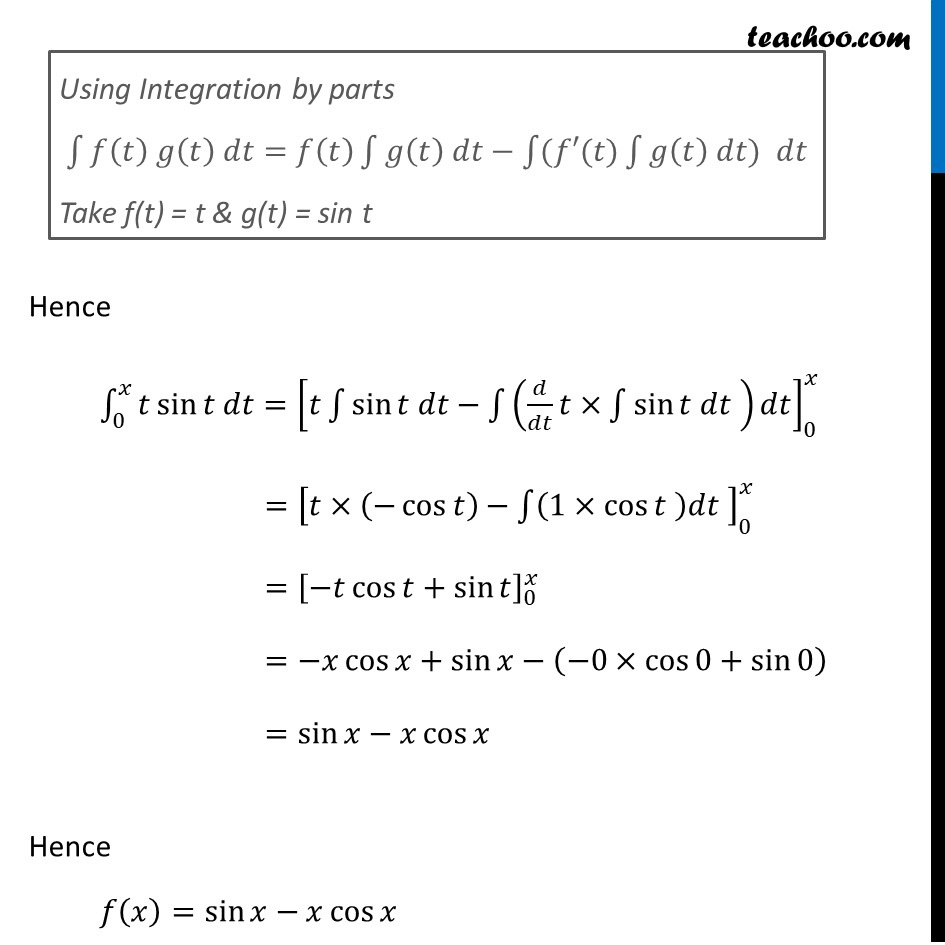
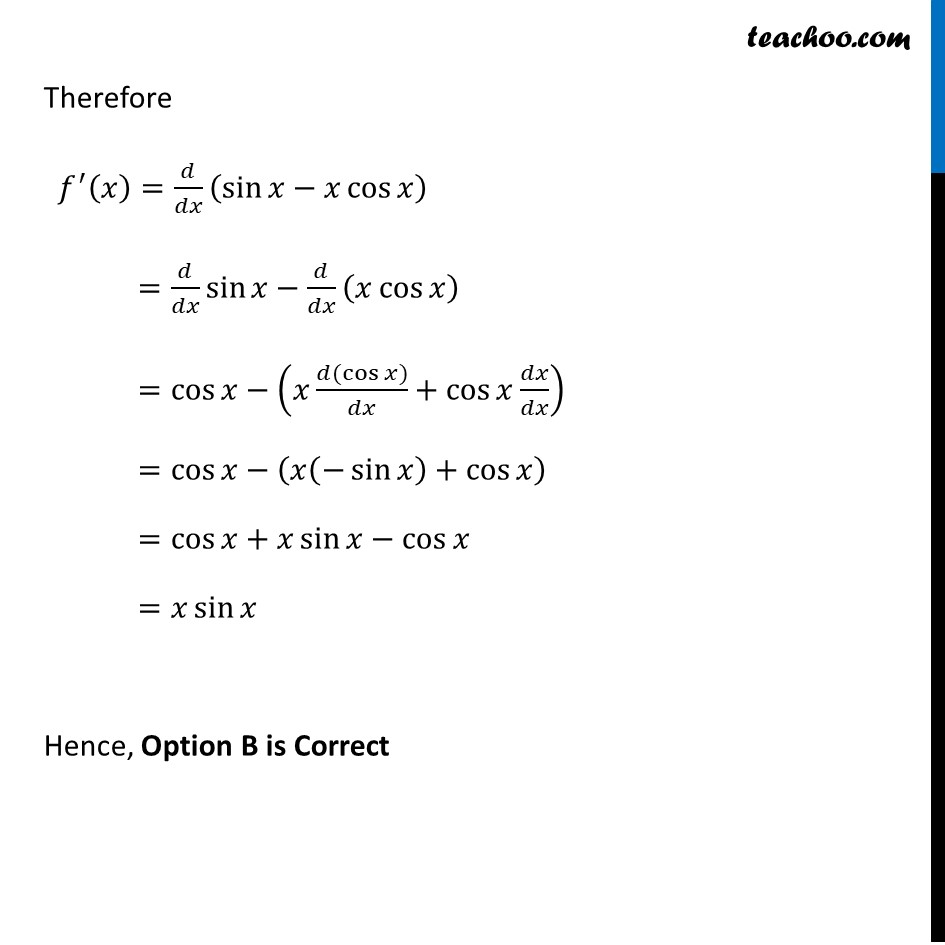
Ex 7.9, 10 If 𝑓(𝑥)=∫_0^𝑥▒〖𝑡 sin⁡𝑡 𝑑𝑡〗, then 𝑓′(𝑥) is (A) cos 𝑥+𝑥 𝑠𝑖𝑛 𝑥 (B) 𝑥 𝑠𝑖𝑛 𝑥 (C) 𝑥 cos 𝑥 (D) sin⁡𝑥 𝑥 cos⁡𝑥 If 𝑓(𝑥)=∫_0^𝑥▒〖𝑡 sin⁡〖𝑡 𝑑𝑡〗〗 Integrating by parts 𝑓(𝑥)=∫_0^𝑥▒〖𝑡 sin⁡〖𝑡 𝑑𝑡〗〗 By ILATE rule , 𝑡 is first function and sin 𝑡 is second function Hence ∫_0^𝑥▒〖𝑡 sin⁡〖𝑡 𝑑𝑡=[𝑡∫1▒sin⁡〖𝑡 𝑑𝑡−∫1▒(𝑑/𝑑𝑡 𝑡×∫1▒sin⁡〖𝑡 𝑑𝑡〗 )𝑑𝑡〗 ]_0^𝑥 〗〗 =[𝑡×(−cos⁡𝑡 )−∫1▒(1×cos⁡𝑡 )𝑑𝑡 ]_0^𝑥 =[−𝑡 cos⁡〖𝑡+sin⁡𝑡 〗 ]_0^𝑥 =−𝑥 cos⁡〖𝑥+sin⁡〖𝑥−(−0×cos⁡〖0+sin⁡0 〗 )〗〗 =sin⁡𝑥−𝑥 cos⁡𝑥 Hence 𝑓(𝑥)=sin⁡〖𝑥−𝑥 cos⁡𝑥 〗 Therefore 𝑓^′ (𝑥)=𝑑/𝑑𝑥 (sin⁡〖𝑥−𝑥 cos⁡𝑥 〗 ) =𝑑/𝑑𝑥 sin⁡〖𝑥−𝑑/𝑑𝑥 (𝑥 cos⁡𝑥 )〗 =cos⁡〖𝑥−(𝑥 (𝑑(cos⁡𝑥))/𝑑𝑥+cos⁡〖𝑥 𝑑𝑥/𝑑𝑥〗 )〗 =cos⁡〖𝑥−(𝑥(−sin⁡𝑥 )+cos⁡𝑥 )〗 =cos⁡〖𝑥+𝑥 sin⁡〖𝑥−cos⁡𝑥 〗〗 =𝑥 sin⁡𝑥 Hence, Option B is Correct

Ex 7.9, 10 (MCQ) - Chapter 7 Class 12 Integrals

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular