Misc 39 (MCQ) - Chapter 7 Class 12 Integrals

Transcript

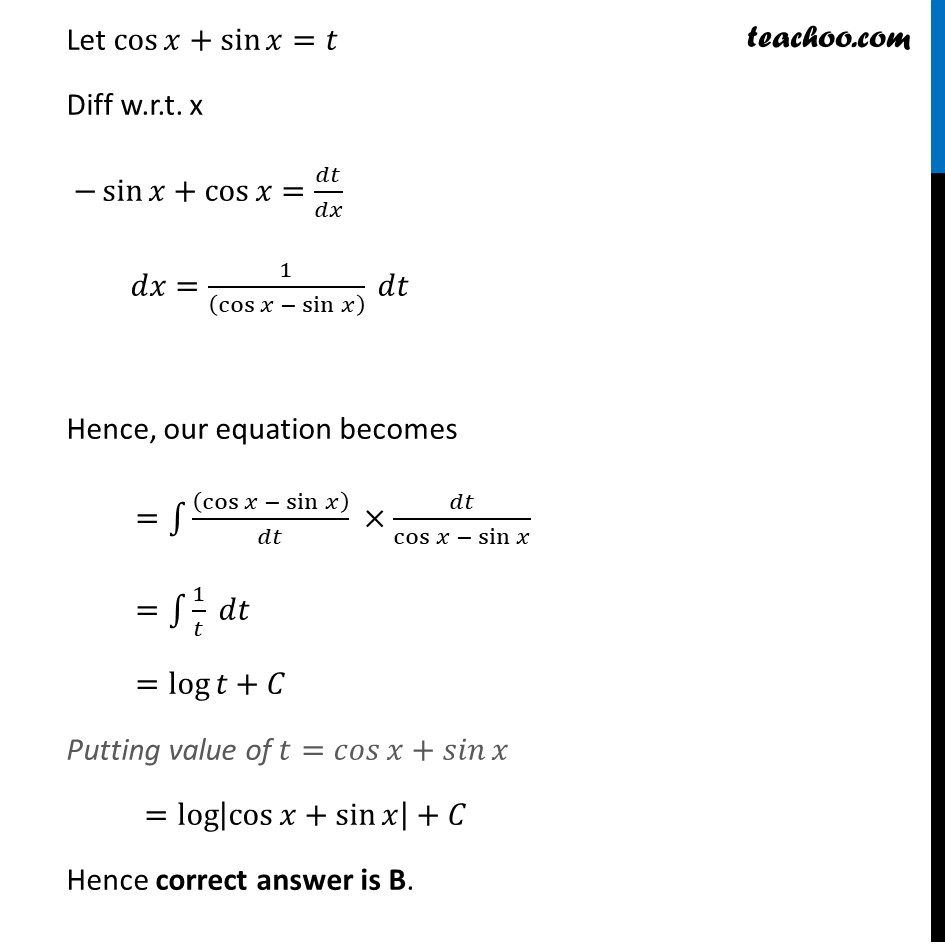
Misc 39 Choose the correct answer ∫▒cos⁡2𝑥/((sin⁡𝑥 + cos⁡𝑥 )^2 ) 𝑑𝑥 is equal to (A) (−1)/(sin⁡𝑥 + cos⁡𝑥 )+𝐶 (B) log⁡|sin⁡𝑥+cos⁡𝑥 |+𝐶 (C) log⁡|sin⁡𝑥−cos⁡𝑥 |+𝐶 (D) " " 1/(sin⁡𝑥 + cos⁡𝑥 )^2 ∫1▒cos⁡2𝑥/(cos⁡𝑥 + sin⁡𝑥 )^2 =∫1▒(cos^2⁡𝑥 − sin^2⁡𝑥)/(cos⁡𝑥 + sin⁡𝑥 )^2 𝑑𝑥 =∫1▒(cos⁡𝑥 − sin⁡𝑥 )(cos⁡𝑥 + sin⁡𝑥 )/(cos⁡𝑥 + sin⁡𝑥 )^2 𝑑𝑥 =∫1▒(cos⁡𝑥 − sin⁡𝑥)/(cos⁡𝑥 + sin⁡𝑥 ) 𝑑𝑥 Let cos⁡𝑥+sin⁡𝑥=𝑡 Diff w.r.t. x −sin⁡𝑥+cos⁡𝑥=𝑑𝑡/𝑑𝑥 𝑑𝑥=1/((cos⁡𝑥 − sin⁡𝑥 ) ) 𝑑𝑡 Hence, our equation becomes =∫1▒((cos⁡𝑥 − sin⁡𝑥 ))/𝑑𝑡 ×𝑑𝑡/(cos⁡𝑥 − sin⁡𝑥 ) =∫1▒1/𝑡 𝑑𝑡 =log⁡𝑡+𝐶 Putting value of 𝑡=𝑐𝑜𝑠⁡𝑥+𝑠𝑖𝑛⁡𝑥 =log⁡|cos⁡𝑥+sin⁡𝑥 |+𝐶 Hence correct answer is B.