Misc 35 - Chapter 7 Class 12 Integrals

Last updated at Dec. 16, 2024 by Teachoo

Next: Misc 36 Important → Share on WhatsApp

Transcript

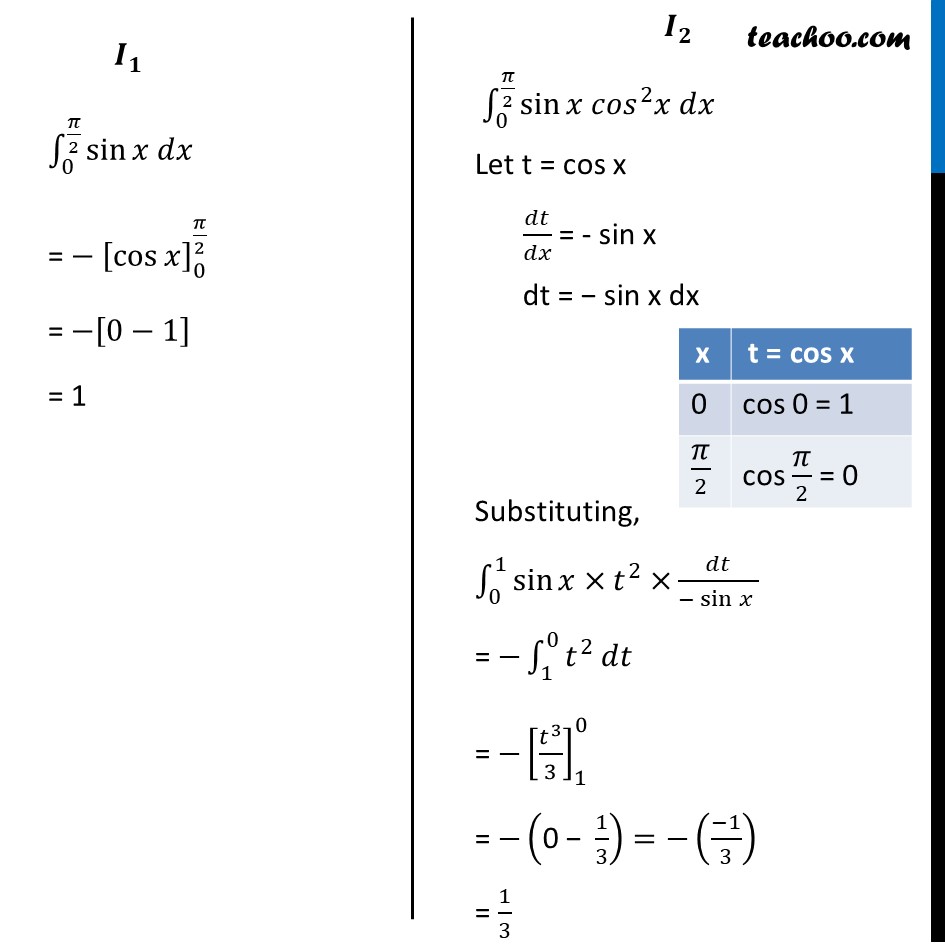
Misc 35 Prove that ∫_0^(𝜋/2)▒sin^3⁡𝑥 𝑑𝑥=2/3 Solving L.H.S ∫_0^(𝜋/2)▒sin^3⁡𝑥 𝑑𝑥 = ∫_0^(𝜋/2)▒〖〖sin 𝑥 (sin〗^2⁡〖𝑥)〗〗 𝑑𝑥 = ∫_0^(𝜋/2)▒𝑠𝑖𝑛⁡〖𝑥 (1−〖𝑐𝑜𝑠〗^2 𝑥)〗 𝑑𝑥 = ∫_0^(𝜋/2)▒𝑠𝑖𝑛⁡〖𝑥 𝑑𝑥− ∫1_0^(𝜋/2)▒〖sin⁡〖𝑥 〖𝑐𝑜𝑠〗^2 𝑥〗 𝑑𝑥〗〗 𝑰_𝟏 ∫1_0^(𝜋/2)▒sin⁡〖𝑥 𝑑𝑥〗 = − [cos⁡𝑥 ]_0^(𝜋/2) = −[0−1] = 1 𝑰_𝟐 ∫1_0^(𝜋/2)▒sin⁡〖𝑥 〖𝑐𝑜𝑠〗^2 𝑥 𝑑𝑥〗 Let t = cos x 𝑑𝑡/𝑑𝑥 = - sin x dt = − sin x dx Substituting, ∫1_0^1▒sin⁡〖𝑥×𝑡^2×〗 𝑑𝑡/(−sin⁡〖𝑥 〗 ) = −∫1_1^0▒〖𝑡^2 𝑑𝑡〗 = 〖−[𝑡^3/3]〗_1^0 = −("0 − " 1/3)=−((−1)/3) = 1/3 L.H.S = 𝐼_1− 𝐼_2 = 1 − 1/3 = 𝟐/𝟑 = R.H.S Hence, proved.

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular