Misc 32 - Chapter 7 Class 12 Integrals

Transcript

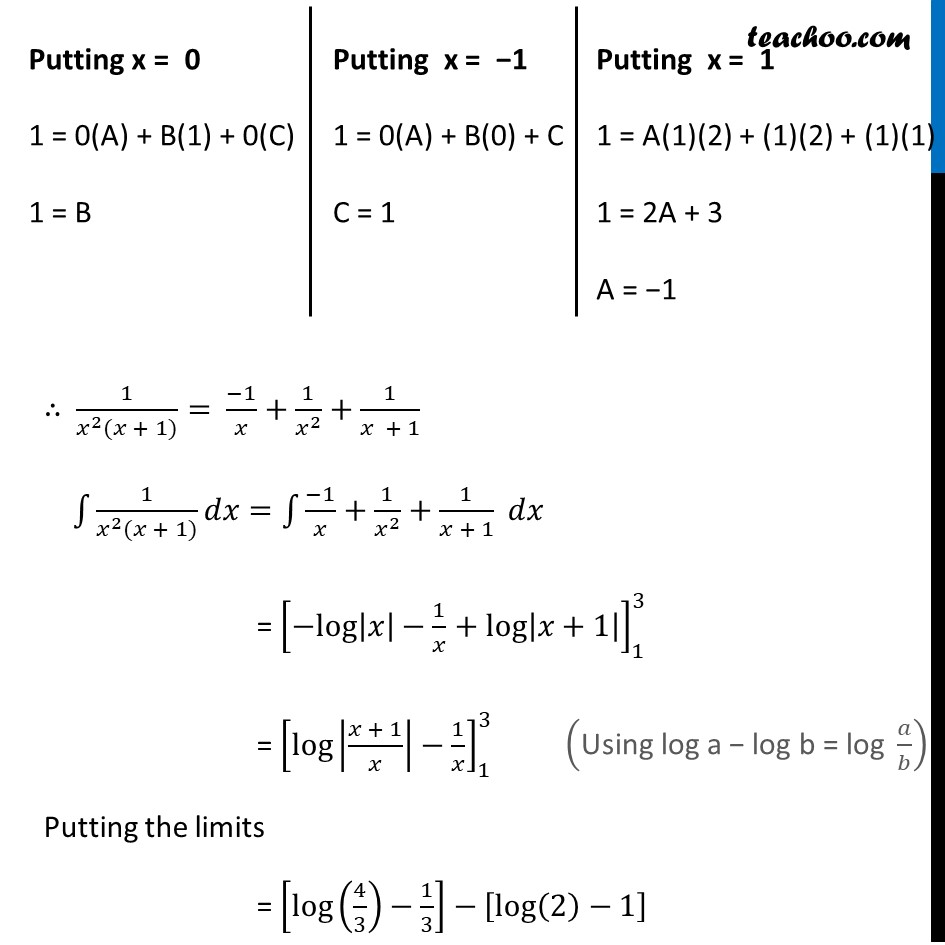
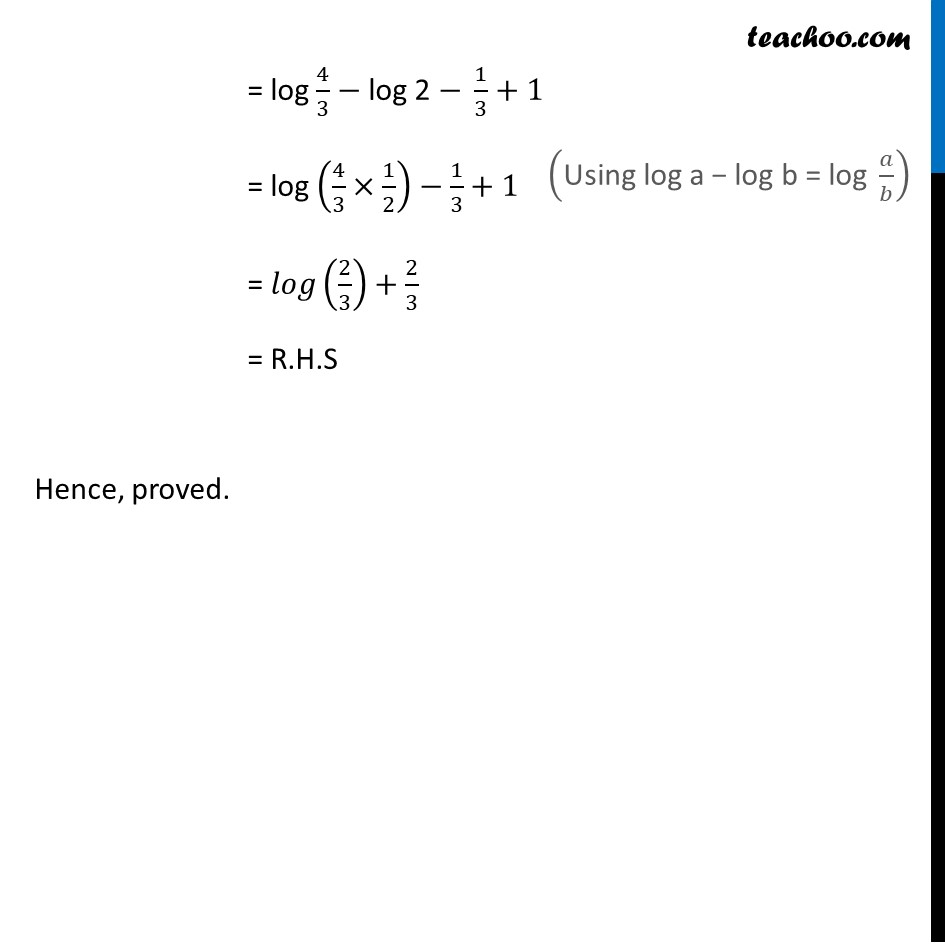
Misc 32 Prove that ∫_1^3▒〖𝑑𝑥/(𝑥^2 (𝑥 + 1) )= 2/3〗+log⁡〖2/3〗 Solving L.H.S : ∫_1^3▒𝑑𝑥/(𝑥^2 (𝑥 + 1) ) By partial fraction, 1/(𝑥^2 (𝑥 + 1)) = A/𝑥+B/𝑥^2 +C/(𝑥 + 1) 1/(𝑥^2 (𝑥 + 1)) = ( A 𝑥 (𝑥 + 1) + B (𝑥 + 1) + C𝑥^2)/(𝑥^2 (𝑥 + 1)) ∴ 1 = Ax (x + 1) + B (x + 1) + C𝑥^2 Finding A,B,C ∴ 1/(𝑥^2 (𝑥 + 1))= (−1)/𝑥+1/𝑥^2 +1/(𝑥 + 1) ∫1▒1/(𝑥^2 (𝑥 + 1)) 𝑑𝑥=∫1▒(−1)/𝑥+1/𝑥^2 +1/(𝑥 + 1) 𝑑𝑥 = [−log|𝑥|−1/𝑥+log|𝑥+1|]_1^3 = [log|(𝑥 + 1)/𝑥|−1/𝑥]_1^3 Putting the limits = [log(4/3)−1/3]−[log(2)−1] = "log" 4/3−"log" 2 − 1/3+1 = "log" (4/3×1/2)−1/3+1 = 𝑙𝑜𝑔(2/3)+2/3 = R.H.S Hence, proved.