You are here
Misc 13 - Chapter 7 Class 12 Integrals
Last updated at December 16, 2024 by Teachoo
Integration by partial fraction - Type 1
Chapter 7 Class 12 Integrals
Concept wise
- Using Formulaes
- Using Trignometric Formulaes
- Integration by substitution - x^n
- Integration by substitution - lnx
- Integration by substitution - e^x
- Integration by substitution - Trignometric - Normal
- Integration by substitution - Trignometric - Inverse
- Integration using trigo identities - sin^2,cos^2 etc formulae
- Integration using trigo identities - a-b formulae
- Integration using trigo identities - 2x formulae
- Integration using trigo identities - 3x formulae
- Integration using trigo identities - CD and CD inv formulae
- Integration using trigo identities - Inv Trigo formulae
- Integration by parts
- Integration by parts - e^x integration
- Integration by specific formulaes - Formula 1
- Integration by specific formulaes - Formula 2
- Integration by specific formulaes - Formula 3
- Integration by specific formulaes - Formula 4
- Integration by specific formulaes - Formula 5
- Integration by specific formulaes - Formula 6
- Integration by specific formulaes - Formula 7
- Integration by specific formulaes - Formula 8
- Integration by specific formulaes - Method 9
- Integration by specific formulaes - Method 10
-
Integration by partial fraction - Type 1
- Integration by partial fraction - Type 2
- Integration by partial fraction - Type 3
- Integration by partial fraction - Type 4
- Integration by partial fraction - Type 5
- Definite Integral as a limit of a sum
- Definite Integration - By Formulae
- Definite Integration - By Partial Fraction
- Definite Integration - By e formula
- Definite Integration - By Substitution
- Definite Integration by properties - P2
- Definite Integration by properties - P3
- Definite Integration by properties - P4
- Definite Integration by properties - P6
- Definite Integration by properties - P7
Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 5 questions, selected from your answers, mistakes, and progress.
Transcript
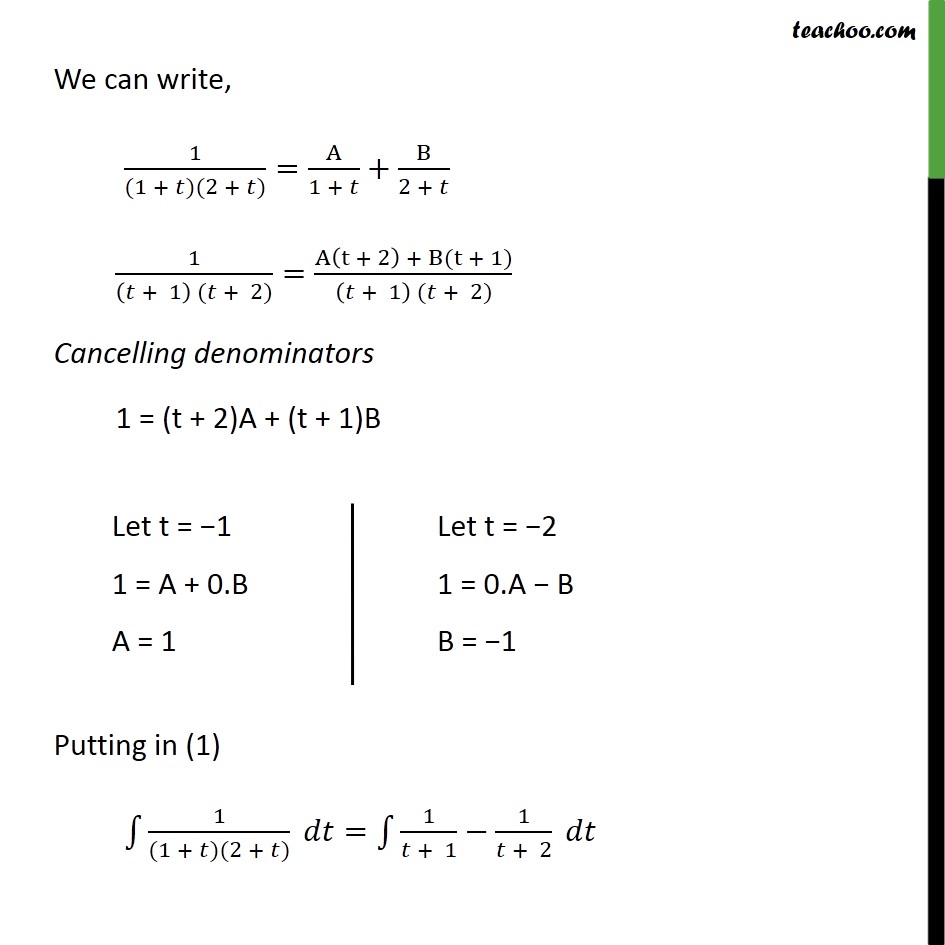
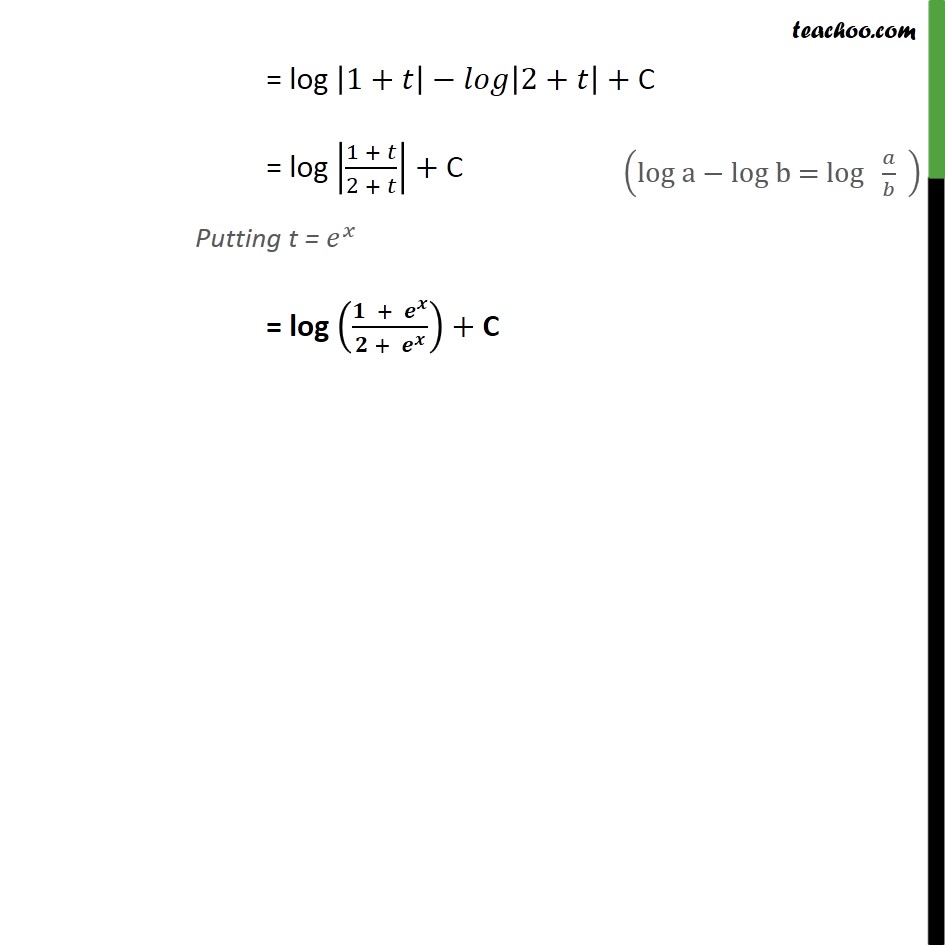
Misc 13 Integrate the function ^ /((1 + ^ )(2+ ^ ) ) 1 ^ /((1 + ^ )(2+ ^ ) ) Let t = ^ / = ^ dt = ^ Substituting 1 ^ /((1 + ^ )(2+ ^ ) ) = 1 /((1 + ) (2 + )) We can write, 1/((1 + )(2 + ))=A/(1 + )+B/(2 + ) 1/(( + 1) ( + 2))=(A(t + 2) + B(t + 1))/(( + 1) ( + 2)) Cancelling denominators 1 = (t + 2)A + (t + 1)B Putting in (1) 1 1/((1 + )(2 + )) = 1 1/( + 1) 1/( + 2) = log |1+ | |2+ |+ C = log |(1 + )/(2 + )|+ C Putting t = ^ = log (( + ^ )/( + ^ ))+ C
