Example 39 - Evaluate integral [root cot x + root tan x] dx

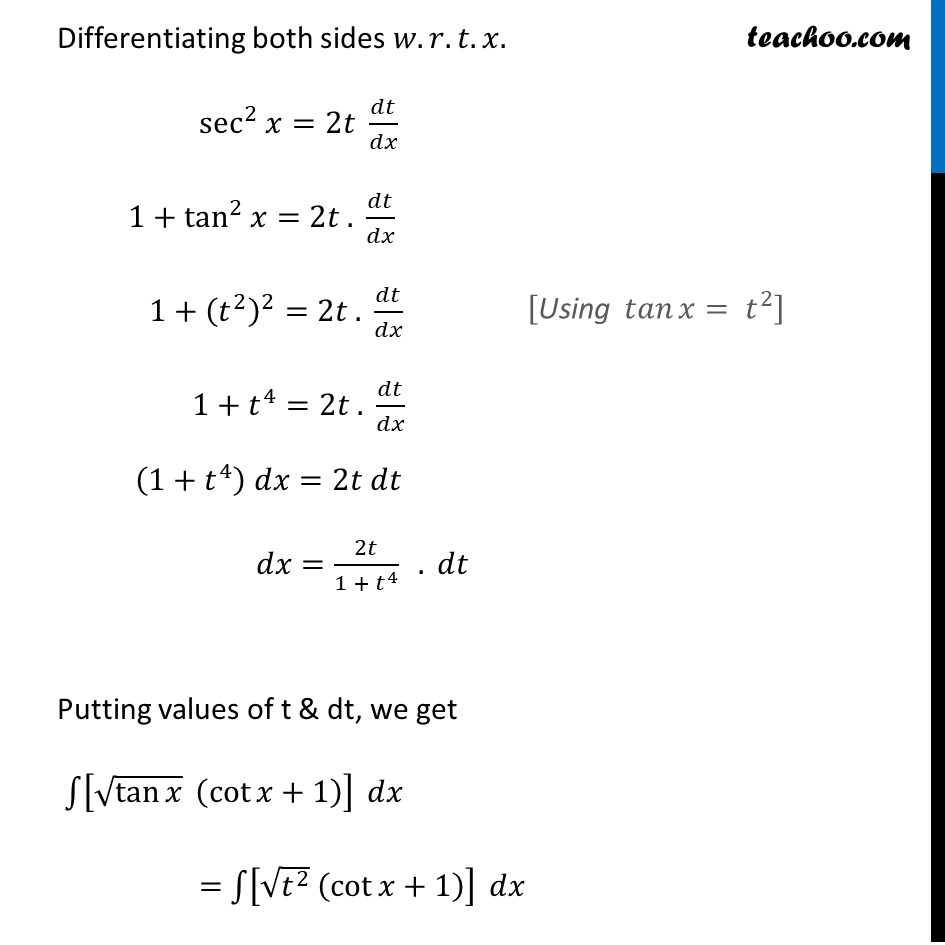
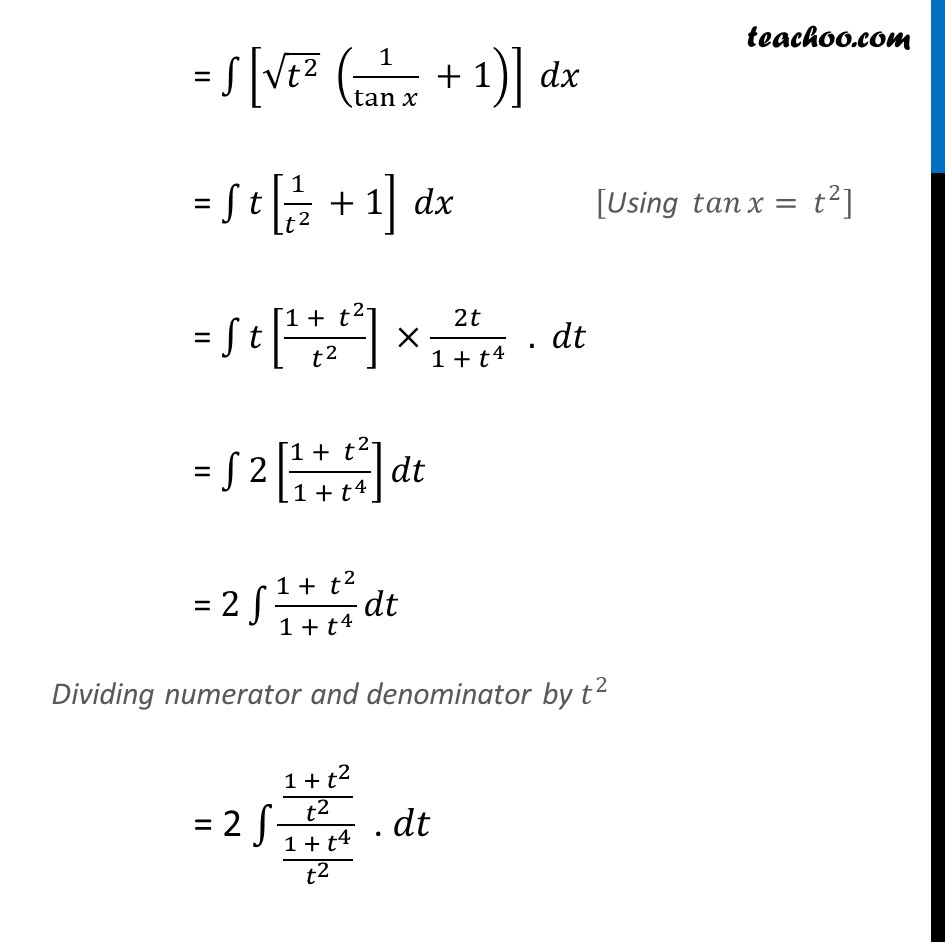
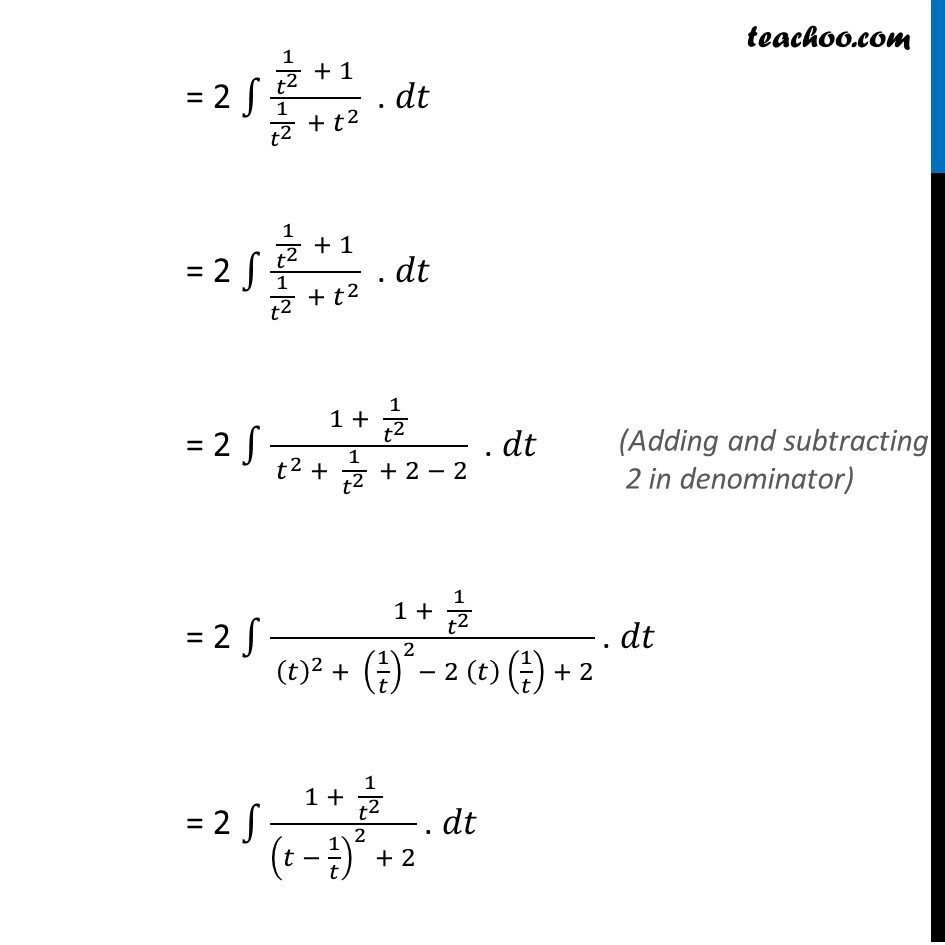
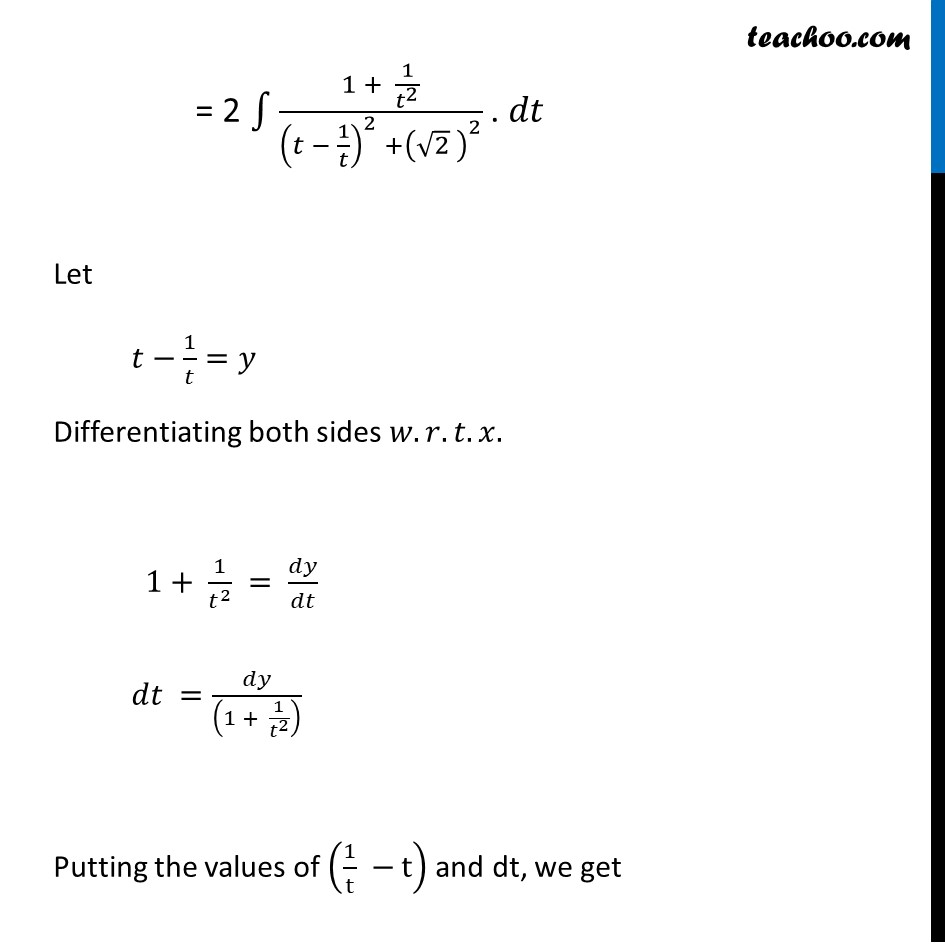
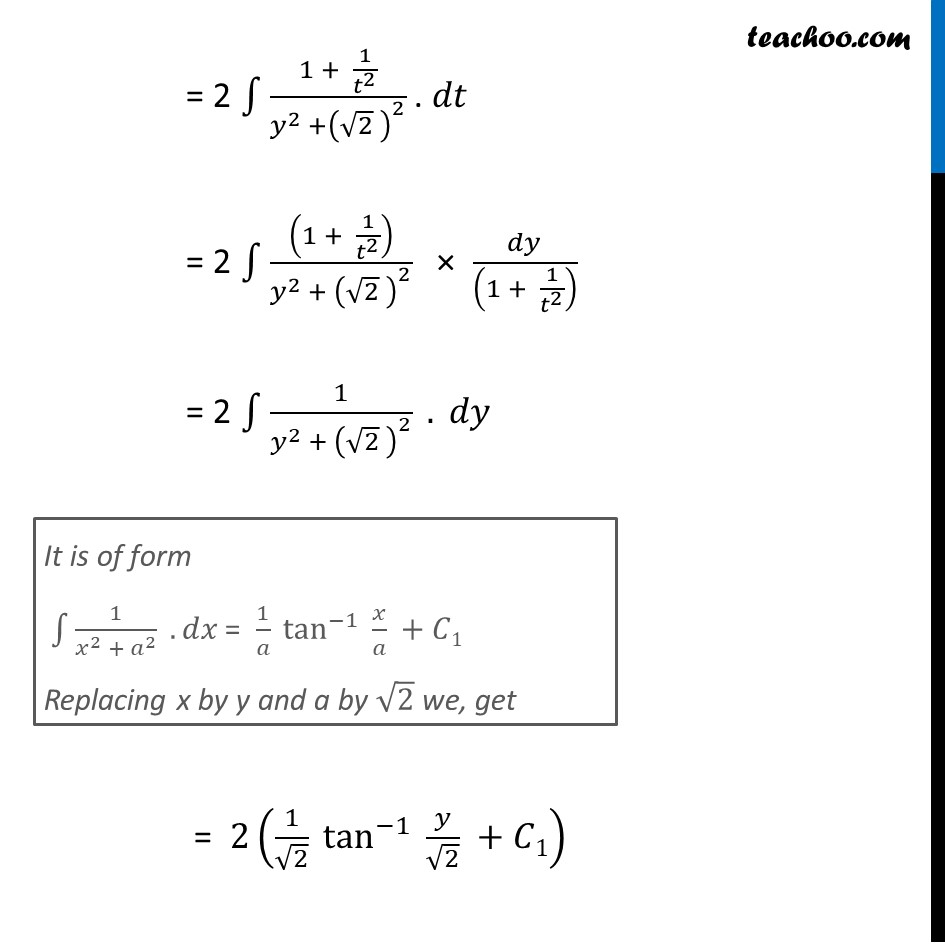
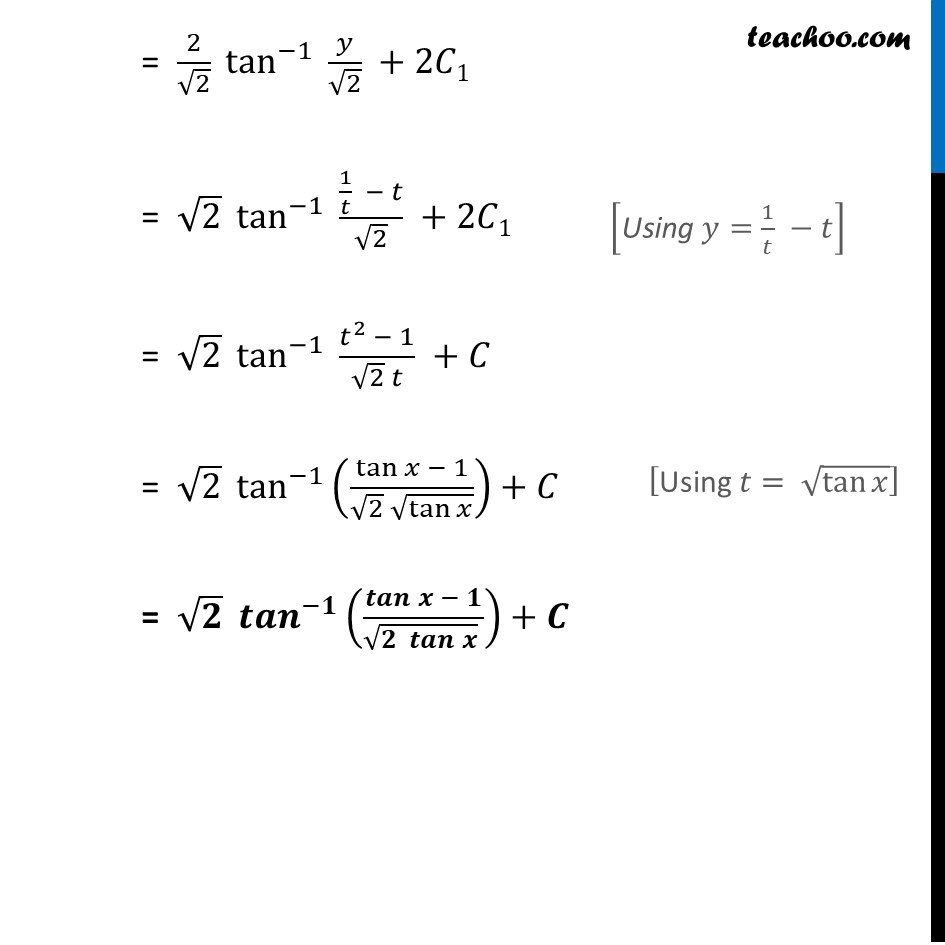
Example 39 Evaluate ∫1▒[√(cot⁡𝑥 )+√(tan⁡𝑥 )] 𝑑𝑥 ∫1▒[√(cot⁡𝑥 )+√(tan⁡𝑥 )] 𝑑𝑥 =∫1▒[√(cot⁡𝑥 )+1/√(cot⁡𝑥 )] 𝑑𝑥 =∫1▒[(cot⁡𝑥 + 1)/√(cot⁡𝑥 )] 𝑑𝑥 =∫1▒[√(tan⁡𝑥 ) (cot⁡𝑥+1)] 𝑑𝑥 Let tan⁡𝑥=𝑡^2 Differentiating both sides 𝑤.𝑟.𝑡.𝑥. sec^2 𝑥=2𝑡 𝑑𝑡/𝑑𝑥 1+tan^2 𝑥=2𝑡 . 𝑑𝑡/𝑑𝑥 1+(𝑡^2 )^2=2𝑡 . 𝑑𝑡/𝑑𝑥 1+𝑡^4=2𝑡 . 𝑑𝑡/𝑑𝑥 (1+𝑡^4 ) 𝑑𝑥=2𝑡 𝑑𝑡 𝑑𝑥=2𝑡/(1 + 𝑡^4 ) . 𝑑𝑡 Putting values of t & dt, we get ∫1▒[√(tan⁡𝑥 ) (cot⁡𝑥+1)] 𝑑𝑥 = ∫1▒[√(𝑡^2 ) (cot⁡𝑥+1)] 𝑑𝑥 = ∫1▒[√(𝑡^2 ) (1/tan⁡𝑥 +1)] 𝑑𝑥 = ∫1▒𝑡[1/𝑡^2 +1] 𝑑𝑥 = ∫1▒𝑡[(1 + 𝑡^2)/𝑡^2 ] ×2𝑡/(1 + 𝑡^4 ) . 𝑑𝑡 = ∫1▒2[(1 + 𝑡^2)/(1 + 𝑡^4 )] 𝑑𝑡 = 2∫1▒(1 + 𝑡^2)/(1 + 𝑡^4 ) 𝑑𝑡 Dividing numerator and denominator by 𝑡^2 = 2 ∫1▒((1 + 𝑡^2)/𝑡^2 )/((1 + 𝑡^4)/𝑡^2 ) . 𝑑𝑡 = 2 ∫1▒(1/𝑡^2 + 1)/(1/𝑡^2 + 𝑡^2 ) . 𝑑𝑡 = 2 ∫1▒(1/𝑡^2 + 1)/(1/𝑡^2 + 𝑡^2 ) . 𝑑𝑡 = 2 ∫1▒(1 + 1/𝑡^2 )/( 𝑡^2 + 1/𝑡^2 + 2 − 2) . 𝑑𝑡 = 2 ∫1▒(1 + 1/𝑡^2 )/( (𝑡)^2 + (1/𝑡)^2− 2 (𝑡) (1/𝑡) + 2) . 𝑑𝑡 = 2 ∫1▒(1 + 1/𝑡^2 )/((𝑡 − 1/𝑡)^2 + 2) . 𝑑𝑡 = 2 ∫1▒(1 + 1/𝑡^2 )/((𝑡 − 1/𝑡)^2 +(√2 )^2 ) . 𝑑𝑡 Let 𝑡−1/𝑡=𝑦 Differentiating both sides 𝑤.𝑟.𝑡.𝑥. 1+ 1/𝑡^2 = 𝑑𝑦/𝑑𝑡 𝑑𝑡 =𝑑𝑦/((1 + 1/𝑡^2 ) ) Putting the values of (1/t −t) and dt, we get = 2 ∫1▒(1 + 1/𝑡^2 )/(𝑦^2 +(√2 )^2 ) . 𝑑𝑡 = 2 ∫1▒((1 + 1/𝑡^2 ))/(𝑦^2 + (√2 )^2 ) × 𝑑𝑦/((1 + 1/𝑡^2 ) ) = 2 ∫1▒1/(𝑦^2 + (√2 )^2 ) . 𝑑𝑦 = 2(1/√2 tan^(−1)⁡〖 𝑦/√2〗 +𝐶1) = 2/√2 tan^(−1)⁡〖 𝑦/√2〗 +2𝐶1 = √2 tan^(−1)⁡〖 (1/𝑡 − 𝑡)/√2〗 +2𝐶1 = √2 tan^(−1)⁡〖 (𝑡^2 − 1)/(√2 𝑡)〗 +𝐶 = √2 tan^(−1)⁡((tan⁡𝑥 − 1)/(√2 √(tan⁡𝑥 )))+𝐶 = √𝟐 〖𝒕𝒂𝒏〗^(−𝟏)⁡((𝒕𝒂𝒏⁡𝒙 − 𝟏)/(√(𝟐 𝒕𝒂𝒏⁡𝒙 ) ))+𝑪

Example 39 - Chapter 7 Class 12 Integrals (Important Question)

Transcript

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular