Example 39

Transcript

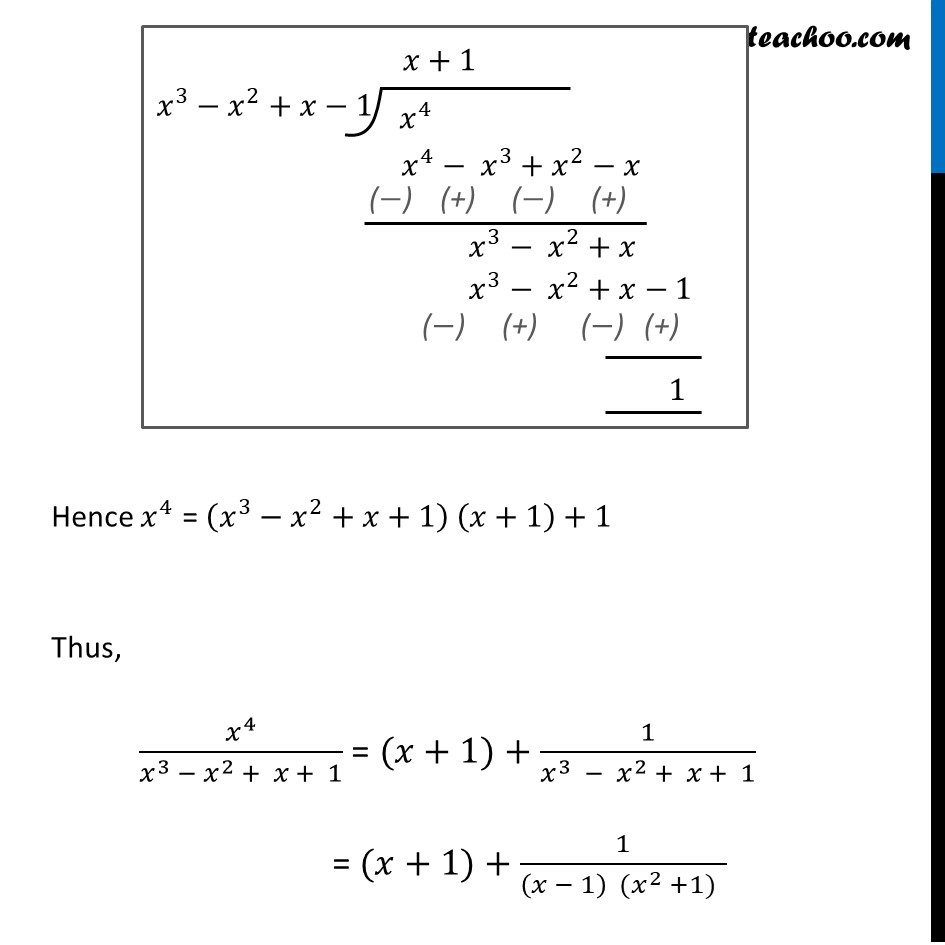
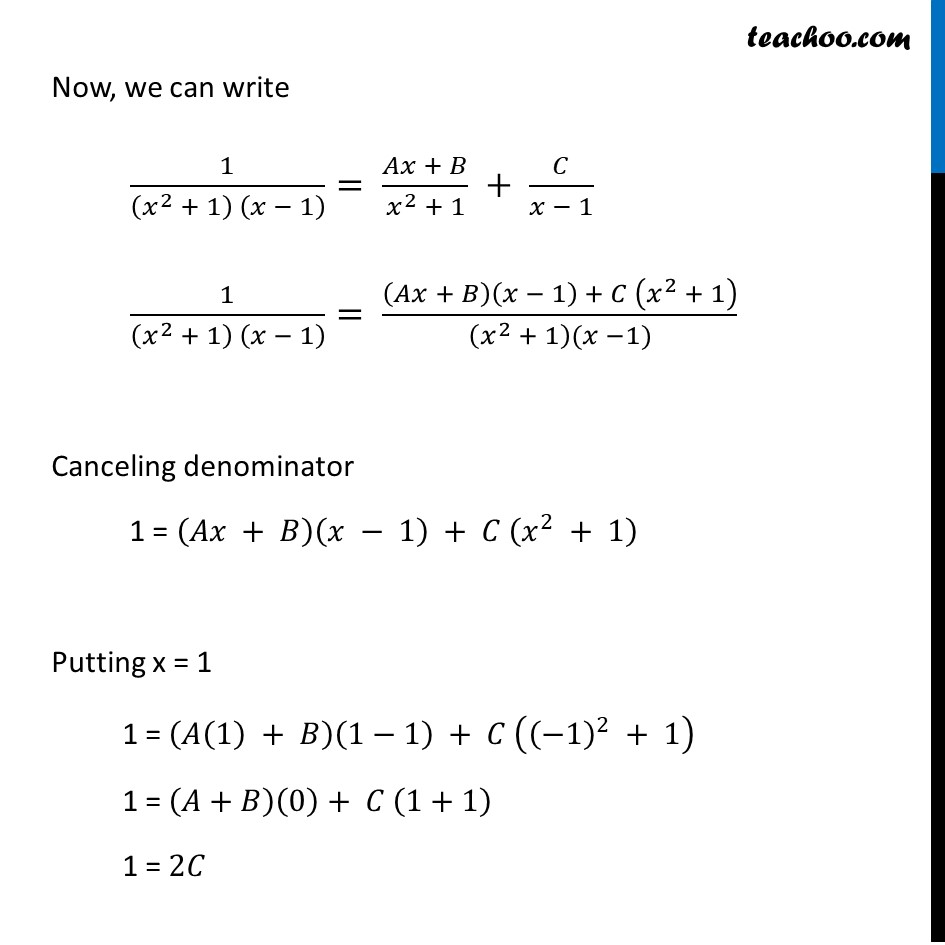
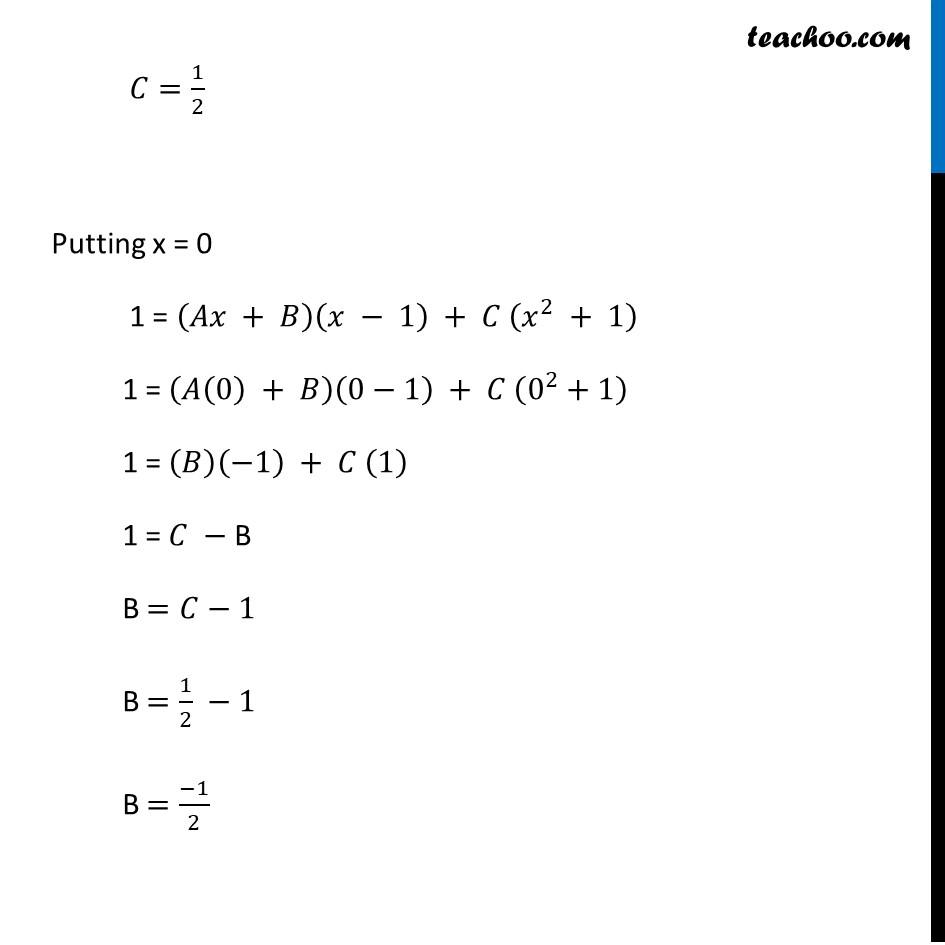
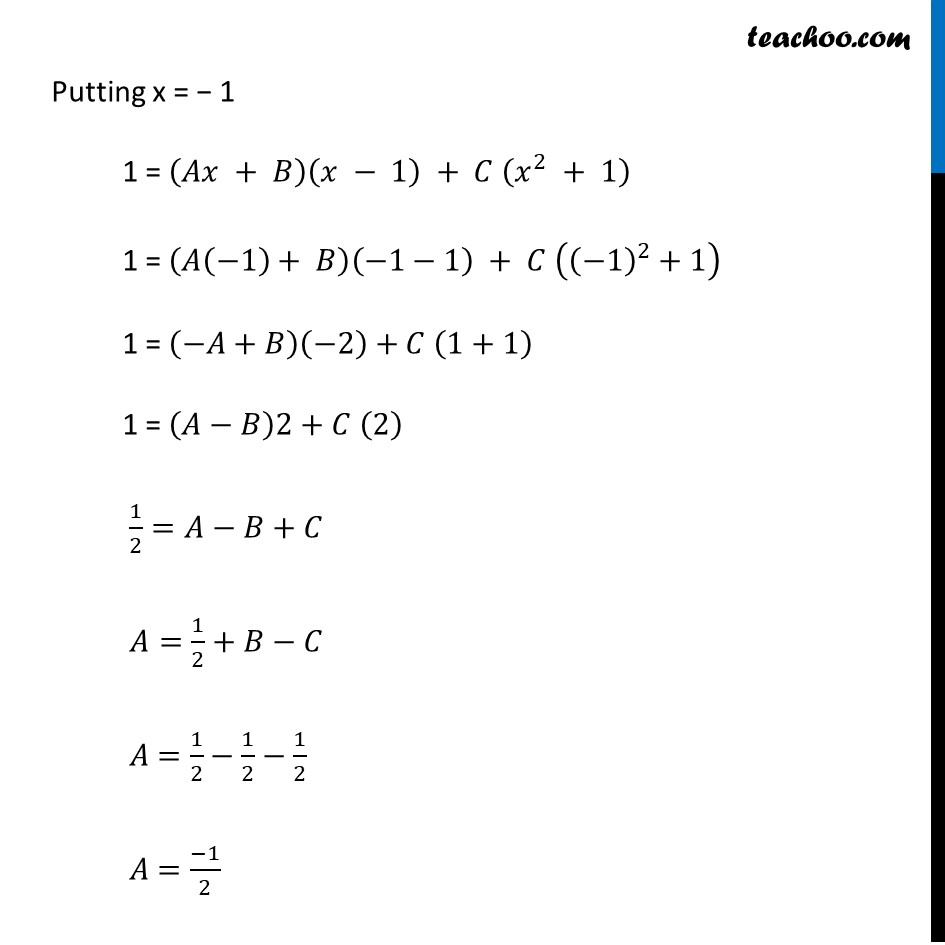
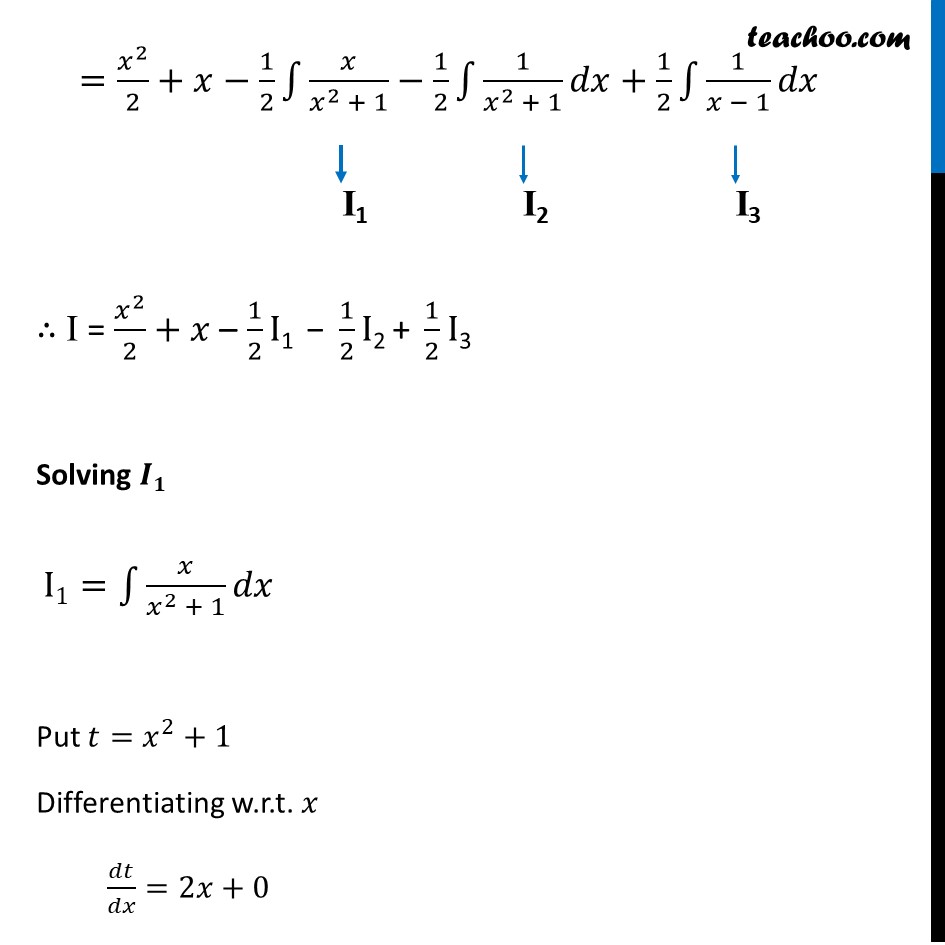
Example 39 Evaluate ﷮﷮ 𝑥﷮4﷯ 𝑑𝑥﷮ 𝑥 −1﷯ 𝑥﷮2﷯ + 1﷯﷯﷯ Let I = ﷮﷮ 𝑥﷮4﷯ 𝑑𝑥﷮ 𝑥 −1﷯ 𝑥﷮2﷯ + 1﷯﷯﷯ 𝑑𝑥 We can write 𝑥﷮4﷯﷮ 𝑥 −1﷯ 𝑥﷮2﷯ + 1﷯﷯= 𝑥﷮4﷯﷮ 𝑥﷮3﷯ − 𝑥﷮2﷯+ 𝑥 − 1﷯ Dividing Numerator by denominator as follows. Hence 𝑥﷮4﷯ = 𝑥﷮3﷯− 𝑥﷮2﷯+𝑥+1﷯ 𝑥+1﷯+1 Thus, 𝑥﷮4﷯﷮ 𝑥﷮3﷯ − 𝑥﷮2﷯ + 𝑥 + 1﷯ = 𝑥+1+ 1﷮ 𝑥﷮3﷯ − 𝑥﷮2﷯ + 𝑥 + 1﷯ = 𝑥+1+ 1﷮ 𝑥 − 1﷯ ( 𝑥﷮2﷯ +1) ﷯ We can write this as 1﷮ 𝑥﷮2﷯ + 1﷯ 𝑥 − 1﷯﷯= 𝐴𝑥 + 𝐵﷮ 𝑥﷮2﷯ + 1﷯ + 𝐶﷮𝑥 − 1﷯ 1﷮ 𝑥﷮2﷯ + 1﷯ 𝑥 − 1﷯﷯= 𝐴𝑥 + 𝐵﷯ 𝑥 − 1﷯ + 𝐶 𝑥﷮2﷯ + 1﷯﷮ 𝑥﷮2﷯ + 1﷯(𝑥 −1)﷯ By canceling denominator 1 = 𝐴𝑥 + 𝐵﷯ 𝑥 − 1﷯ + 𝐶 𝑥﷮2﷯ + 1﷯ Putting x = 1 1 = 𝐴 1﷯ + 𝐵﷯ 1−1﷯ + 𝐶 −1﷯﷮2﷯ + 1﷯ 1 = 𝐴+𝐵﷯ 0﷯+ 𝐶 1+1﷯ 1 = 2𝐶 𝐶= 1﷮2﷯ Similarly putting x = 0 1 = 𝐴 0﷯ + 𝐵﷯ 0−1﷯ + 𝐶 0﷮2﷯+1﷯ 1 = 𝐵﷯ −1﷯ + 𝐶 1﷯ 1 = 𝐶 −B B =𝐶−1 B = 1﷮2﷯ −1 B =− 1﷮2﷯ Similarly putting x = − 1 1 = 𝐴 −1﷯+ 𝐵﷯ −1−1﷯ + 𝐶 −1﷯﷮2﷯+1﷯ 1 = −𝐴+𝐵﷯ −2﷯+𝐶 1+1﷯ 1 = 𝐴−𝐵﷯2+𝐶 2﷯ 1﷮2﷯=𝐴−𝐵+𝐶 𝐴= 1﷮2﷯+𝐵−𝐶 𝐴 = 1﷮2﷯− 1﷮2﷯− 1﷮2﷯ 𝐴 =− 1﷮2﷯ Hence we can write 1﷮ 𝑥﷮2﷯ + 1﷯ 𝑥 − 1﷯﷯ = − 1﷮2﷯ 𝑥 − 1﷮2﷯﷮ 𝑥﷮2﷯ + 1﷯ + 1﷮2﷯﷮𝑥 − 1﷯ = −1﷮2﷯ 𝑥﷮ 𝑥﷮2﷯ + 1﷯ − 1﷮2﷯ 1﷮ 𝑥﷮2﷯ + 1﷯+ 1﷮2 𝑥 − 1﷯﷯ Therefore, we have 𝑥+1﷯+ 1﷮ 𝑥﷮2﷯ + 1﷯ 𝑥 − 1﷯﷯ =(𝑥+1)− 1﷮2﷯ 𝑥﷮ 𝑥﷮2﷯ + 1﷯ − 1﷮2﷯ 1﷮ 𝑥﷮2﷯ + 1﷯+ 1﷮2 𝑥 − 1﷯﷯ Integrating 𝑤.𝑟.𝑡.𝑥. I= ﷮﷮ 𝑥+1﷯+ 1﷮ 𝑥﷮2﷯+1﷯ 𝑥−1﷯﷯ 𝑑𝑥﷯ = ﷮﷮ 𝑥+1﷯− 1﷮2﷯ 𝑥﷮ 𝑥﷮2﷯+1﷯﷯𝑑𝑥− ﷮﷮ 1﷮2﷯ 1﷮ 𝑥﷮2﷯+1﷯ 𝑑𝑥+ ﷮﷮ 1﷮2﷯ 1﷮ 𝑥−1﷯﷯𝑑𝑥﷯﷯﷯﷯ = ﷮﷮ 𝑥+1﷯𝑑𝑥− ﷮﷮ 1﷮2﷯ 𝑑﷮ 𝑥﷮2﷯+1﷯﷯𝑑𝑥− ﷮﷮ 1﷮2﷯ 1﷮ 𝑥﷮2﷯+1﷯𝑑𝑥+ ﷮﷮ 1﷮2﷯ 1﷮ 𝑥−1﷯﷯𝑑𝑥﷯﷯﷯﷯ = 𝑥﷮2﷯﷮2﷯+𝑥− 1﷮2﷯ ﷮﷮ 𝑥﷮ 𝑥﷮2﷯+1﷯− 1﷮2﷯ ﷮﷮ 1﷮ 𝑥﷮2﷯+1﷯𝑑𝑥+ 1﷮2﷯ ﷮﷮ 1﷮𝑥−1﷯𝑑𝑥﷯﷯﷯ ∴ I = 𝑥﷮2﷯﷮2﷯+𝑥 – 1﷮2﷯I1 − 1﷮2﷯I2 + 1﷮2﷯I3 Solving 𝐈𝟏= ﷮﷮ 𝒙﷮ 𝒙﷮𝟐﷯ + 𝟏﷯𝒅𝒙﷯ Put 𝑡= 𝑥﷮2﷯+1 Differentiating w.r.t. 𝑥 𝑑𝑡﷮𝑑𝑥﷯=2𝑥+0 𝑑𝑡﷮2𝑥﷯=𝑑𝑥 Therefore, ﷮﷮ 𝑥 𝑑𝑥﷮ 𝑥﷮2﷯ + 1﷯= ﷮﷮ 𝑥﷮𝑡﷯﷯ 𝑑𝑡﷮2𝑥﷯﷯= ﷮﷮ 1﷮2﷯﷯ 𝑑𝑡﷮𝑡﷯= 1﷮2﷯𝑙𝑜𝑔 𝑡﷯+𝐶1 Putting 𝑡= 𝑥﷮2﷯+1 = 1﷮2﷯𝑙𝑜𝑔 𝑥﷮2﷯+1﷯+𝐶1 I2= ﷮﷮ 1﷮ 𝑥﷮2﷯+ 1﷯𝑑𝑥﷯= tan﷮−1﷯﷮𝑥+﷯𝐶2 I3= ﷮﷮ 1﷮𝑥−1﷯𝑑𝑥﷯=𝑙𝑜𝑔 𝑥−1﷯+𝐶3 Hence 𝐼= 𝑥﷮2﷯﷮2﷯+𝑥− 1﷮2﷯𝐼1− 1﷮2﷯ 𝐼2+ 1﷮2﷯ 𝐼3 = 𝑥﷮2﷯﷮2﷯− 1﷮2﷯ 1﷮2﷯𝑙𝑜𝑔 𝑥﷮2﷯+1﷯+𝐶1﷯− 1﷮2﷯ 𝑡𝑎𝑛﷮−1﷯ 𝑥﷯+ C﷮2﷯﷯− 1﷮2﷯ 𝑙𝑜𝑔 𝑥−1﷯+𝐶3﷯ = 𝑥﷮2﷯﷮2﷯− 1﷮4﷯𝑙𝑜𝑔 𝑥﷮2﷯+1﷯+ 𝐶1﷮2﷯− 1﷮2﷯ tan﷮−1﷯﷮𝑥 𝐶2﷮2﷯+ 1﷮2﷯𝑙𝑜𝑔 𝑥−1﷯+ 𝐶3﷮2﷯﷯ = 𝒙﷮𝟐﷯﷮𝟐﷯+ 𝟏﷮𝟐﷯𝒍𝒐𝒈 𝒙−𝟏﷯− 𝟏﷮𝟒﷯𝒍𝒐𝒈 𝒙﷮𝟐﷯+𝟏﷯− 𝟏﷮𝟐﷯ 𝒕𝒂𝒏﷮−𝟏﷯﷮𝒙+𝑪﷯