Example 34 - Integration of log (sin x) from 0 to pi/2

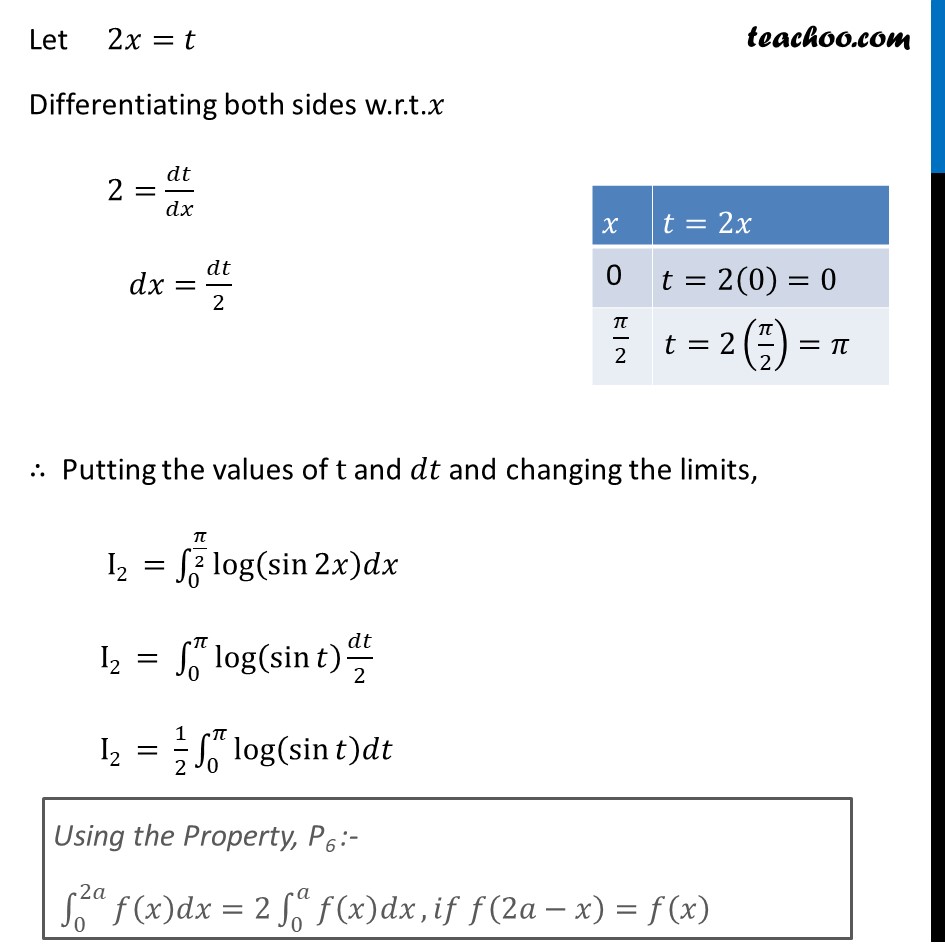
Example 34 Evaluate ∫_0^(𝜋/2 )▒log⁡sin⁡𝑥 𝑑𝑥 Let I1=∫_0^(𝜋/2 )▒𝑙𝑜𝑔(𝑠𝑖𝑛𝑥) 𝑑𝑥 ∴ I1=∫_0^(𝜋/2)▒𝑠𝑖𝑛(𝜋/2−𝑥)𝑑𝑥 I1= ∫_0^(𝜋/2)▒𝑙𝑜𝑔(cos⁡𝑥 )𝑑𝑥 Adding (1) and (2) i.e. (1) + (2) I1+ I1=∫_0^(𝜋/2)▒〖𝑙𝑜𝑔(sin⁡𝑥 )𝑑𝑥+∫_0^(𝜋/2)▒𝑙𝑜𝑔(cos⁡𝑥 )𝑑𝑥〗 2I1 =∫_0^(𝜋/2)▒〖log⁡[sin⁡〖𝑥 cos⁡𝑥 〗 ] 𝑑𝑥〗 2I1 = ∫_0^(𝜋/2)▒〖log⁡[2sin⁡〖𝑥 cos⁡𝑥 〗/2] 𝑑𝑥〗 2I1 = ∫_0^(𝜋/2)▒[log[2sin⁡〖𝑥 cos⁡𝑥 〗 ]−log⁡2 ]𝑑𝑥 2I1 = ∫_0^(𝜋/2)▒[log[sin⁡2𝑥 ]−log⁡2 ]𝑑𝑥 2I1 = ∫_0^(𝜋/2)▒log[sin⁡2𝑥 ]𝑑𝑥−∫_0^(𝜋/2)▒〖log 2 𝑑𝑥〗 Solving 𝐈𝟐 I2=∫_0^(𝜋/2)▒〖log sin⁡2𝑥 𝑑𝑥〗 Let 2𝑥=𝑡 Differentiating both sides w.r.t.𝑥 2=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/2 ∴ Putting the values of t and 𝑑𝑡 and changing the limits, I2 =∫_0^(𝜋/2)▒log(sin⁡2𝑥 )𝑑𝑥 I2 = ∫_0^𝜋▒〖log(sin⁡𝑡 ) 𝑑𝑡/2〗 I2 = 1/2 ∫_0^𝜋▒log(sin⁡𝑡 )𝑑𝑡 Here, 𝑓(𝑡)=log⁡𝑠𝑖𝑛𝑡 𝑓(2𝑎−𝑡)=𝑓(2𝜋−𝑡)=log⁡𝑠𝑖𝑛(2𝜋−𝑡)=log⁡sin⁡𝑡 Since 𝑓(𝑡)=𝑓(2𝑎−𝑡) ∴ I2 = 1/2 ∫_0^𝜋▒log⁡sin⁡〖𝑡 𝑑𝑡〗 =1/2 ×2∫_0^(𝜋/2)▒log⁡sin⁡〖𝑡. 𝑑𝑡〗 =∫_0^(𝜋/2)▒log⁡sin⁡〖𝑡. 𝑑𝑡〗 I2=∫_0^(𝜋/2)▒log⁡sin⁡〖𝑥 𝑑𝑥〗 Putting the value of I2 in equation (3), we get 2I1 =∫_𝟎^(𝝅/𝟐)▒𝐥𝐨𝐠[𝒔𝒊𝒏⁡𝟐𝒙 ]⁡𝒅𝒙 −∫_0^(𝜋/2)▒log(2)⁡𝑑𝑥 2I1 = ∫_𝟎^(𝝅/𝟐)▒𝐥𝐨𝐠(𝒔𝒊𝒏⁡𝒙 )⁡𝒅𝒙 −log(2) ∫_0^(𝜋/2)▒〖1.〗⁡𝑑𝑥 2I1 = 𝐈𝟏 − log(2) [𝑥]_0^(𝜋/2) 2I1−I1=−log⁡2 [𝜋/2−0] I1=−log⁡2 [𝜋/2] ∴ 𝐈𝟏=(− 𝝅)/𝟐 𝐥𝐨𝐠⁡𝟐

Example 34 - Chapter 7 Class 12 Integrals (Important Question)

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular