Example 29 - Chapter 7 Class 12 Integrals

Transcript

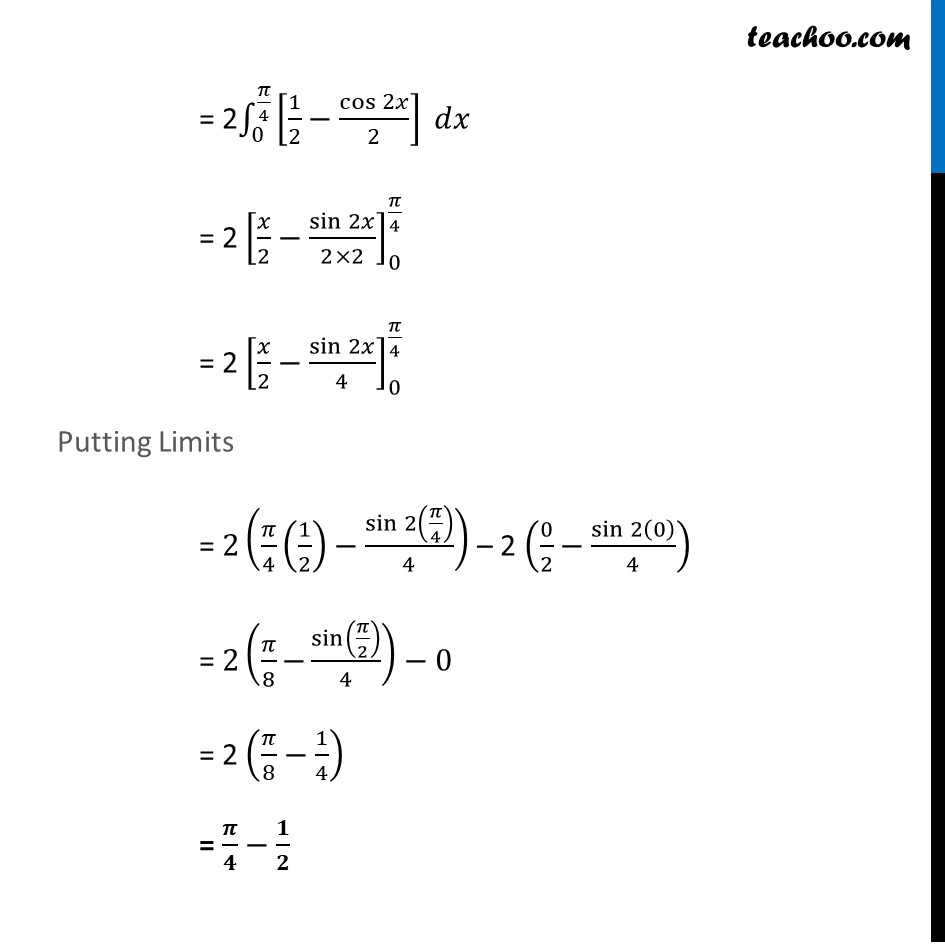
Example 29 Evaluate ∫_((−𝜋)/4)^(𝜋/4)▒sin^2⁡𝑥 𝑑𝑥 Let f(x) = 〖𝑠𝑖𝑛〗^2 𝑥 f(-x) = 〖𝑠𝑖𝑛〗^2 (−𝑥)=(−sin⁡𝑥 )^2=〖𝑠𝑖𝑛〗^2 𝑥 Since f(x) = f(-x) Hence, 〖𝑠𝑖𝑛〗^2 𝑥 is an even function ∫_((−𝜋)/4)^(𝜋/4)▒sin^2⁡𝑥 𝑑𝑥=∫_0^(𝜋/4)▒sin^2⁡𝑥 𝑑𝑥 = ∫_0^(𝜋/4)▒((1 − cos⁡〖2 〗 𝑥)/2) 𝑑𝑥 = 2∫_0^(𝜋/4)▒〖[1/2−(cos⁡2 𝑥)/2] 𝑑𝑥〗 = 2 [𝑥/2−sin⁡2𝑥/(2×2)]_0^(𝜋/4) = 2 [𝑥/2−sin⁡2𝑥/4]_0^(𝜋/4) Putting Limits = 2(𝜋/4 (1/2)−sin⁡2(𝜋/4)/4) – 2 (0/2−sin⁡2(0)/4) = 2(𝜋/8 −⁡〖sin⁡(𝜋/2)/4〗 )−0 = 2 (𝜋/8−1/4) = 𝝅/𝟒−𝟏/𝟐