Example 20 - Chapter 7 Class 12 Integrals

Transcript

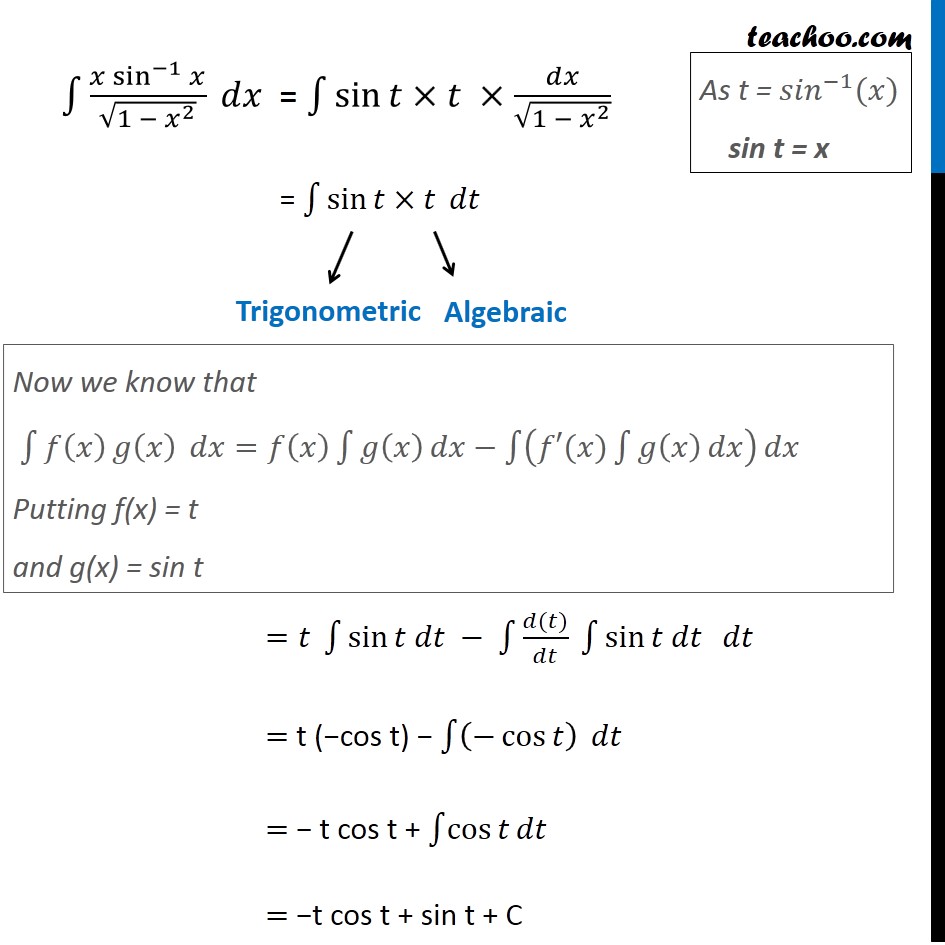
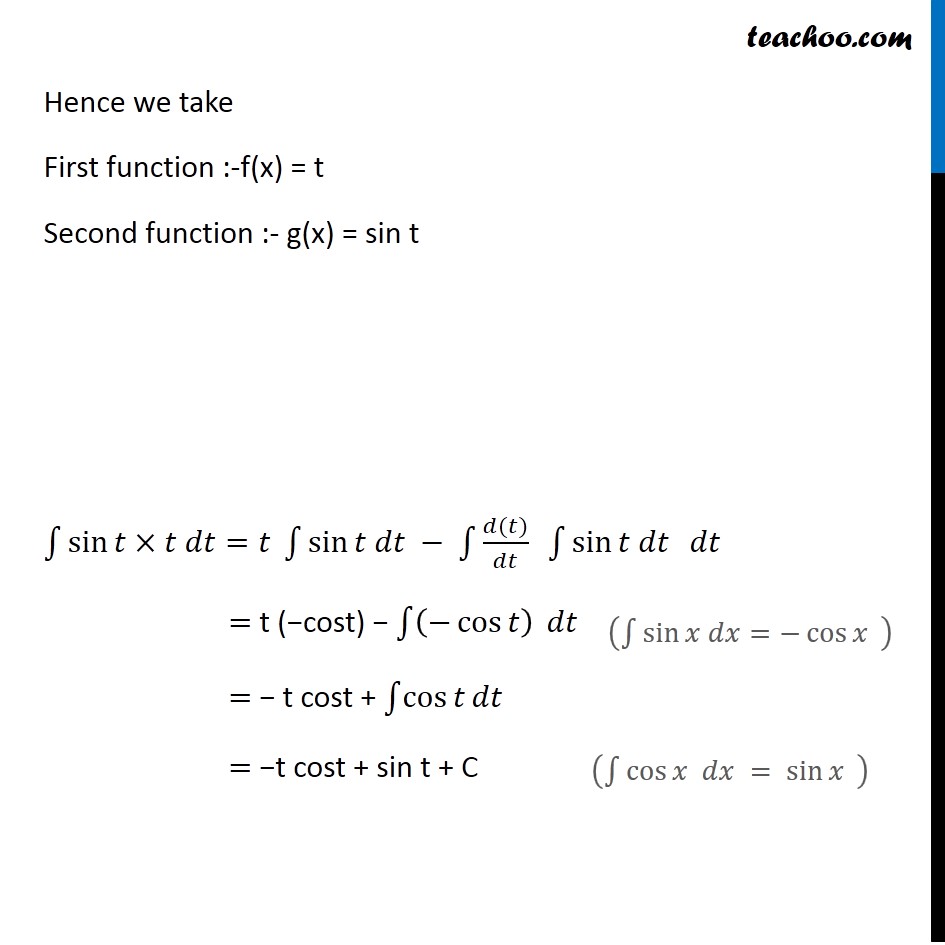
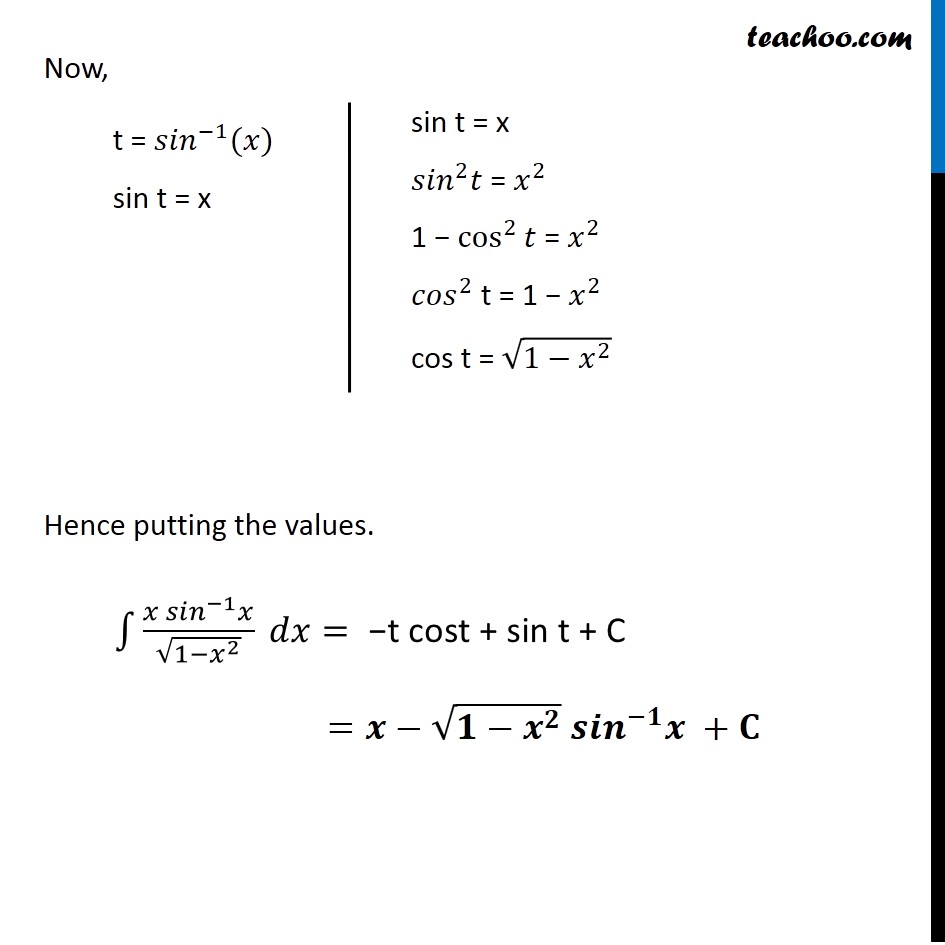
Example 20 Find ∫1▒(𝑥 sin^(−1)⁡𝑥)/√(1 − 𝑥^2 ) 𝑑𝑥 Example 20 Find ∫1▒(𝑥 sin^(−1)⁡𝑥)/√(1 − 𝑥^2 ) 𝑑𝑥 ∫1▒(𝑥 sin^(−1)⁡𝑥)/√(1 − 𝑥^2 ) 𝑑𝑥 Let t = 〖𝑠𝑖𝑛〗^(−1) (𝑥) 𝑑𝑡/𝑑𝑥=1/√(1 − 𝑥^2 ) dt = 𝑑𝑥/√(1 − 𝑥^2 ) So, our equation becomes ∫1▒(𝑥 sin^(−1)⁡𝑥)/√(1 − 𝑥^2 ) 𝑑𝑥 = ∫1▒〖sin⁡〖𝑡×𝑡 〗×𝑑𝑥/√(1 − 𝑥^2 )〗 = ∫1▒〖sin⁡〖𝑡×𝑡 〗 𝑑𝑡〗 =𝑡 ∫1▒〖sin⁡〖𝑡 𝑑𝑡 − ∫1▒(𝑑(𝑡))/𝑑𝑡〗 〗 ∫1▒sin⁡〖𝑡 𝑑𝑡 〗 𝑑𝑡 = t (−cos t) − ∫1▒(−cos⁡𝑡 ) 𝑑𝑡 = − t cos t + ∫1▒cos⁡𝑡 𝑑𝑡 = −t cos t + sin t + C Now we know that ∫1▒〖𝑓(𝑥) 𝑔⁡(𝑥) 〗 𝑑𝑥=𝑓(𝑥) ∫1▒𝑔(𝑥) 𝑑𝑥−∫1▒(𝑓′(𝑥)∫1▒𝑔(𝑥) 𝑑𝑥) 𝑑𝑥 Putting f(x) = t and g(x) = sin t Hence we take First function :-f(x) = t Second function :- g(x) = sin t ∫1▒〖sin⁡〖𝑡×𝑡 𝑑𝑡=𝑡 ∫1▒〖sin⁡〖𝑡 𝑑𝑡 − ∫1▒(𝑑(𝑡))/𝑑𝑡〗 〗〗 〗 ∫1▒sin⁡〖𝑡 𝑑𝑡 〗 𝑑𝑡 = t (−cost) − ∫1▒(−cos⁡𝑡 ) 𝑑𝑡 = − t cost + ∫1▒cos⁡𝑡 𝑑𝑡 = −t cost + sin t + C (∫1▒sin⁡〖𝑥 𝑑𝑥=−cos⁡𝑥 〗 " " ) (∫1▒cos⁡〖𝑥 𝑑𝑥 = sin⁡𝑥 〗 " " ) t = 〖𝑠𝑖𝑛〗^(−1) (𝑥) sin t = x sin t = x 〖𝑠𝑖𝑛〗^2 𝑡 = 𝑥^2 1 − cos^2⁡𝑡 = 𝑥^2 〖𝑐𝑜𝑠〗^2 t = 1 − 𝑥^2 cos t = √(1−𝑥^2 ) Now, Hence putting the values. ∫1▒(𝑥 〖𝑠𝑖𝑛〗^(−1) 𝑥)/√(1−𝑥^2 ) 𝑑𝑥=" −t cost + sin t + C" =𝒙−√(𝟏−𝒙^𝟐 ) 〖𝒔𝒊𝒏〗^(−𝟏) 𝒙 +𝐂