Example 10 (i) - Chapter 7 Class 12 Integrals (Important Question)

Transcript

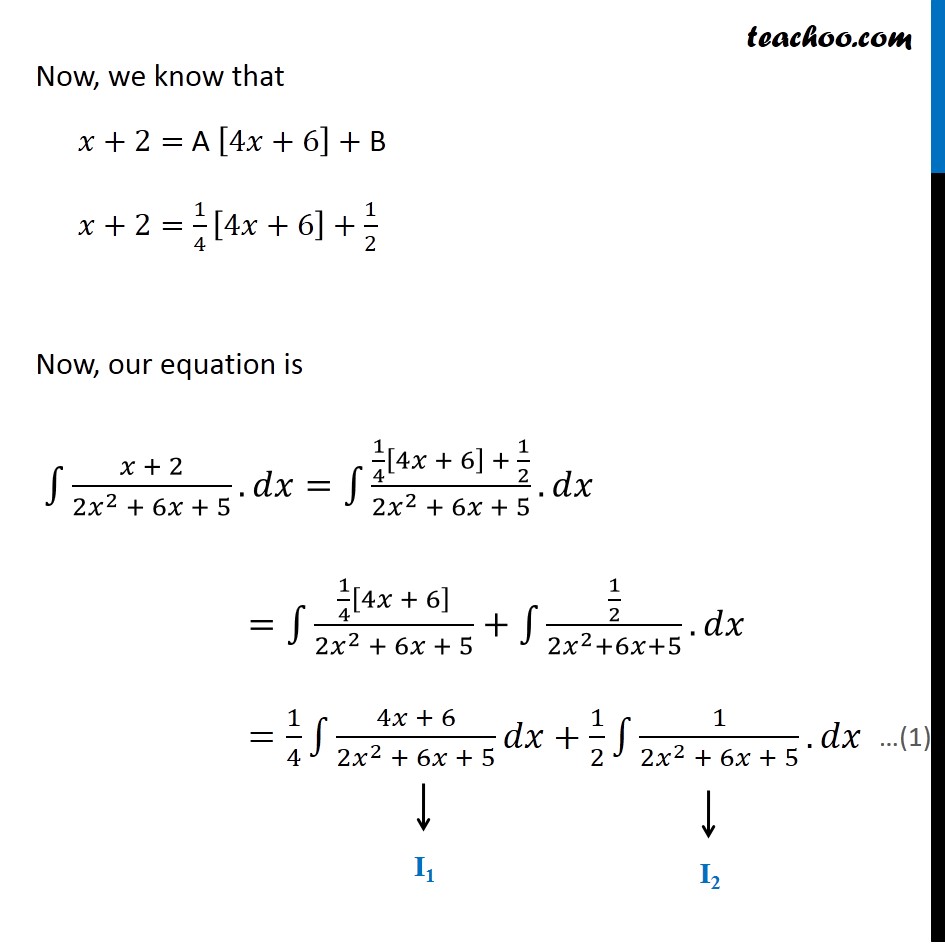
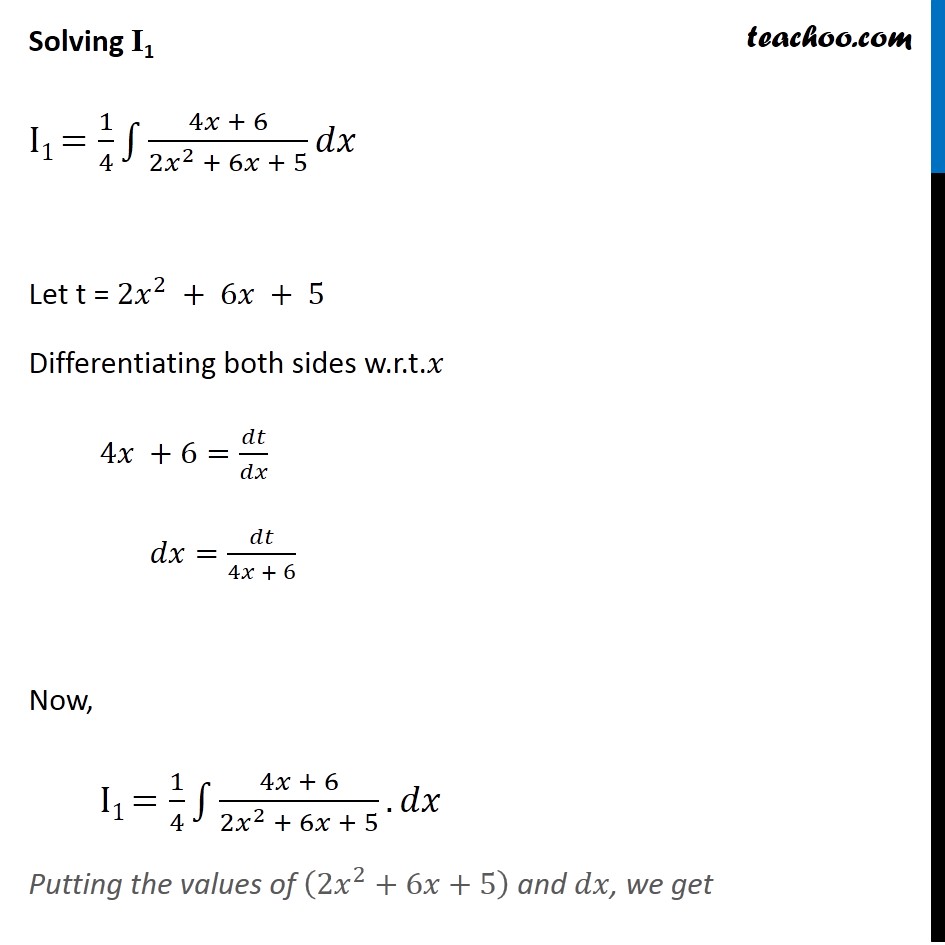
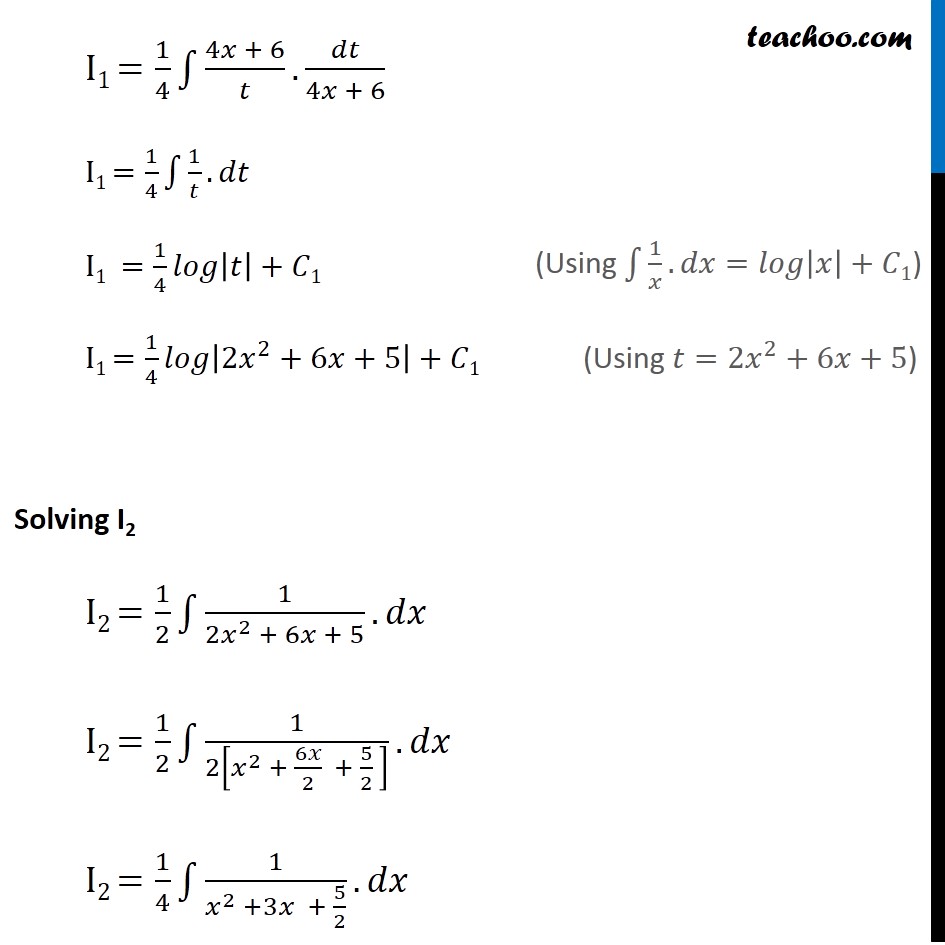
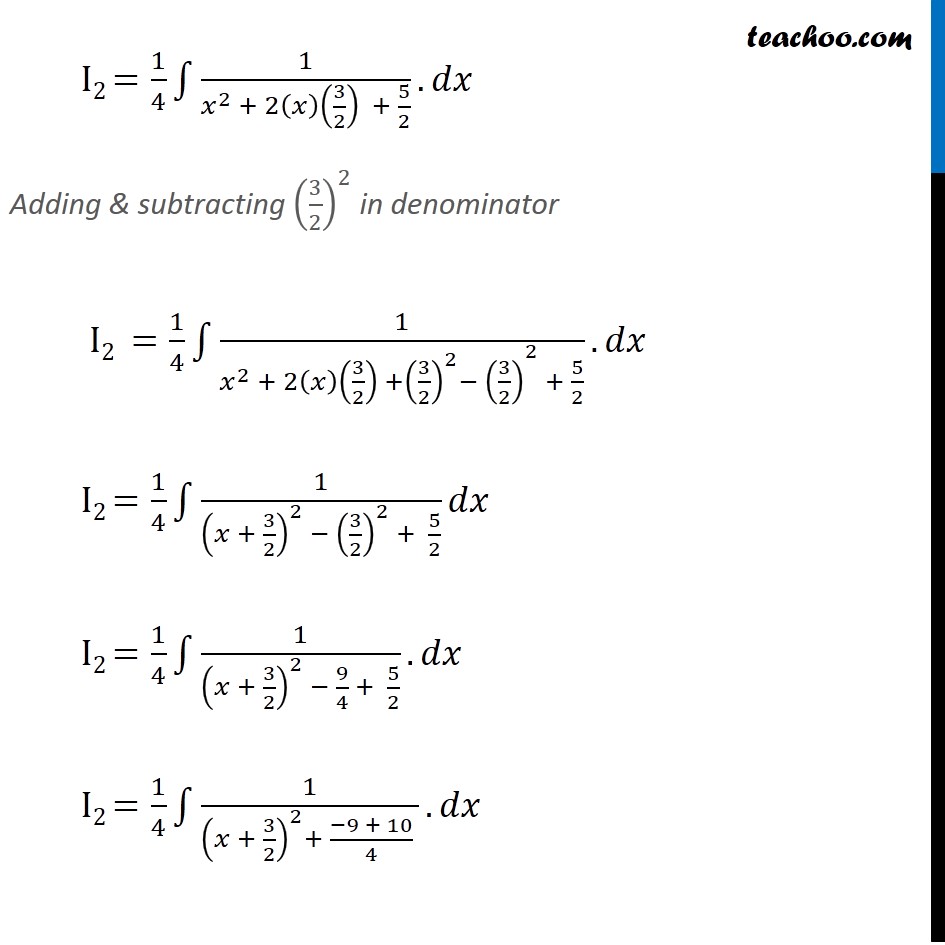
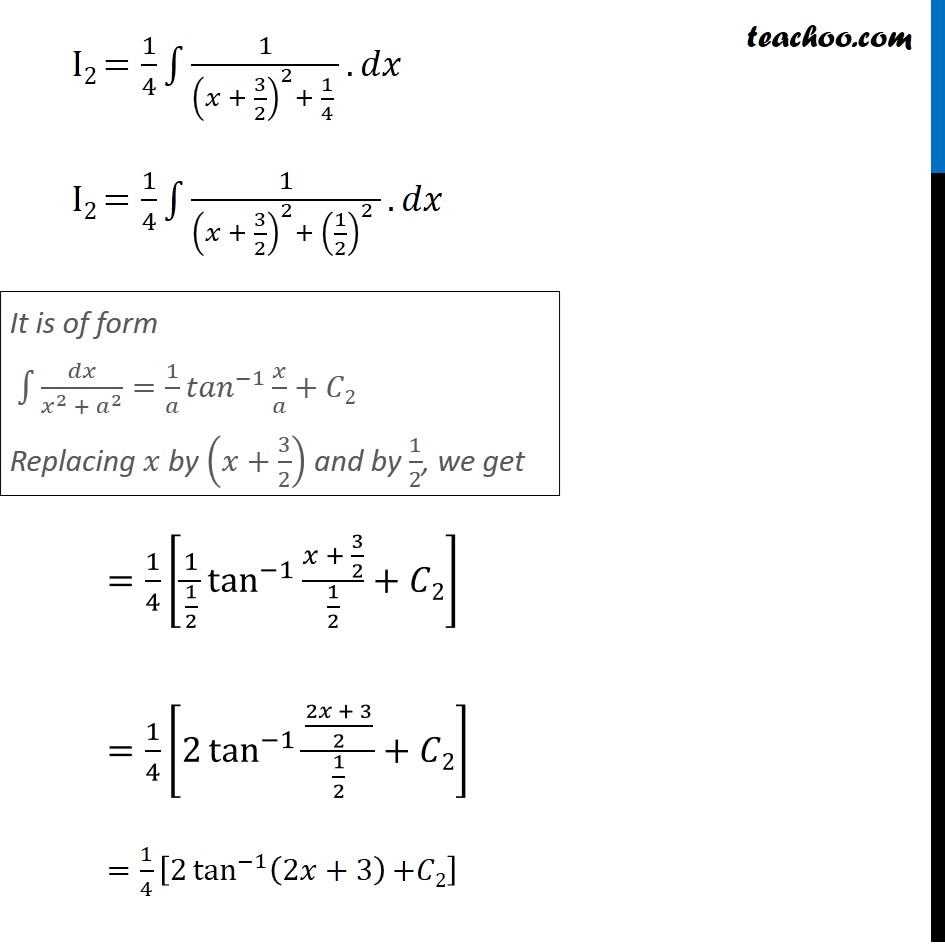
Example 10 Find the following integrals: (i) ∫1▒(𝑥 + 2)/(2𝑥^2 + 6𝑥 + 5 ) 𝑑𝑥 We can write numerator as 𝑥+2= A 𝑑/𝑑𝑥 (2𝑥^2+6𝑥+5) + B 𝑥+2= A [4𝑥+6]+ B 𝑥+2=4𝐴𝑥+6A+B Finding A & B Comparing coefficient of 𝑥 𝑥=4𝐴𝑥 1 =4A A=1/4 Comparing constant term 2=6A+B 2=6(1/4)+B 2=3/2+B B=2−3/2=1/2 Now, we know that 𝑥+2= A [4𝑥+6]+ B 𝑥+2=1/4 [4𝑥+6]+1/2 Now, our equation is ∫1▒〖(𝑥 + 2)/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥=∫1▒〖(1/4 [4𝑥 + 6] + 1/2)/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥〗〗 =∫1▒〖(1/4 [4𝑥 + 6])/(2𝑥^2 + 6𝑥 + 5)+∫1▒〖(1/2)/(2𝑥^2+6𝑥+5).𝑑𝑥〗〗 =1/4 ∫1▒〖(4𝑥 + 6)/(2𝑥^2 + 6𝑥 + 5) 𝑑𝑥+1/2 ∫1▒〖1/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥〗〗 Solving I1 I1 =1/4 ∫1▒〖(4𝑥 + 6)/(2𝑥^2 + 6𝑥 + 5) 𝑑𝑥〗 Let t = 2𝑥^2 + 6𝑥 + 5 Differentiating both sides w.r.t.𝑥 4𝑥 +6=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/(4𝑥 + 6) Now, I1 =1/4 ∫1▒〖(4𝑥 + 6)/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥〗 Putting the values of (2𝑥^2+6𝑥+5) and 𝑑𝑥, we get I1 =1/4 ∫1▒〖(4𝑥 + 6)/𝑡.𝑑𝑡/(4𝑥 + 6) 〗 I1 =1/4 ∫1▒〖1/𝑡.𝑑𝑡 〗 I1 =1/4 𝑙𝑜𝑔|𝑡|+𝐶1 I1 =1/4 𝑙𝑜𝑔|2𝑥^2+6𝑥+5|+𝐶1 Solving I2 I2 =1/2 ∫1▒〖1/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥 〗 I2 =1/2 ∫1▒〖1/2[𝑥^2 + 6𝑥/2 + 5/2 ] .𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/(𝑥^2 +3𝑥 + 5/2).𝑑𝑥 〗 (Using ∫1▒〖1/𝑥.𝑑𝑥=𝑙𝑜𝑔|𝑥|+𝐶1〗) (Using 𝑡=2𝑥^2+6𝑥+5) I2 =1/4 ∫1▒〖1/(𝑥^2 + 2(𝑥)(3/2) + 5/2).𝑑𝑥 〗 Adding & subtracting (3/2)^2 in denominator I2 =1/4 ∫1▒〖1/(𝑥^2 + 2(𝑥) 〖(3/2) +(3/2)^2− (3/2)〗^2 + 5/2).𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/((𝑥 + 3/2)^2 − (3/2)^2 + 5/2) 𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/((𝑥 + 3/2)^2 − 9/4 + 5/2).𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/((𝑥 + 3/2)^2+ (−9 + 10)/4 ).𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/((𝑥 + 3/2)^2+ 1/4 ).𝑑𝑥 〗 I2 =1/4 ∫1▒〖1/((𝑥 + 3/2)^2+ (1/2)^2 ).𝑑𝑥 〗 =1/4 [1/(1/2) tan^(−1)⁡〖(𝑥 + 3/2)/(1/2)+𝐶2〗 ] =1/4 [2 tan^(−1)⁡〖((2𝑥 + 3)/2)/(1/2)+𝐶2〗 ] =1/4 [2 〖tan^(−1) (2𝑥+3)〗⁡〖+𝐶2〗 ] It is of form ∫1▒〖𝑑𝑥/(𝑥^2 + 𝑎^2 )=1/𝑎 〖𝑡𝑎𝑛〗^(−1)⁡〖𝑥/𝑎+𝐶2" " 〗 〗 Replacing 𝑥 by (𝑥+3/2) and by 1/2, we get =2/4 tan^(−1)⁡〖(2𝑥+3)+𝐶2/4〗 =1/2 tan^(−1)⁡〖(2𝑥+3)+𝐶3〗 Now, putting the value of I1 and I2 in eq. (1) ∴ ∫1▒〖(𝑥+2)/(2𝑥^2 + 6𝑥 + 5).𝑑𝑥〗 =1/4 𝑙𝑜𝑔|2𝑥^2+6𝑥+5|+𝐶1+1/2 tan^(−1)⁡〖(2𝑥+3)+〗 𝐶3 =𝟏/𝟒 𝒍𝒐𝒈|𝟐𝒙^𝟐+𝟔𝒙+𝟓|+𝟏/𝟐 〖𝒕𝒂𝒏〗^(−𝟏)⁡〖(𝟐𝒙+𝟑)+〗 𝑪 (where 𝐶3 = 𝐶2/4 )