Chapter 7 Class 12 Integrals

Chapter 8 Class 12 Application of Integrals →
Class 12
Important Questions for exams Class 12

Example 9 (i) - Chapter 7 Class 12 Integrals (Important Question)

Last updated at Dec. 16, 2024 by

Example 9 - Find integrals (i) dx / x2 - 6x + 13 - Class 12

Example 9 - Chapter 7 Class 12 Integrals - Part 2

 

Share on WhatsApp

Transcript

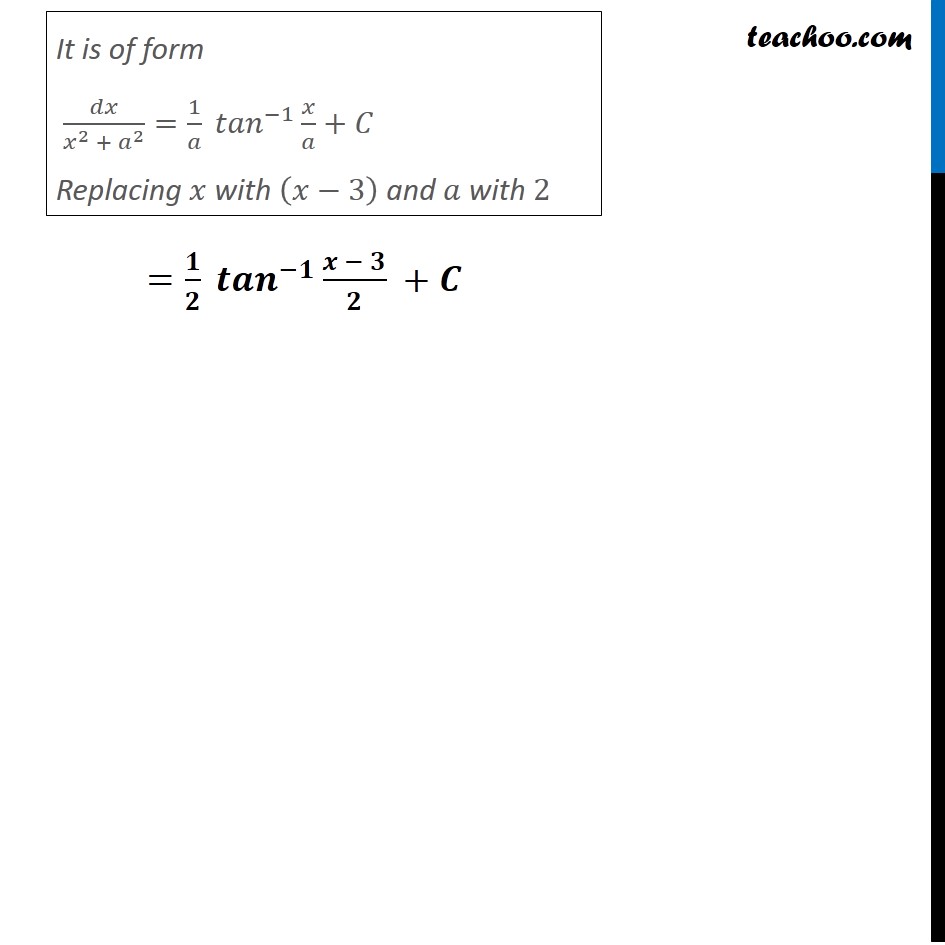
Example 9 Find the following integrals: (i) ∫1▒𝑑𝑥/(𝑥^2− 6𝑥 + 13) ∫1▒𝑑𝑥/(𝑥^2− 6𝑥 + 13) =∫1▒𝑑𝑥/(𝑥^2− 2 × 3 × 𝑥 + 13) = ∫1▒𝑑𝑥/((𝑥^2 − 2 . 3 𝑥 + 3^2 ) + 13 − 3^2 ) = ∫1▒𝑑𝑥/((𝑥 − 3)^2 + 13 − 9) = ∫1▒𝑑𝑥/((𝑥 − 3)^2 + 4) = ∫1▒𝑑𝑥/((𝑥 − 3)^2 + 2^2 ) ("Adding and subtracting " (3)^2 ) ("Using " 𝑎^2−2𝑎𝑏+𝑏^2=(𝑎−𝑏)^2 ) It is of form 𝑑𝑥/(𝑥^2 + 𝑎^2 )=1/𝑎 〖𝑡𝑎𝑛〗^(−1)⁡〖𝑥/𝑎〗+𝐶 Replacing 𝑥 with (𝑥−3) and 𝑎 with 2 =𝟏/𝟐 〖𝒕𝒂𝒏〗^(−𝟏)⁡〖(𝒙 − 𝟑)/𝟐〗 +𝑪

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo