Chapter 7 Class 12 Integrals
Concept wise

Ex 7.1, 22 (MCQ) - Chapter 7 Class 12 Integrals

Last updated at July 28, 2026 by

Ex 7.1, 22 - If f'(x) = 4x3 - 3/x4, f(2) = 0, then f(x) is

Ex 7.1, 22 - Chapter 7 Class 12 Integrals - Part 2
Ex 7.1, 22 - Chapter 7 Class 12 Integrals - Part 3

Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 5 questions, selected from your answers, mistakes, and progress.
Remove Ads

Transcript

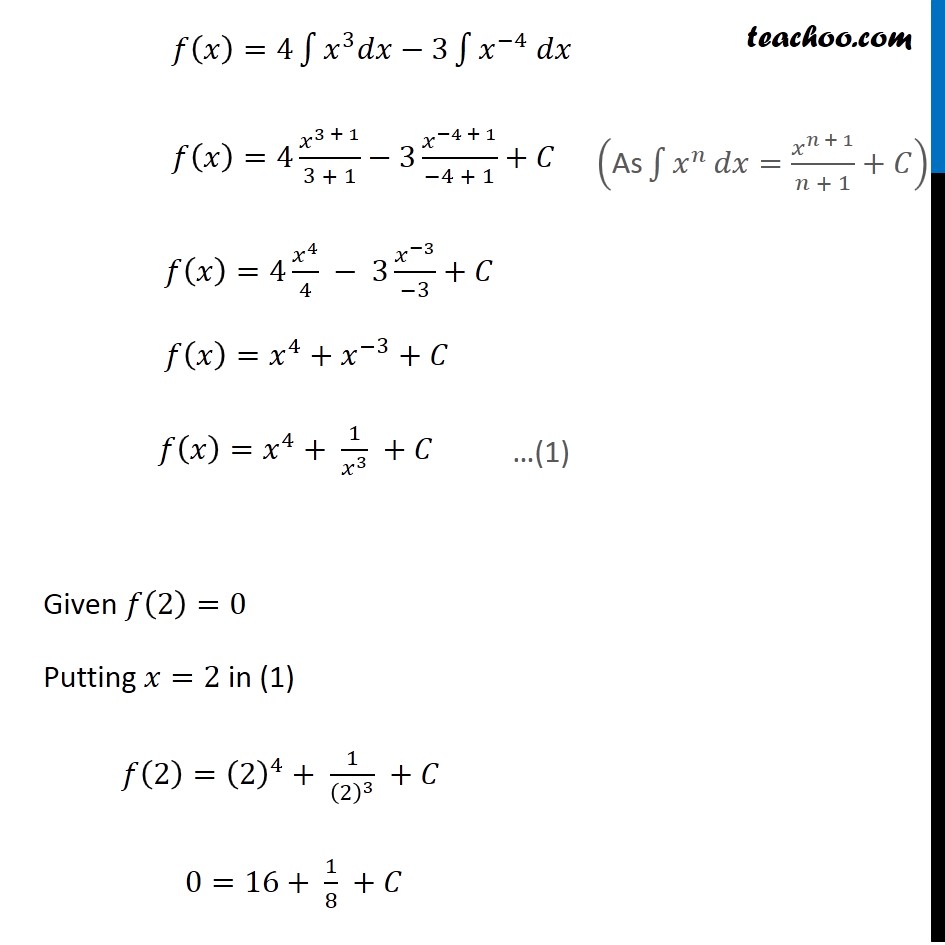
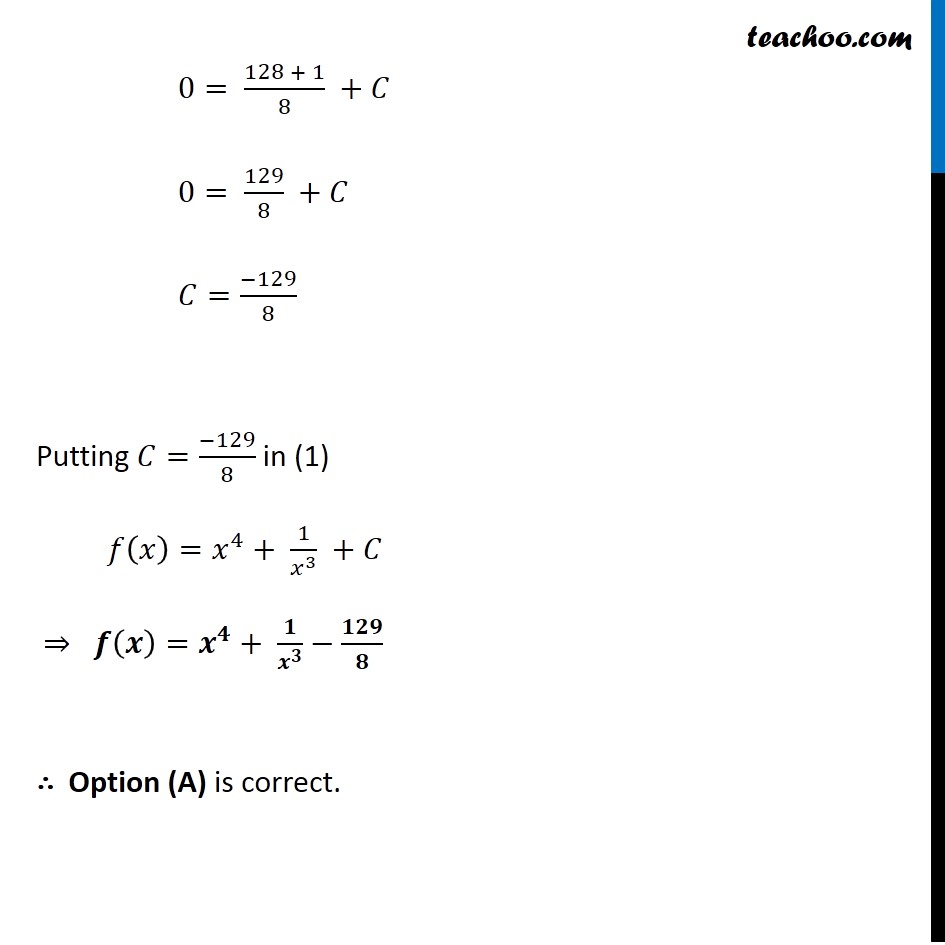
Ex 7.1, 22 If š‘‘/š‘‘š‘„ f(x) = 4x3 āˆ’ 3/š‘„4 such that f(2) = 0, then f(x) is x4 + 1/š‘„3 āˆ’ 129/8 (B) x3 + 1/š‘„4 + 129/8 (C) x4 + 1/š‘„3 + 129/8 (D) x3 + 1/š‘„4 āˆ’ 129/8 Given š‘‘/š‘‘š‘„ f(x) = 4x3 āˆ’ 3/š‘„4 Integrating both sides āˆ«1ā–’怖š‘‘/š‘‘š‘„ š‘“(š‘„) 怗=āˆ«1ā–’(4š‘„^3āˆ’ 3/š‘„^4 )š‘‘š‘„ āˆ«1ā–’š‘‘/š‘‘š‘„ š‘“(š‘„)=4āˆ«1ā–’怖š‘„^3 š‘‘š‘„怗āˆ’3āˆ«1ā–’怖1/š‘„^4 š‘‘š‘„怗 š‘“(š‘„)=4āˆ«1ā–’怖š‘„^3 š‘‘š‘„怗āˆ’3āˆ«1ā–’怖š‘„^(āˆ’4) š‘‘š‘„怗 š‘“(š‘„)=4 š‘„^(3 + 1)/(3 + 1)āˆ’3 š‘„^(āˆ’4 + 1)/(āˆ’4 + 1)+š¶ š‘“(š‘„)=4 š‘„^4/4 āˆ’ 3 š‘„^(āˆ’3)/(āˆ’3)+š¶ š‘“(š‘„)=š‘„^4+š‘„^(āˆ’3)+š¶ š‘“(š‘„)=š‘„^4+ 1/š‘„^3 +š¶ Given š‘“(2)=0 Putting š‘„=2 in (1) š‘“(2)=(2)^4+ 1/(2)^3 +š¶ 0=16+ 1/8 +š¶ 0= (128 + 1)/8 +š¶ 0= 129/8 +š¶ š¶=(āˆ’129)/8 Putting š¶=(āˆ’129)/8 in (1) š‘“(š‘„)=š‘„^4+ 1/š‘„^3 +š¶ ā‡’ š’‡(š’™)=š’™^šŸ’+ šŸ/š’™^šŸ‘ āˆ’šŸšŸšŸ—/šŸ– āˆ“ Option (A) is correct.

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.