Ex 9.3, 18 - Chapter 9 Class 12 Differential Equations

Transcript

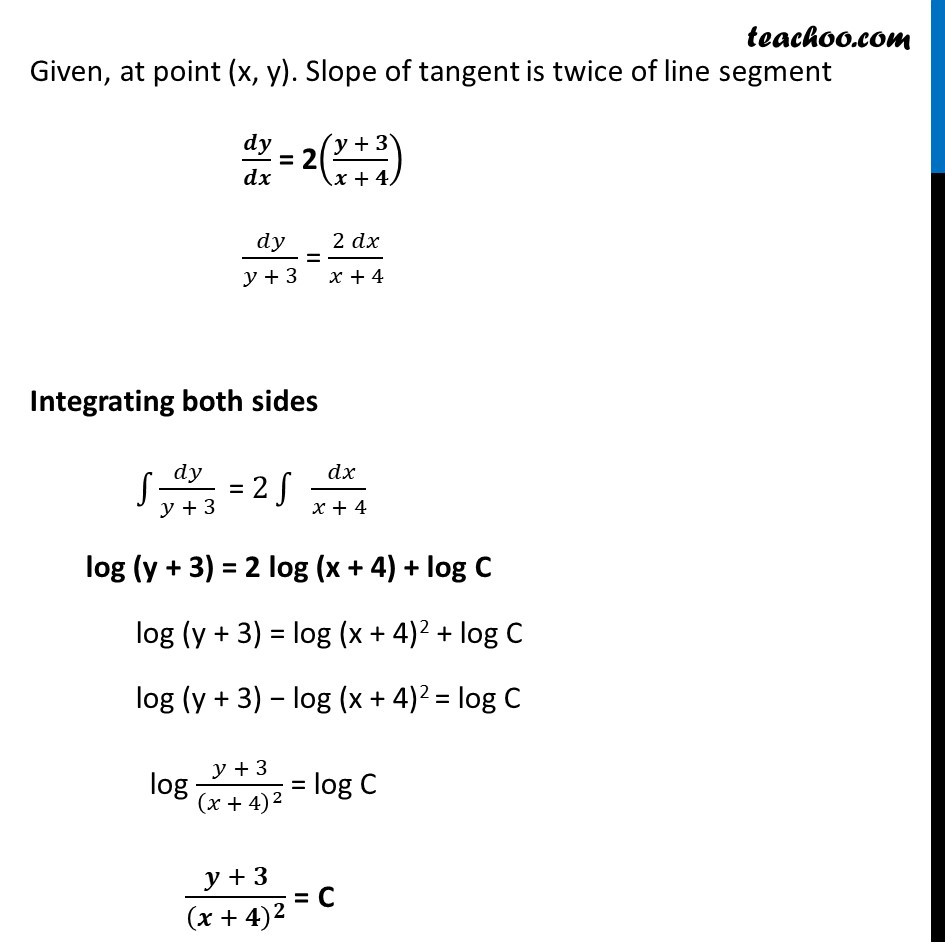
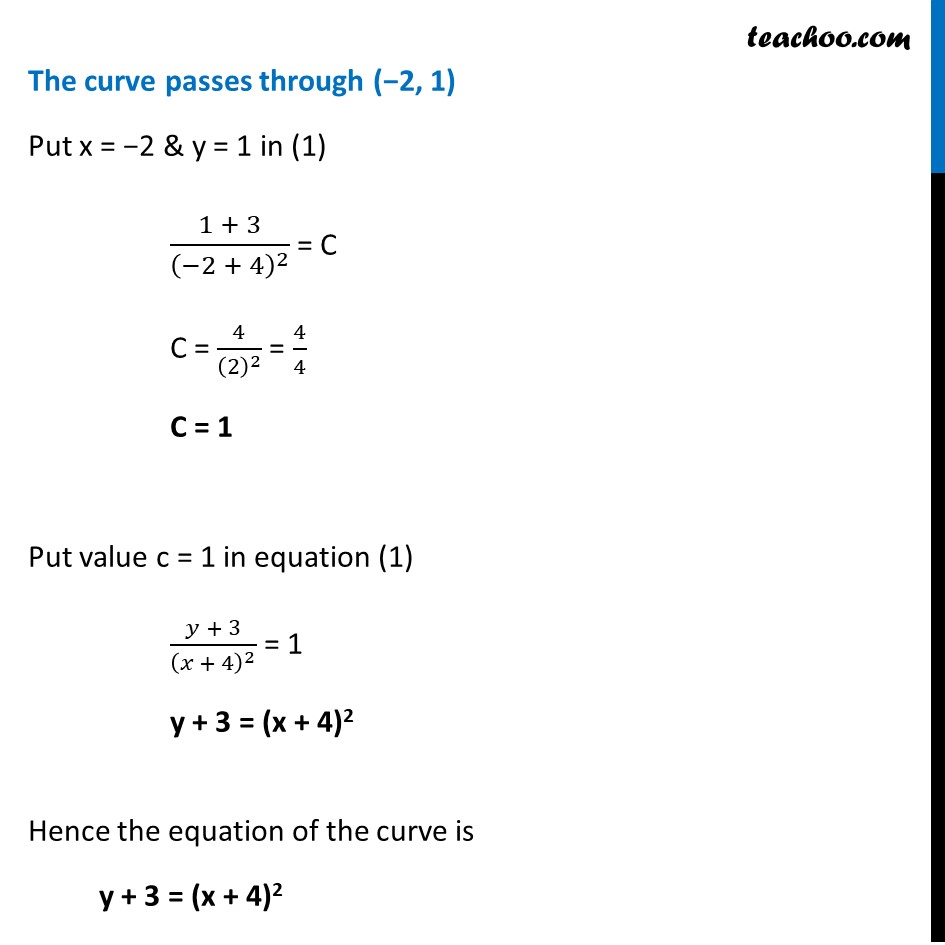
Ex 9.3, 18 At any point (𝑥 , 𝑦) of a curve , the slope of the tangent is twice the slope of the line segment joining the point of contact to the point (−4 , −3) . Find the equation of the curve given that its passes through(−2 , 1) Slope of tangent to the curve = 𝒅𝒚/𝒅𝒙 Slope of line segment joining (x, y) & (−4, −3) = (𝑦2 − 𝑦1)/(𝑥2 −𝑥1) = (−𝟑 − 𝒚)/(−𝟒 − 𝒙) = (−(𝑦 + 3))/(−(𝑥 + 4)) = (𝒚 + 𝟑)/(𝒙 + 𝟒) Given, at point (x, y). Slope of tangent is twice of line segment 𝒅𝒚/𝒅𝒙 = 2((𝒚 + 𝟑)/(𝒙 + 𝟒)) 𝑑𝑦/(𝑦 + 3) = (2 𝑑𝑥)/(𝑥 + 4) Integrating both sides ∫1▒〖𝑑𝑦/(𝑦 + 3) " " 〗= 2∫1▒〖" " ( 𝑑𝑥)/(𝑥 + 4)〗 log (y + 3) = 2 log (x + 4) + log C log (y + 3) = log (x + 4)2 + log C log (y + 3) − log (x + 4)2 = log C log (𝑦 + 3)/(𝑥 + 4)^2 = log C (𝒚 + 𝟑)/(𝒙 + 𝟒)^𝟐 = C The curve passes through (−2, 1) Put x = −2 & y = 1 in (1) (1 + 3)/(−2 + 4)^2 = C C = 4/(2)^2 = 4/4 C = 1 Put value c = 1 in equation (1) (𝑦 + 3)/(𝑥 + 4)^2 = 1 y + 3 = (x + 4)2 Hence the equation of the curve is y + 3 = (x + 4)2