

Variable separation - Statement given
Last updated at Dec. 16, 2024 by Teachoo



Transcript
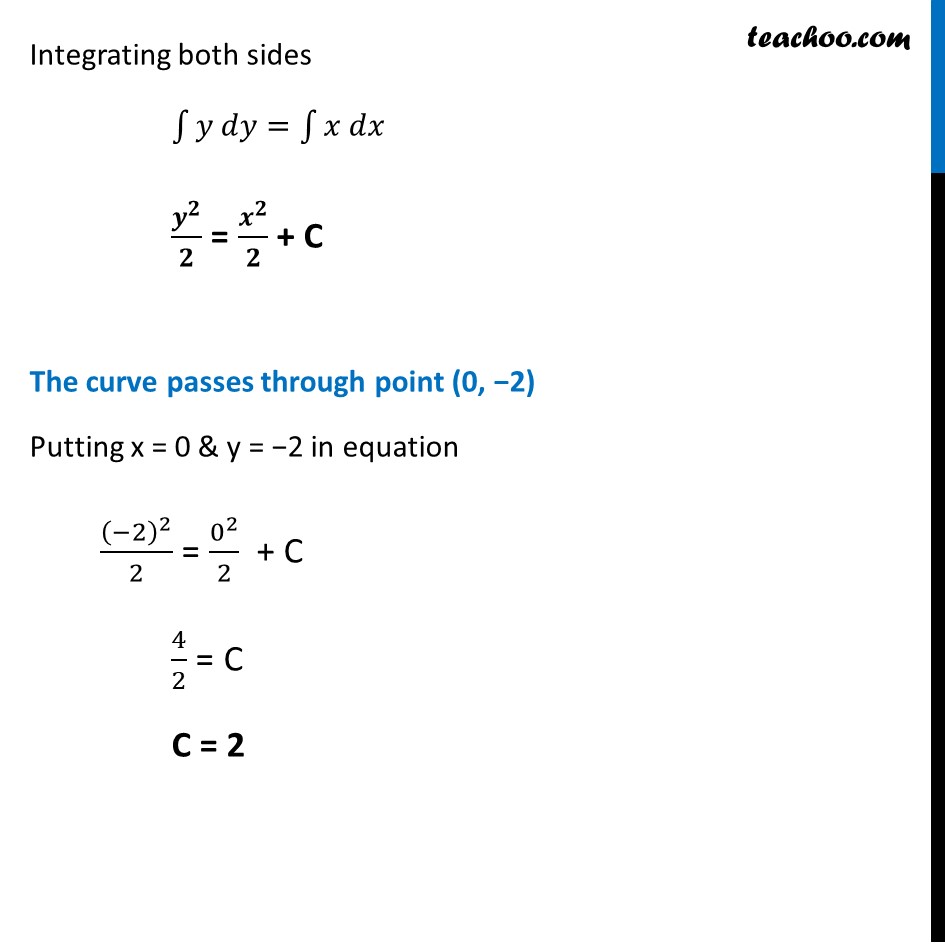
Ex 9.3, 17 Find the equation of a curve passing through the point (0 , −2) , given that at any point (𝑥 , 𝑦) on the curve , the product of the slope of its tangent and 𝑦 coordinate of the point is equal to the 𝑥 coordinate of the point .Slope of tangent to the curve = 𝒅𝒚/𝒅𝒙 Given at any point (x, y), product of slope of its tangent and y-coordinate is equal to x-coordinate of the point Therefore, y 𝒅𝒚/𝒅𝒙 = x y dy = x dx Integrating both sides ∫1▒〖𝑦 𝑑𝑦=∫1▒〖𝑥 𝑑𝑥 〗 〗 𝒚^𝟐/𝟐 = 𝒙^𝟐/𝟐 + C The curve passes through point (0, −2) Putting x = 0 & y = −2 in equation (−2)^2/2 = 0^2/2 + C 4/2 = C C = 2 Putting back value of C in equation 𝒚^𝟐/𝟐 = 𝒙^𝟐/𝟐 + 2 𝑦^2/2 = (𝑥^2 + 4)/2 𝑦^2 = 𝑥^2 + 4 𝒚^𝟐 − 𝒙^𝟐= 4 Hence, equation of the curve is 𝒚^𝟐 − 𝒙^𝟐= 4