Question 1 - Formation of Differntial equation when general solution given - Chapter 9 Class 12 Differential Equations

Last updated at Dec. 16, 2024 by

Slide109.JPG

Slide110.JPG

Go Ad-free

Transcript

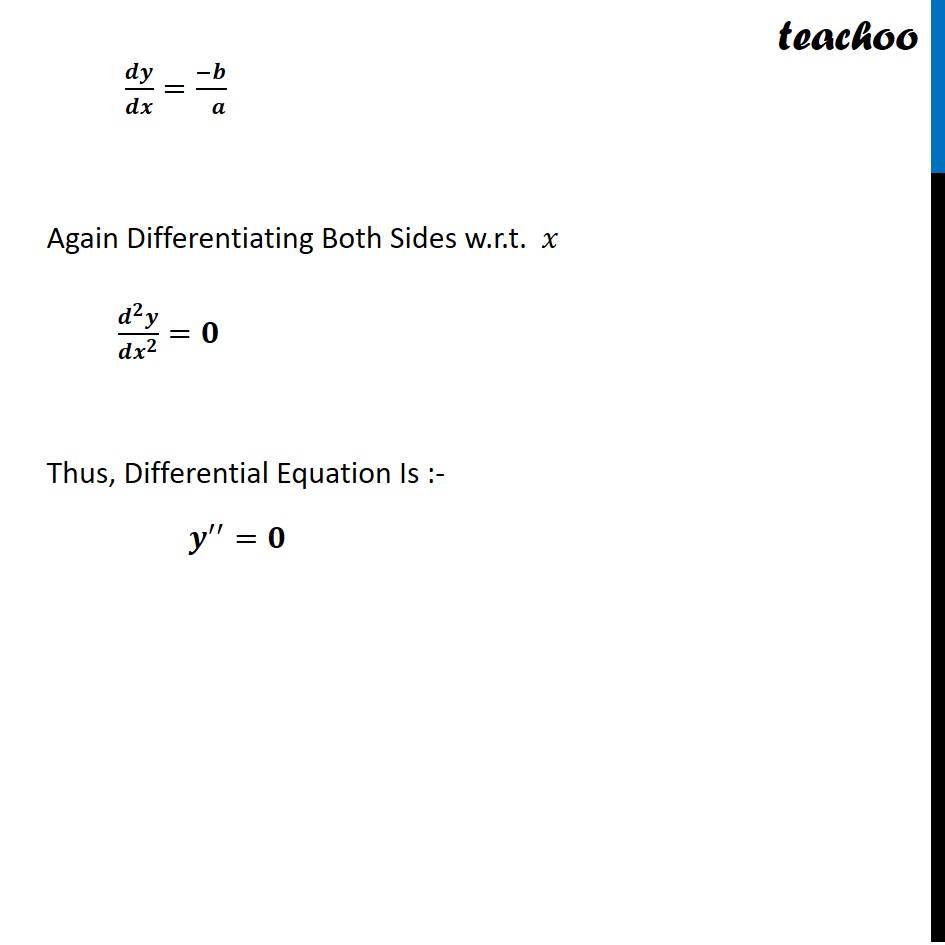
Question 1 Form a differential equation representing the given family of curves by eliminating arbitrary constants 𝑎 and 𝑏. 𝑥/𝑎+𝑦/𝑏=1 Here , we eliminate constants by Differentiating both sides w.r.t.x Now, 𝑥/𝑎+𝑦/𝑏=1 Differentiating Both Sides w.r.t. 𝑥 1/𝑎+1/𝑏.𝑑𝑦/𝑑𝑥=0 1/𝑏.𝑑𝑦/𝑑𝑥=(−1)/( 𝑎) The number of times we differentiate is equal to number of constants 𝒅𝒚/𝒅𝒙=(−𝒃)/( 𝒂) Again Differentiating Both Sides w.r.t. 𝑥 (𝒅^𝟐 𝒚)/(𝒅𝒙^𝟐 )=𝟎 Thus, Differential Equation Is :- 𝒚^′′=𝟎

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo