Misc 9 - Chapter 9 Class 12 Differential Equations (Important Question)

Transcript

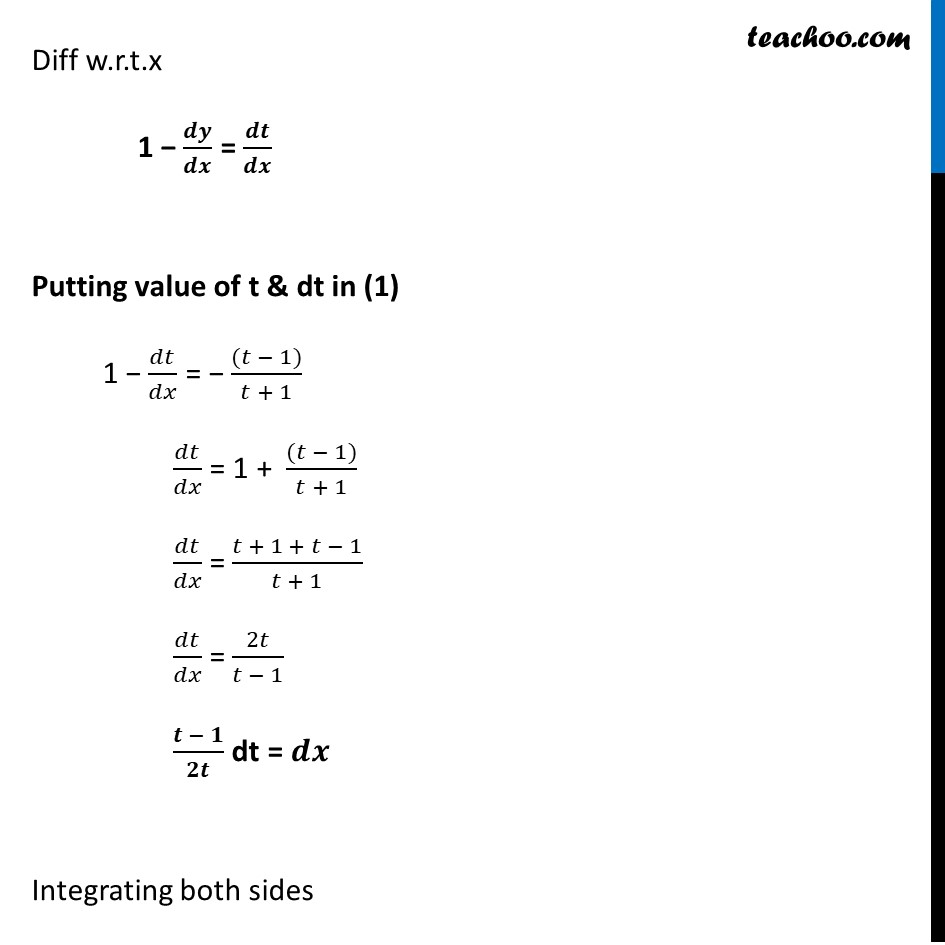
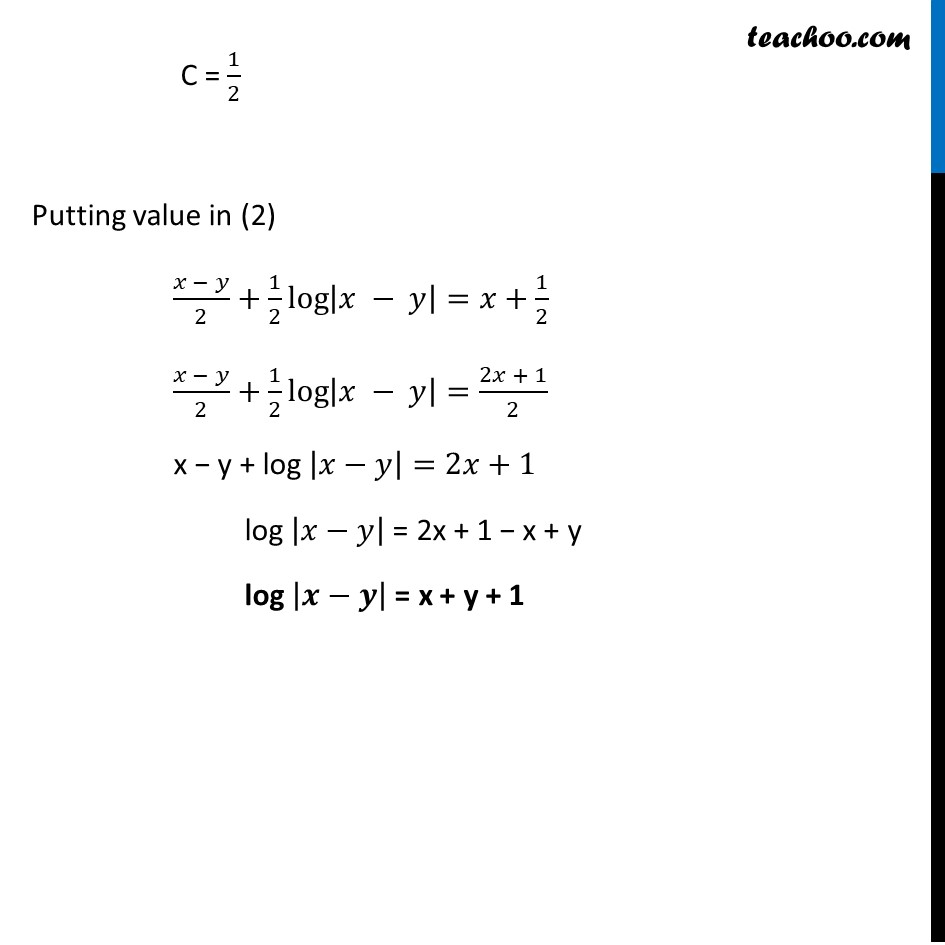
Misc 9 Find a particular solution of the differential equation (𝑥−𝑦)(𝑑𝑥+𝑑𝑦)=𝑑𝑥−𝑑𝑦 , given that 𝑦=−1 , when 𝑥=0 (𝐻𝑖𝑛𝑡:𝑝𝑢𝑡 𝑥−𝑦=𝑡) (𝑥−𝑦)(𝑑𝑥+𝑑𝑦)=𝑑𝑥−𝑑𝑦 𝑥𝑑𝑥 + 𝑥𝑑𝑦 − y dx − y dy = dx − dy x dx − y dx − dx = − xdy + y dy − dy (x − y − 1) dx = (−x + y − 1)dy 𝑑𝑦/𝑑𝑥 = (𝑥 − 𝑦 − 1)/(−𝑥 + 𝑦 − 1) 𝒅𝒚/𝒅𝒙 = ((𝒙 − 𝒚 − 𝟏))/(−(𝒙 −𝒚 + 𝟏)) Let x − y = t Diff w.r.t.x 1 − 𝒅𝒚/𝒅𝒙 = 𝒅𝒕/𝒅𝒙 Putting value of t & dt in (1) 1 − 𝑑𝑡/𝑑𝑥 = − ((𝑡 − 1))/(𝑡 + 1) 𝑑𝑡/𝑑𝑥 = 1 + ((𝑡 − 1))/(𝑡 + 1) 𝑑𝑡/𝑑𝑥 = (𝑡 + 1 + 𝑡 − 1)/(𝑡 + 1) 𝑑𝑡/𝑑𝑥 = 2𝑡/(𝑡 − 1) (𝒕 − 𝟏)/𝟐𝒕 dt = 𝒅𝒙 Integrating both sides ∫1▒〖(𝑡 + 1 )/2𝑡 𝑑𝑡〗 = ∫1▒𝑑𝑥 ∫1▒〖(𝑡/2𝑡+1/2𝑡) 𝑑𝑡〗 = x + c ∫1▒(1/2+1/2𝑡)𝑑𝑡 = x + c 𝒕/𝟐 + 𝟏/𝟐 log |𝒕| = x + c Putting value of t = x – y (𝑥 − 𝑦)/2 + 1/2 log |𝑥−𝑦| = x + C Given y = –1 when x = 0 Put x = 0 & y = −1 in (2) (0 + 1)/2 + 1/2 log 1 = 0 + C 1/2 = C C = 1/2 Putting value in (2) (𝑥 − 𝑦)/2+1/2 log⁡〖|𝑥 − 𝑦|=𝑥+1/2〗 (𝑥 − 𝑦)/2+1/2 log⁡〖|𝑥 − 𝑦|=(2𝑥 + 1)/2〗 x − y + log |𝑥−𝑦|=2𝑥+1 log |𝑥−𝑦| = 2x + 1 − x + y log |𝒙−𝒚| = x + y + 1