Example 16 - Chapter 9 Class 12 Differential Equations
Last updated at Dec. 16, 2024 by Teachoo
Solving Linear differential equations - Equation given
Ex 9.5, 18 (MCQ)
You are here
You are here
Chapter 9 Class 12 Differential Equations
Concept wise
- Order and Degree
- Gen and Particular Solution
- Formation of Differntial equation when general solution given
- Variable separation - Equation given
- Variable separation - Statement given
- Solving homogeneous differential equation
-
Solving Linear differential equations - Equation given
- Solving Linear differential equations - Statement given
Transcript
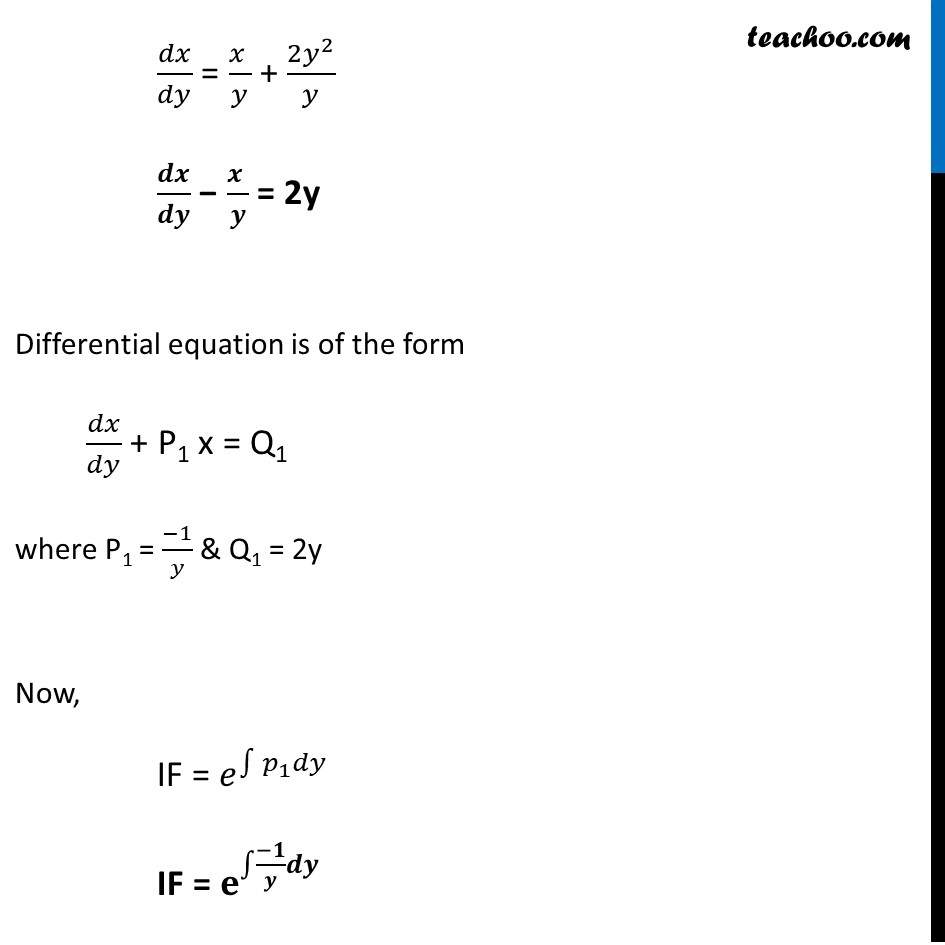
Example 16 Find the general solution of the differential equation π¦ ππ₯β(π₯+2π¦^2 )ππ¦=0 Given equation π¦ ππ₯β(π₯+2π¦^2 )ππ¦=0 π¦ ππ₯=(π₯+2π¦^2 )ππ¦ π π/π π = π/(π + ππ^π ) This is not of the form ππ¦/ππ₯+ππ¦=π β΄ We find π π/π π ππ₯/ππ¦ = (π₯ + 2π¦^2)/π¦ ππ₯/ππ¦ = (π₯ )/π¦ + (2π¦^2)/π¦ π π/π π β (π )/π = 2y Differential equation is of the form ππ₯/ππ¦ + P1 x = Q1 where P1 = (β1)/π¦ & Q1 = 2y Now, IF = π^β«1βγπ_1 ππ¦γ IF = π^β«1βγ(βπ)/π π π" " γ IF = e^(βlogβ‘π¦ ) IF = e^logβ‘(1/π¦) IF = π/π Solution is x(IF) = β«1βγ(πΈΓπ°π)π π+πͺ γ x Γ 1/π¦=β«1βγ2π¦Γ1/π¦γ ππ¦+πΆ π/π = β«1βγππ π+πͺγ π₯/π¦ = 2π¦+πΆ x = y (2y + C) x = 2y2 + Cy
