


Variable separation - Statement given
Last updated at December 16, 2024 by Teachoo





Transcript
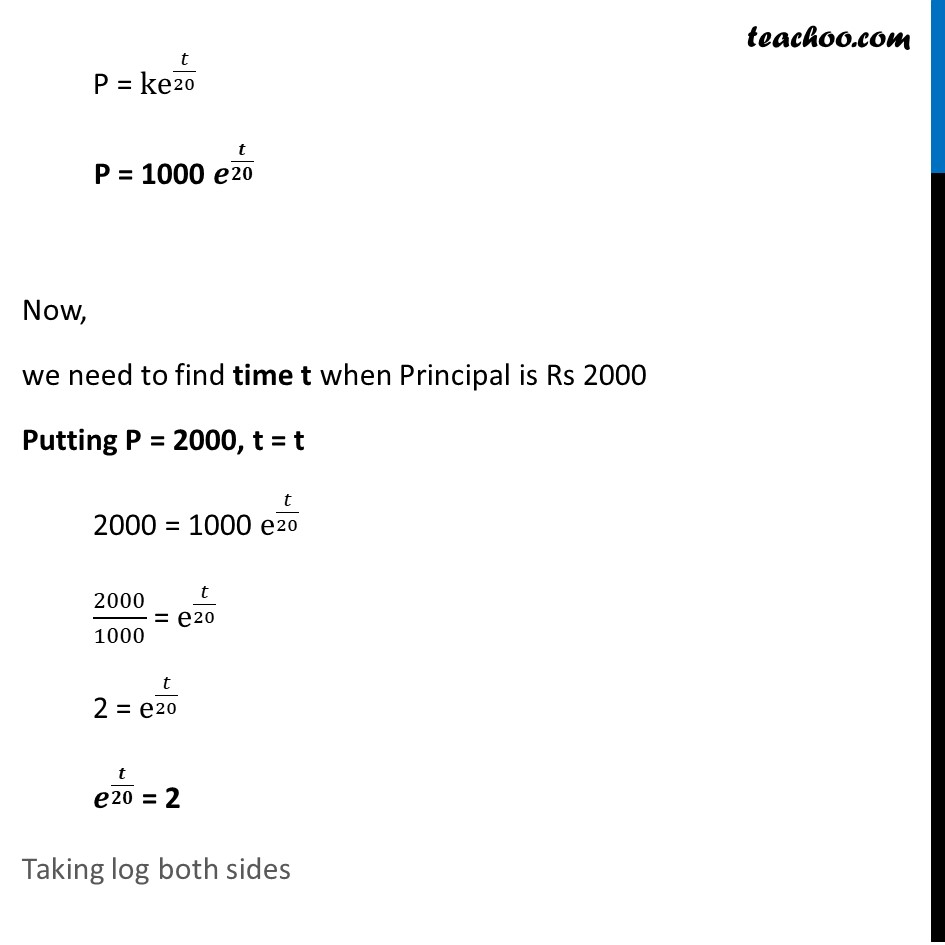
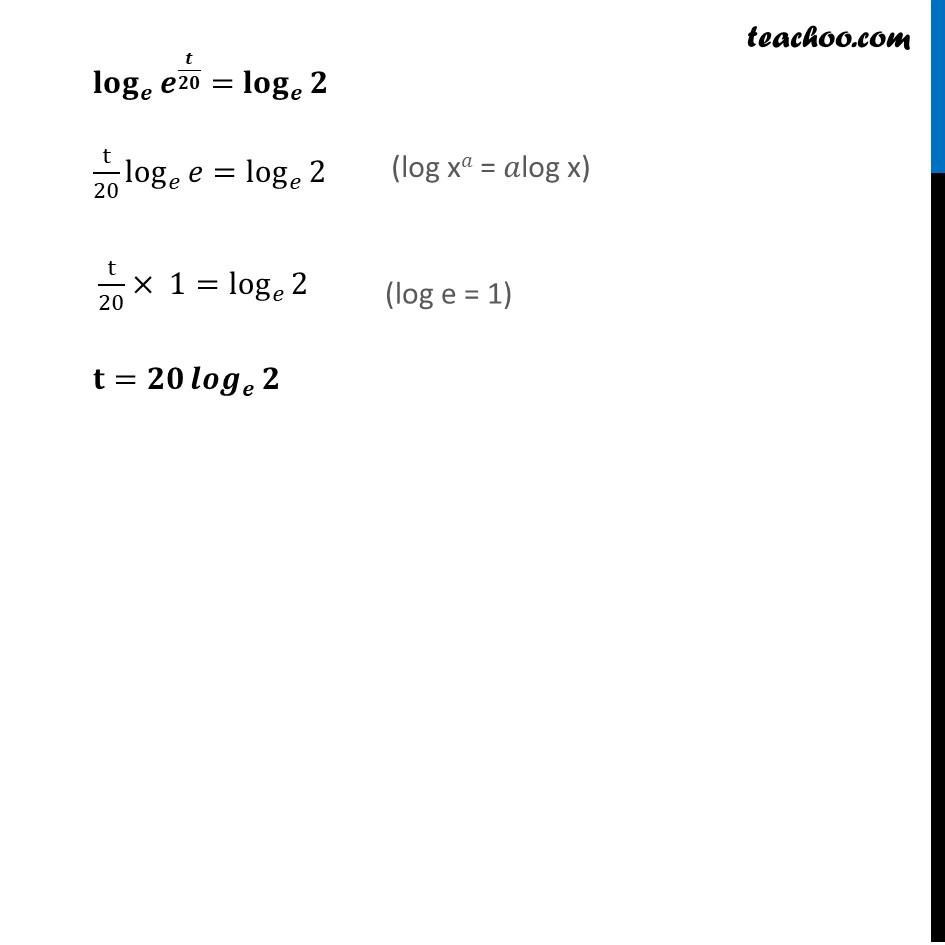
Example 9 In a bank, principal increases continuously at the rate of 5% per year. In how many years Rs 1000 double itself ?Rate of change of principal = 5% of Principal 𝒅𝑷/𝒅𝒕 = 5% × P 𝑑𝑃/𝑑𝑡 = 5/100 × P 𝑑𝑃/𝑑𝑡 = 1/20 × P 𝒅𝒑/𝑷 = 𝒅𝒕/𝟐𝟎 Integrating both sides ∫1▒𝑑𝑝/𝑃 = ∫1▒𝑑𝑡/20 log |𝑷| = 𝒕/𝟐𝟎 + C Removing log P = e^(𝑡/20 + 𝐶) × ec P = e^(𝑡/20 + 𝐶) × ec P = k𝒆^(𝒕/𝟐𝟎) where k = ec Now, we have to find in how many years Rs 1000 double it self Thus, we need to find time T when Principal is Rs 2000 First let us find k At t = 0, P = 1000 Putting in (1) P = ke^(𝑡/20) 1000 = k𝒆^(𝟎/𝟐𝟎) 1000 = k e0 1000 = k × 1 1000 = k So, k = 1000 Put k = 1000 in (1) P = ke^(𝑡/20) P = 1000 𝒆^(𝒕/𝟐𝟎) Now, we need to find time t when Principal is Rs 2000 Putting P = 2000, t = t 2000 = 1000 e^(𝑡/20) 2000/1000 = e^(𝑡/20) 2 = e^(𝑡/20) 𝒆^(𝒕/𝟐𝟎) = 2 Taking log both sides 〖𝐥𝐨𝐠〗_𝒆〖𝒆^(𝒕/𝟐𝟎) 〗=〖𝐥𝐨𝐠〗_𝒆𝟐 t/20 log_𝑒𝑒=log_𝑒2 " " t/20× 1=log_𝑒2 𝐭=𝟐𝟎 〖𝒍𝒐𝒈〗_𝒆𝟐