Ex 9.5, 4 - Chapter 9 Class 12 Differential Equations
Last updated at Dec. 16, 2024 by Teachoo
Solving Linear differential equations - Equation given
Ex 9.5, 18 (MCQ)
You are here
You are here
Chapter 9 Class 12 Differential Equations
Concept wise
- Order and Degree
- Gen and Particular Solution
- Formation of Differntial equation when general solution given
- Variable separation - Equation given
- Variable separation - Statement given
- Solving homogeneous differential equation
-
Solving Linear differential equations - Equation given
- Solving Linear differential equations - Statement given
Transcript
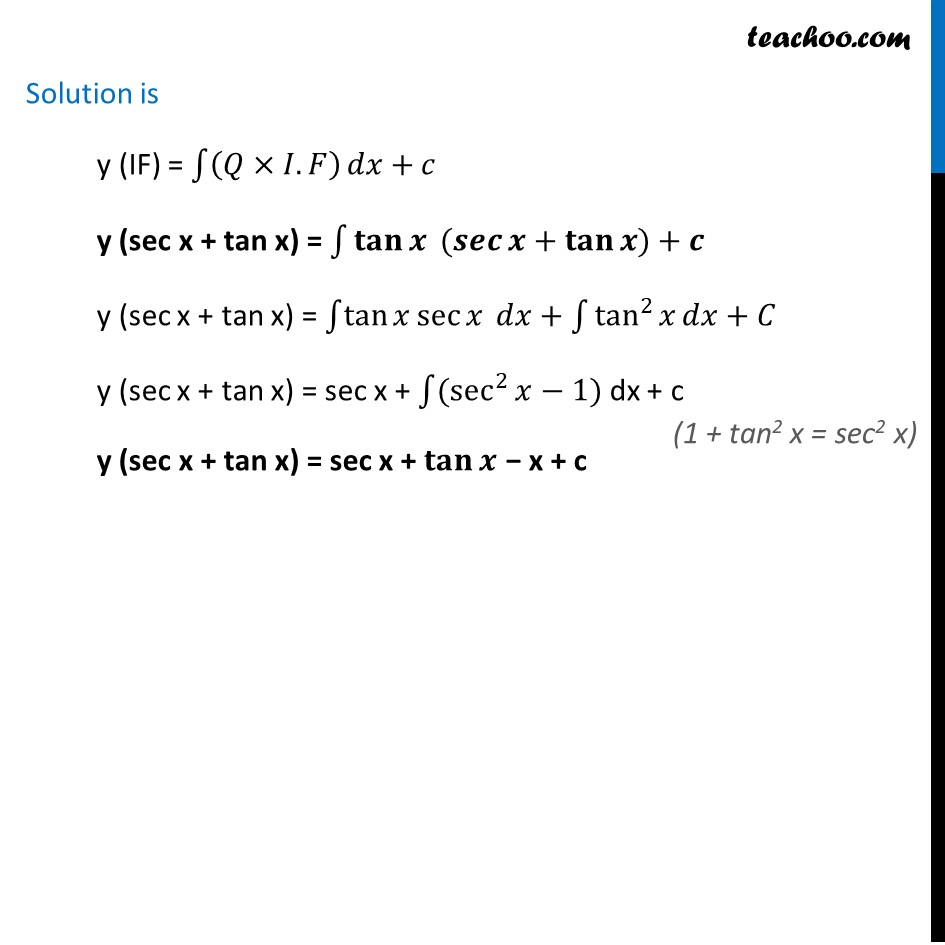
Ex 9.5, 4 For each of the differential equation given in Exercises 1 to 12, find the general solution : ππ¦/ππ₯+(secβ‘π₯ )π¦=π‘πππ₯(0β€π₯<π/2) Differential equation is of the form ππ¦/ππ₯ + Py = Q where P = sec x and Q = tan x Finding integrating factor, IF = π^β«1βγπ ππ₯γ IF = e^β«1βsecβ‘γπ₯ ππ₯γ IF = γe^πππγ^|secβ‘γπ₯ + tanβ‘π₯ γ | I.F = sec x + tan x Solution is y (IF) = β«1β(πΓπΌ.πΉ) ππ₯+π y (sec x + tan x) = β«1βγπππ§β‘π (πππβ‘π+πππ§β‘γπ)γ γ+π y (sec x + tan x) = β«1βγtanβ‘π₯ secβ‘π₯ γ ππ₯+β«1βγtan^2β‘π₯ ππ₯+πΆγ y (sec x + tan x) = sec x + β«1βγ(sec^2β‘π₯β1)γ dx + c y (sec x + tan x) = sec x + πππ§β‘π β x + c
