
Solving homogeneous differential equation
Solving homogeneous differential equation
Last updated at Dec. 16, 2024 by Teachoo




Transcript
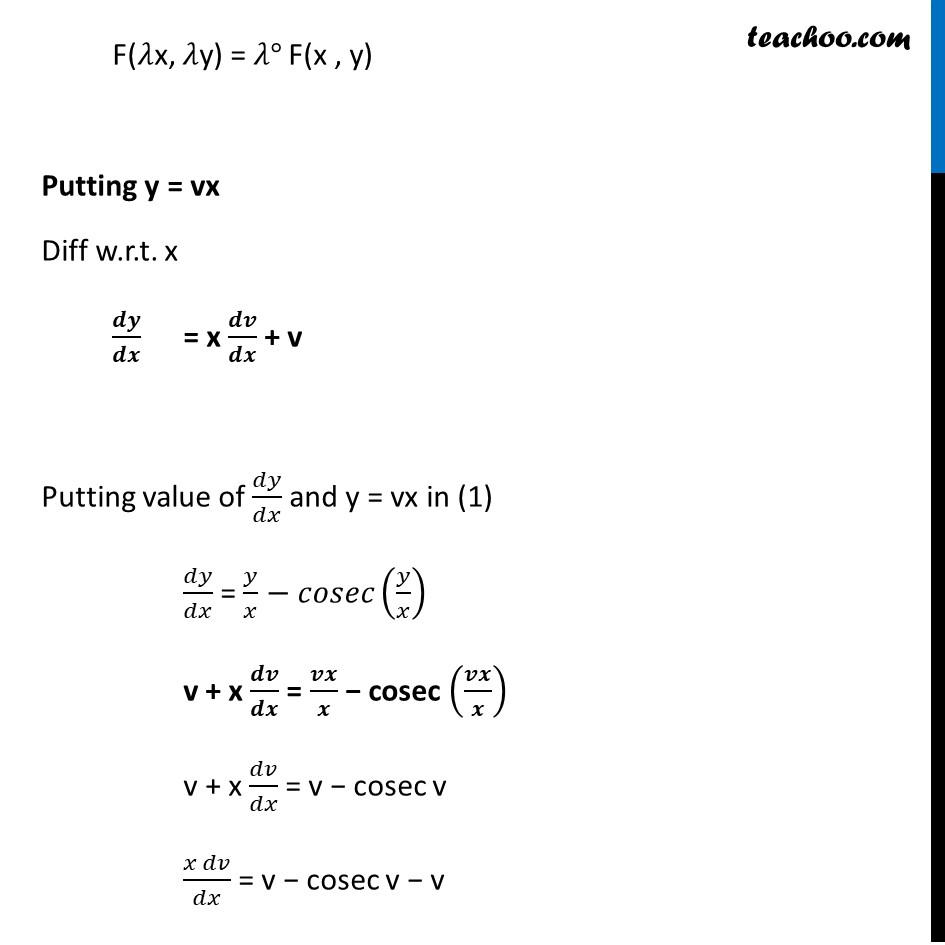
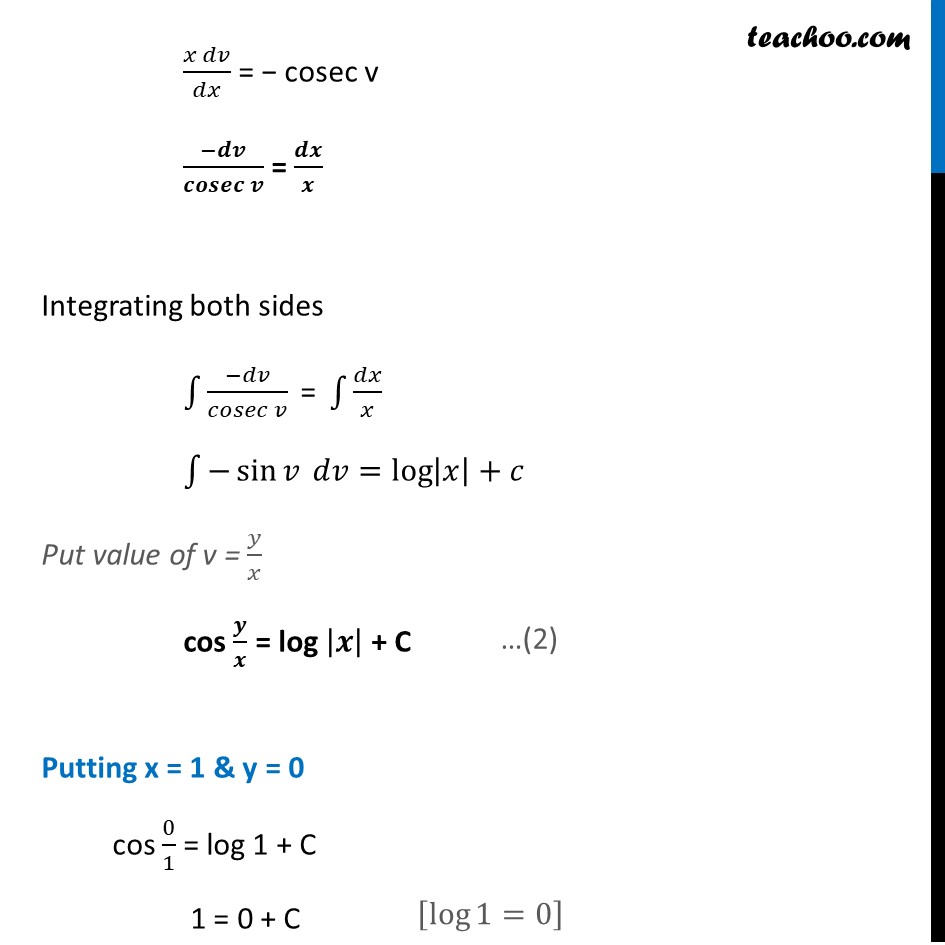
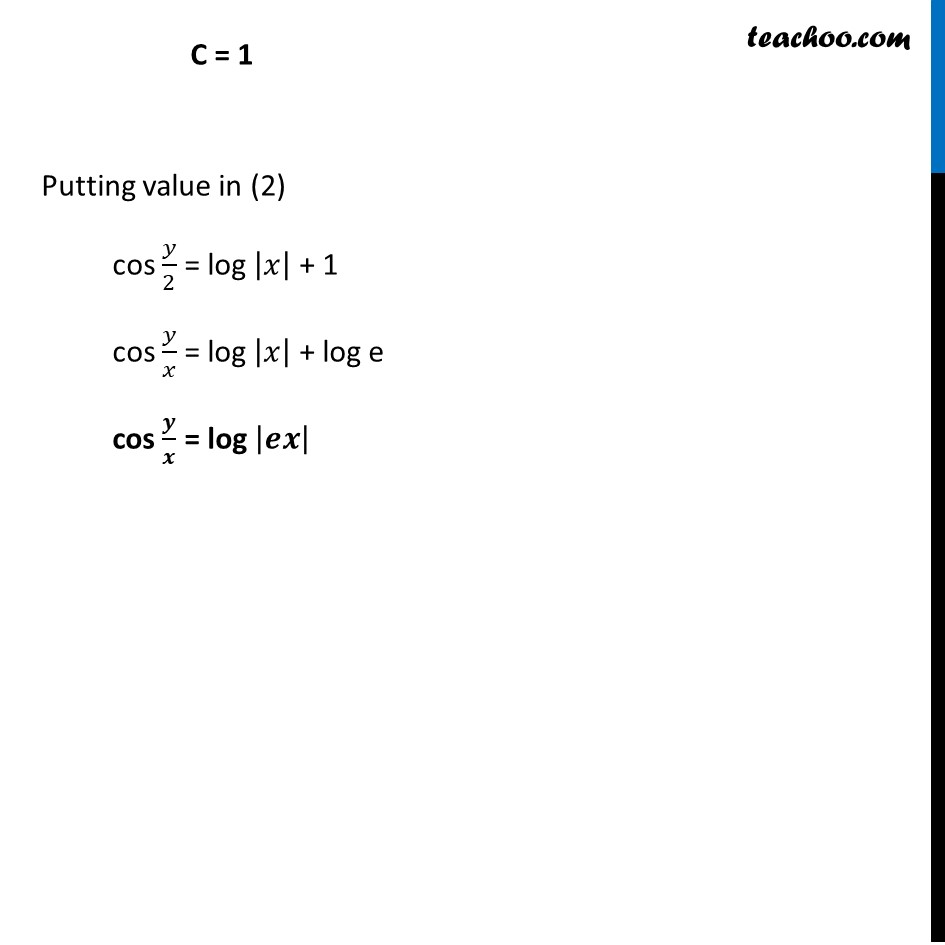
Ex 9.4, 14 For each of the differential equations in Exercises from 11 to 15 , find the particular solution satisfying the given condition : 𝑑𝑦/𝑑𝑥−𝑦/𝑥+𝑐𝑜𝑠𝑒𝑐(𝑦/𝑥)=0;𝑦=0 When 𝑥=1 Differential equation is 𝑑𝑦/𝑑𝑥 = 𝑦/𝑥−𝑐𝑜𝑠𝑒𝑐(𝑦/𝑥) Let F(x, y) = 𝑑𝑦/𝑑𝑥 = 𝑦/𝑥−𝑐𝑜𝑠𝑒𝑐(𝑦/𝑥) Finding F(𝝀x, 𝝀y) F(𝜆x, 𝜆y) = ("𝜆" 𝑦)/("𝜆" 𝑥)−𝑐𝑜𝑠𝑒𝑐(("𝜆" 𝑦)/("𝜆" 𝑥)) = 𝑦/𝑥 − cosec (𝑦/𝑥) = 𝜆° F(x, y) ∴ F(x, y) is 𝑎 homogenous function of degree zero F(𝜆x, 𝜆y) = 𝜆° F(x , y) Putting y = vx Diff w.r.t. x 𝒅𝒚/𝒅𝒙 = x 𝒅𝒗/𝒅𝒙 + v Putting value of 𝑑𝑦/𝑑𝑥 and y = vx in (1) 𝑑𝑦/𝑑𝑥 = 𝑦/𝑥−𝑐𝑜𝑠𝑒𝑐(𝑦/𝑥) v + x 𝒅𝒗/𝒅𝒙 = 𝒗𝒙/𝒙 − cosec (𝒗𝒙/𝒙) v + x 𝑑𝑣/𝑑𝑥 = v − cosec v (𝑥 𝑑𝑣)/𝑑𝑥 = v − cosec v − v (𝑥 𝑑𝑣)/𝑑𝑥 = − cosec v (−𝒅𝒗)/(𝒄𝒐𝒔𝒆𝒄 𝒗) = 𝒅𝒙/𝒙 Integrating both sides ∫1▒〖(−𝑑𝑣)/(𝑐𝑜𝑠𝑒𝑐 𝑣) " = " ∫1▒𝑑𝑥/𝑥〗 ∫1▒〖−sin𝑣 𝑑𝑣〗=log〖|𝑥|+𝑐〗 Put value of v = 𝑦/𝑥 cos 𝒚/𝒙 = log |𝒙| + C Putting x = 1 & y = 0 cos 0/1 = log 1 + C 1 = 0 + C C = 1 Putting value in (2) cos 𝑦/2 = log |𝑥| + 1 cos 𝑦/𝑥 = log |𝑥| + log e cos 𝒚/𝒙 = log |𝒆𝒙|