You are here
Question 1 (a) - Finding approximate value of numbers - Chapter 6 Class 12 Application of Derivatives
Last updated at Dec. 16, 2024 by Teachoo
Finding approximate value of numbers
Chapter 6 Class 12 Application of Derivatives
Concept wise
- Finding rate of change
- To show increasing/decreasing in whole domain
- To show increasing/decreasing in intervals
- Find intervals of increasing/decreasing
- Finding slope of tangent/normal
- Finding point when tangent is parallel/ perpendicular
- Finding equation of tangent/normal when point and curve is given
- Finding equation of tangent/normal when slope and curve are given
-
Finding approximate value of numbers
- Finding approximate value of function
- Finding approximate value- Statement questions
- Finding minimum and maximum values from graph
- Local maxima and minima
- Minima/ maxima (statement questions) - Number questions
- Minima/ maxima (statement questions) - Geometry questions
- Absolute minima/maxima
Transcript
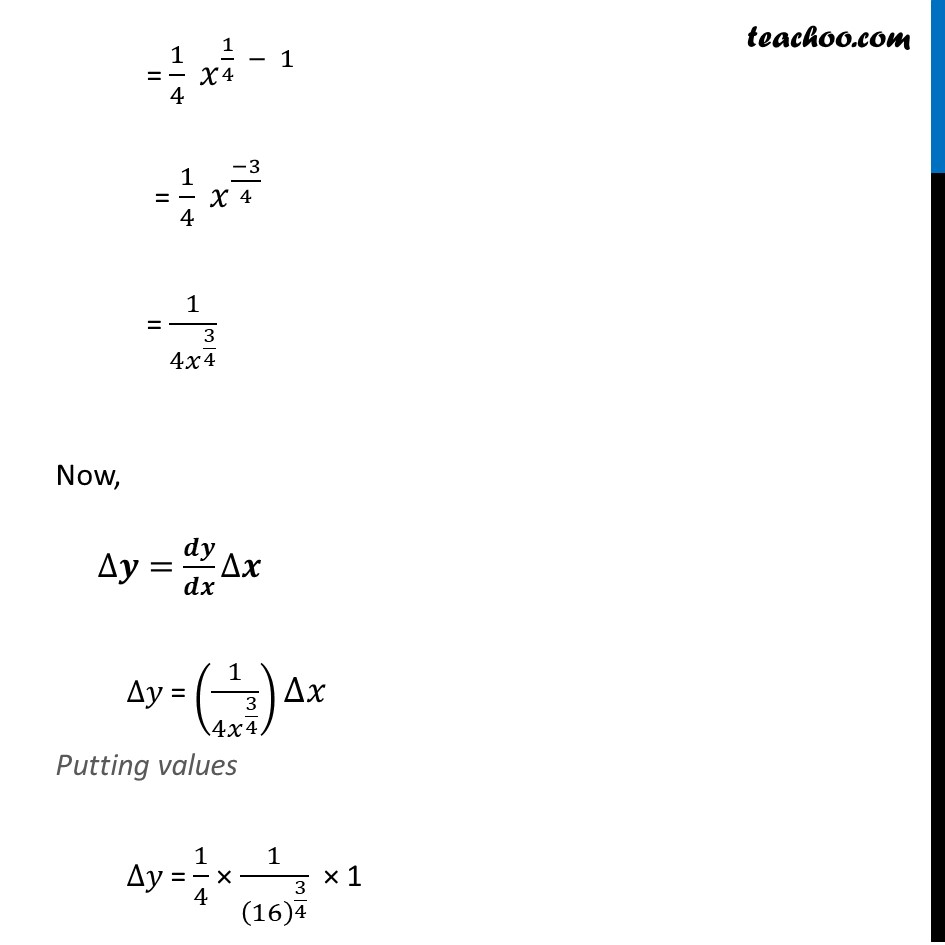
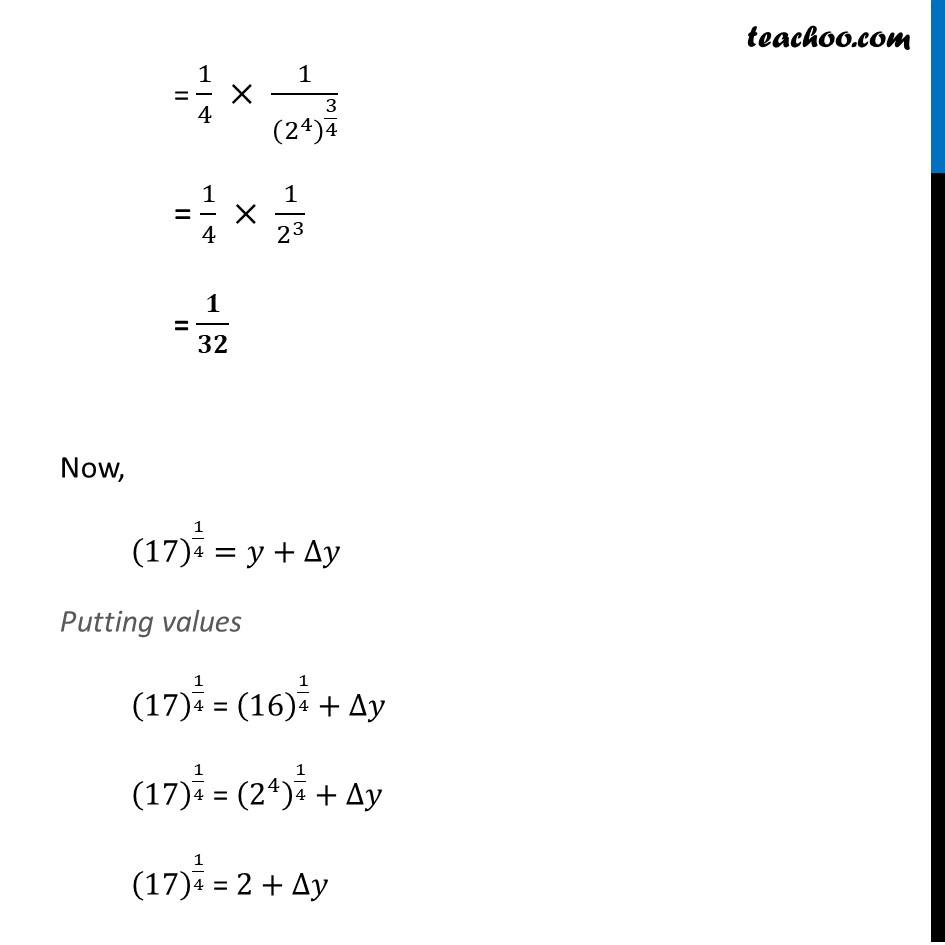
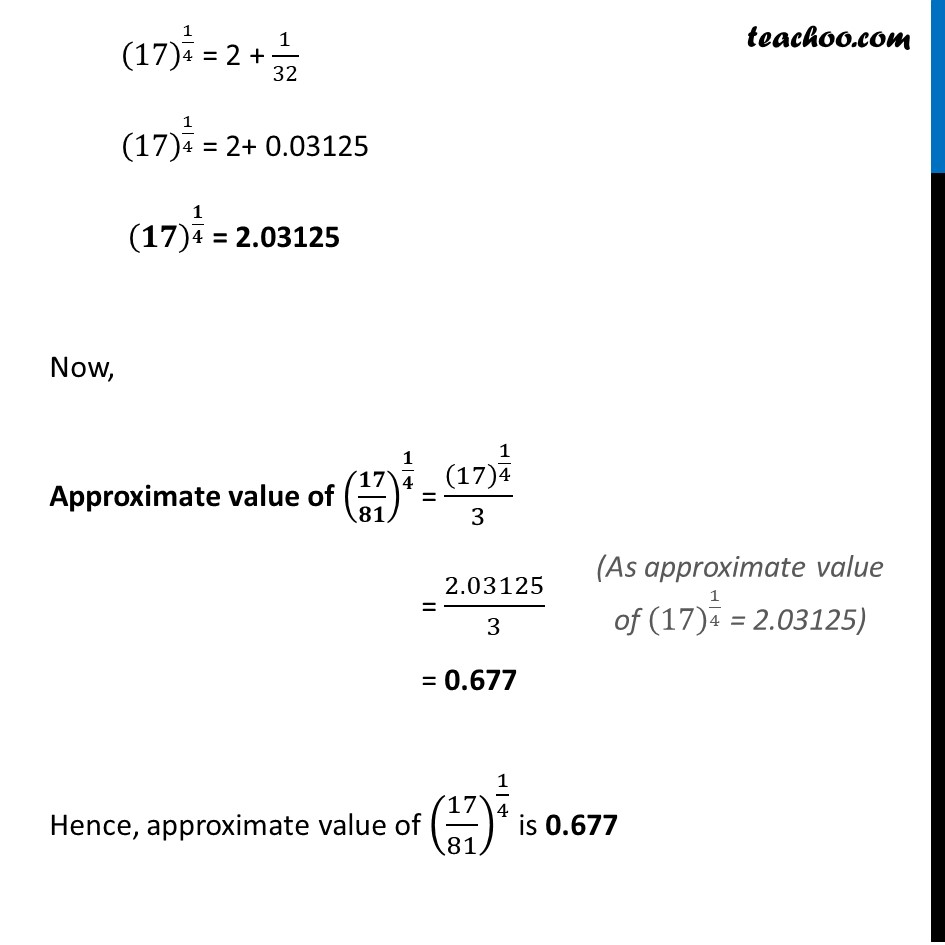
Misc 1 Using differentials, find the approximate value of each of the following: (a) (17/81)^(1/4) (17/81)^(1/4) = (17)^(1/4)/(81)^(1/4) = (17)^(1/4)/3 Let 𝑦 =𝑥^(1/4) Where 𝑥=16 and △𝑥=1 Since 𝒚 =𝒙^(𝟏/𝟒) 𝑑𝑦/𝑑𝑥=𝑑(𝑥^(1/4) )/𝑑𝑥 = 1/4 𝑥^(1/4 − 1) = 1/4 𝑥^((−3)/4) = 1/(4𝑥^(3/4) ) Now, ∆𝒚=𝒅𝒚/𝒅𝒙 ∆𝒙 ∆𝑦 = (1/(4𝑥^(3/4) ))∆𝑥 Putting values ∆𝑦 = 1/4 × 1/(16)^(3/4) × 1 = 1/4 × 1/(2^4 )^(3/4) = 1/4 × 1/2^3 = 𝟏/𝟑𝟐 Now, (17)^(1/4)=𝑦+∆𝑦 Putting values (17)^(1/4) = (16)^(1/4)+∆𝑦 (17)^(1/4) = (2^4 )^(1/4)+∆𝑦 (17)^(1/4) = 2+∆𝑦 (17)^(1/4) = 2 + 1/32 (17)^(1/4) = 2+ 0.03125 (𝟏𝟕)^(𝟏/𝟒) = 2.03125 Now, Approximate value of (𝟏𝟕/𝟖𝟏)^(𝟏/𝟒) = (17)^(1/4)/3 = 2.03125/3 = 0.677 Hence, approximate value of (17/81)^(1/4) is 0.677 (As approximate value of (17)^(1/4) = 2.03125)
