Maths Crash Course - Live lectures + all videos + Real time Doubt solving!
Example 48 - Chapter 6 Class 12 Application of Derivatives (Term 1)
Last updated at April 19, 2021 by Teachoo
Examples
Example 1
Example 2
Example 3
Example 4 Important
Example 5
Example 6
Example 7
Example 8 Important
Example 9 Important
Example 10
Example 11 Important
Example 12
Example 13 Important
Example 14 Deleted for CBSE Board 2023 Exams
Example 15 Deleted for CBSE Board 2023 Exams
Example 16 Deleted for CBSE Board 2023 Exams
Example 17 Important Deleted for CBSE Board 2023 Exams
Example 18 Deleted for CBSE Board 2023 Exams
Example 19 Deleted for CBSE Board 2023 Exams
Example 20 Deleted for CBSE Board 2023 Exams
Example 21 Deleted for CBSE Board 2023 Exams
Example 22 Deleted for CBSE Board 2023 Exams
Example 23 Deleted for CBSE Board 2023 Exams
Example 24
Example 25
Example 26
Example 27
Example 28 Important
Example 29
Example 30 Important
Example 31
Example 32 Important
Example 33 Important
Example 34
Example 35 Important
Example 36
Example 37 Important
Example 38 Important
Example 39
Example 40 Important
Example 41 Important
Example 42 Important
Example 43 Important
Example 44 Important
Example 45 Important Deleted for CBSE Board 2023 Exams
Example 46 Important Deleted for CBSE Board 2023 Exams
Example 47 Important
Example 48 Important You are here
Example 49
Example 50 Important
Example 51
Chapter 6 Class 12 Application of Derivatives
Serial order wise
Transcript
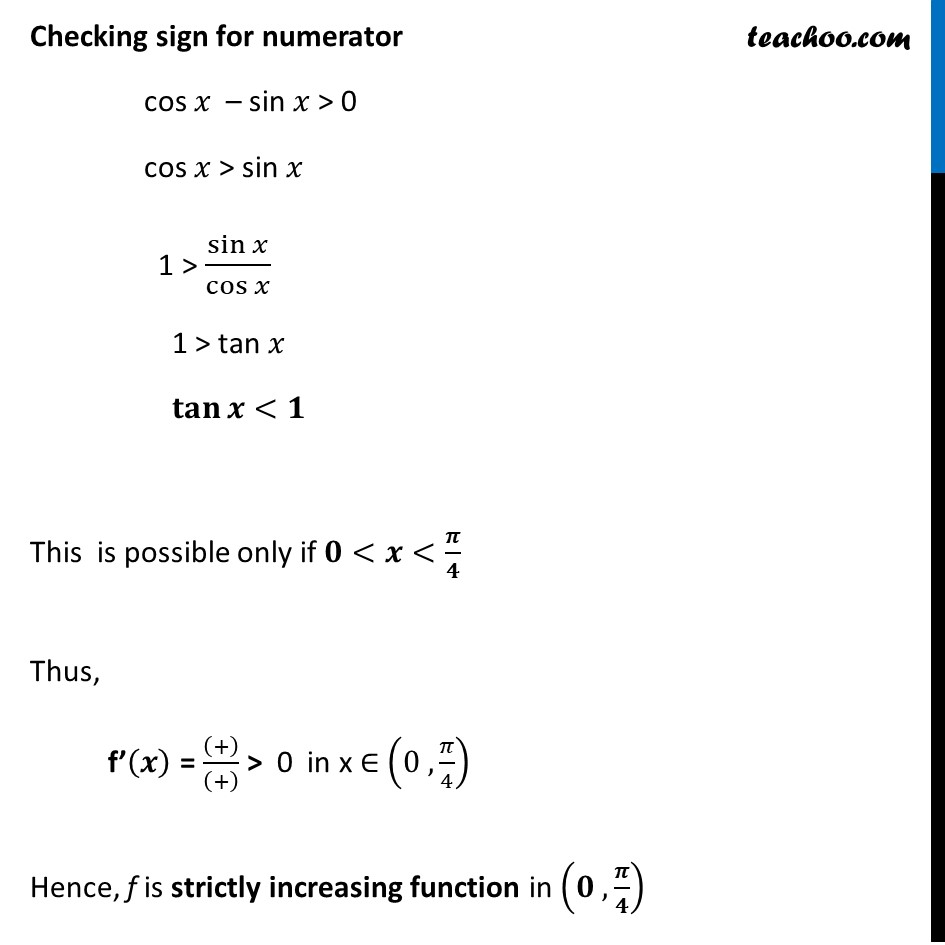
Example 48 Show that the function f given by f (x) = tan–1(sin x + cos x), x > 0 is always an strictly increasing function in (0,𝜋/4) f(𝑥)=tan^(−1)(sin𝑥+cos𝑥 ) Finding f’(𝒙) f’(𝑥) = (𝑑(tan^(−1)〖(sin𝑥 +cos𝑥 ))〗)/𝑑𝑥 = 1/(1 + (sin〖𝑥 + cos𝑥 〗 )^2 ) × 𝑑(sin〖𝑥 + cos𝑥 〗 )/𝑑𝑥 = 1/(1 + (〖〖𝐬𝐢𝐧〗^𝟐 𝒙〗〖+〖𝐜𝐨𝐬〗^𝟐〖𝒙 〗+ 2 sin𝑥 cos𝑥 〗 ) ) × (cos𝑥−sin𝑥 ) = 1/(1 + (𝟏 + 2 sin𝑥 cos𝑥 ) ) × (cos𝑥−sin𝑥 ) = 1/(2 + 2 sin𝑥 cos𝑥 ) × (cos𝑥−sin𝑥 ) = 𝐜𝐨𝐬〖𝒙 −〖 𝐬𝐢𝐧〗𝒙 〗/(𝟐 + 𝐬𝐢𝐧𝟐𝒙 ) For increasing, f’(x) > 0. ∴ Numerator and denominator both must be > 0 Checking sign for denominator Since Hence, denominator is always positive for 0 < x < 𝝅/𝟒 Checking sign for numerator cos 𝑥 – sin 𝑥 > 0 cos 𝑥 > sin 𝑥 1 > sin𝑥/cos𝑥 1 > tan 𝑥 𝐭𝐚𝐧𝒙<𝟏 This is possible only if 𝟎<𝒙<𝝅/𝟒 Thus, f’(𝒙) = ((+))/((+) ) > 0 in x ∈ (0 , 𝜋/4) Hence, f is strictly increasing function in (𝟎 , 𝝅/𝟒)
