Example 11 - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

  Slide33.JPG

Slide34.JPG
Slide35.JPG

Go Ad-free

Transcript

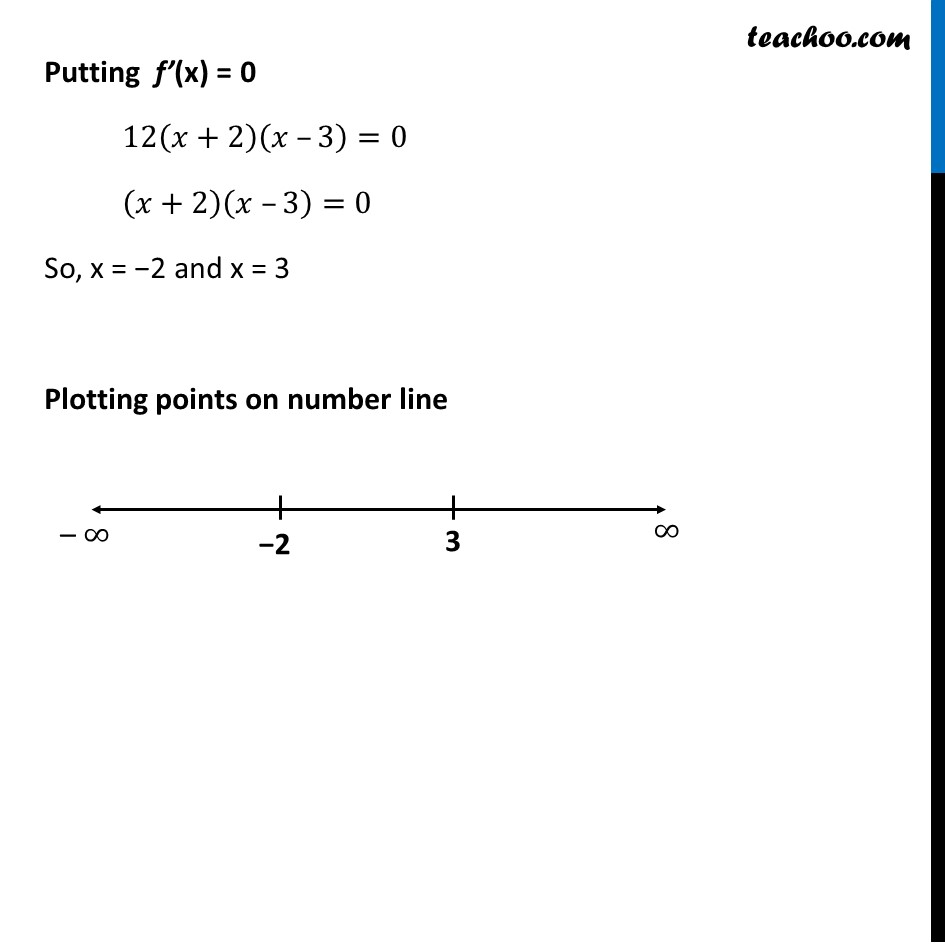
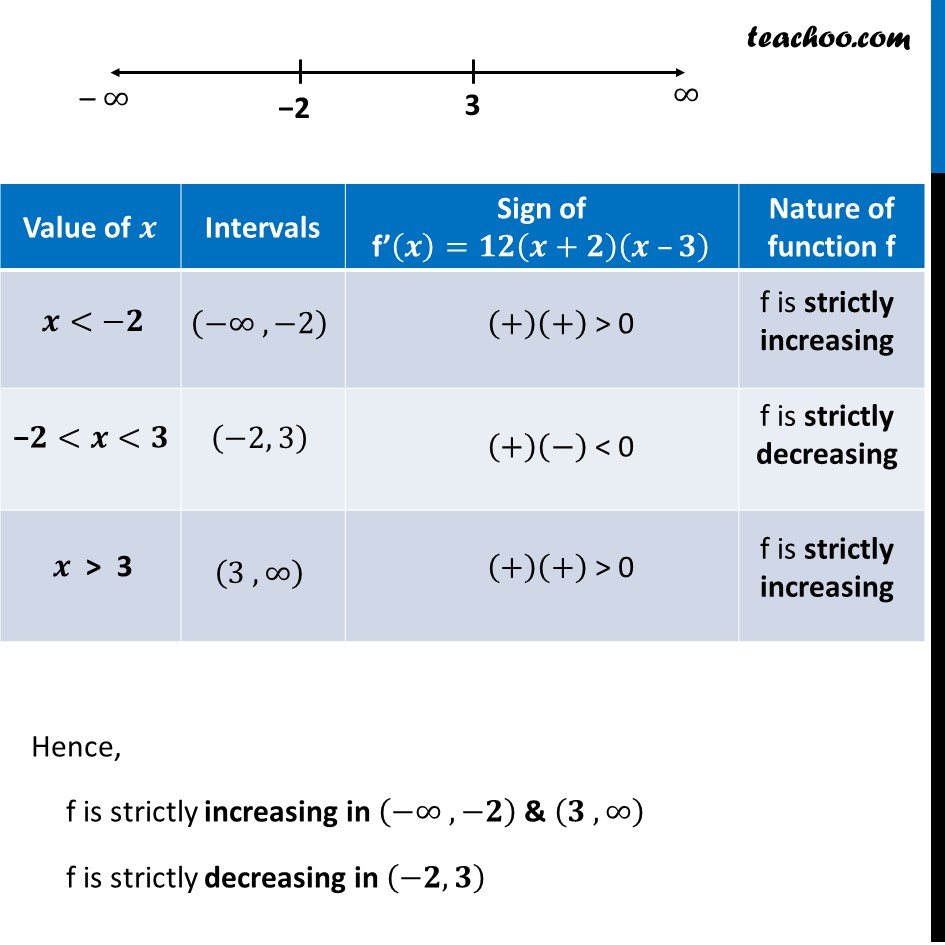
Example 11 Find the intervals in which the function f given by f (𝑥)=4𝑥3−6𝑥2 –72𝑥+30 is (a) strictly increasing (b) strictly decreasing. f (𝑥)=4𝑥3−6𝑥2 –72𝑥+30 Calculating f’(x) f (𝑥)=4𝑥3−6𝑥2–72𝑥+30 𝑓′(𝑥)=12𝑥2−12𝑥 –72𝑥 𝑓′(𝑥) =12(𝑥2−𝑥 – 6) 𝑓′(𝑥) =12(𝑥2−3𝑥+2𝑥 –6) 𝑓′(𝑥) = 12(𝑥(𝑥 − 3) + 2(𝑥 − 3)) 𝒇′(𝒙)=𝟏𝟐(𝒙+𝟐)(𝒙 –𝟑) Putting f’(x) = 0 12(𝑥+2)(𝑥 –3)=0 (𝑥+2)(𝑥 –3)=0 So, x = −2 and x = 3 Plotting points on number line Hence, f is strictly increasing in (−∞ ,−𝟐) & (𝟑 ,∞) f is strictly decreasing in (−𝟐, 𝟑)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo