






Finding minimum and maximum values from graph
Finding minimum and maximum values from graph
Last updated at Feb. 20, 2025 by Teachoo








Transcript
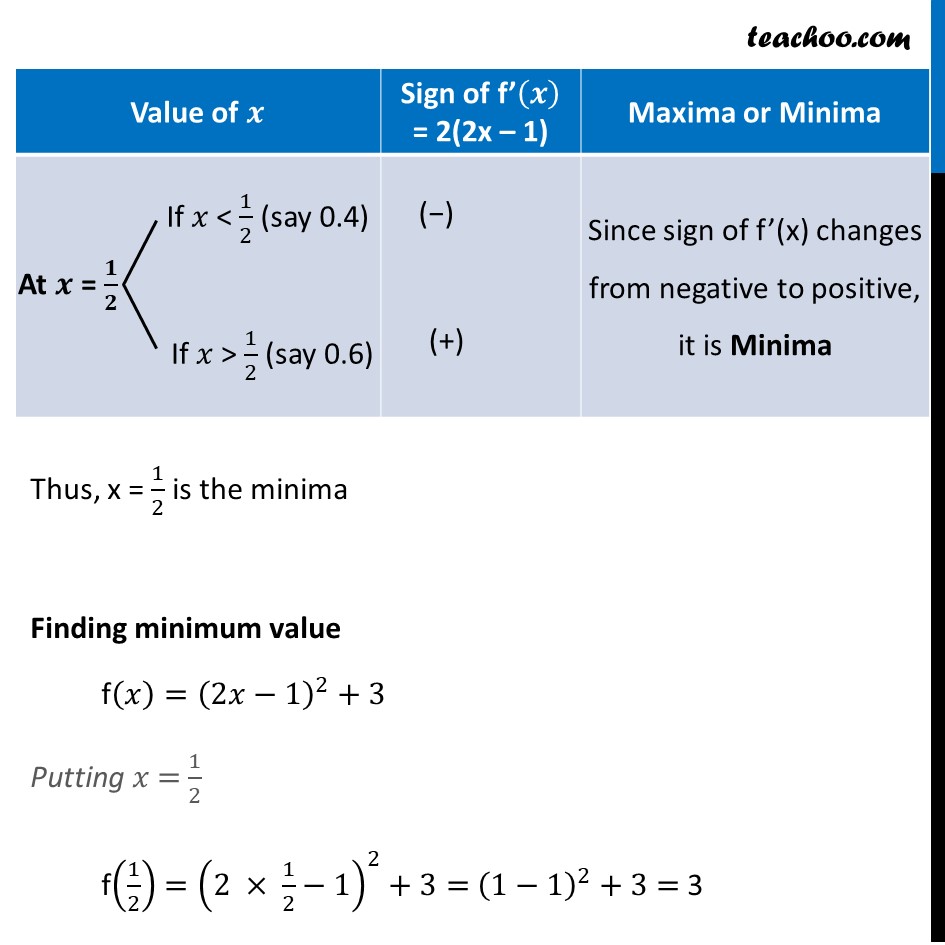
Ex 6.3, 1 (Method 1) Find the maximum and minimum values, if any, of the following functions given by (i) f (𝑥) = (2𝑥 – 1)^2 + 3 Square of number cant be negative It can be 0 or greater than 0 𝑓(𝑥)=(2𝑥−1)^2+3 Hence, Minimum value of (2𝑥−1)^2 = 0 Minimum value of (2𝑥−1^2 )+3 = 0 + 3 = 3 Also, there is no maximum value of 𝑥 ∴ There is no maximum value of f(x) Ex 6.3, 1 (Method 2) Find the maximum and minimum values, if any, of the following functions given by (i) f (𝑥) = (2𝑥 – 1)^2+3Finding f’(x) f(𝑥)=(2𝑥−1)^2+3 f’(𝑥)= 2(2𝑥−1) Putting f’(𝒙)=𝟎 2(2𝑥−1)=0 2𝑥 – 1 = 0 2𝑥 = 1 𝒙 = 𝟏/𝟐 Thus, x = 1/2 is the minima Finding minimum value f(𝑥)=(2𝑥−1)^2+3 Putting 𝑥 = 1/2 f(1/2)=(2 × 1/2−1)^2+3= (1−1)^2+3= 3 ∴ Minimum value = 3 There is no maximum value Ex 6.3, 1 (Method 3) Find the maximum and minimum values, if any, of the following functions given by (i) 𝑓 (𝑥)= (2𝑥 – 1)^2 + 3Double Derivative Test f(𝑥)=(2𝑥−1)^2+3 Finding f’(𝒙) f’(𝑥)=2(2𝑥−1)^(2−1) = 2(2𝑥−1) Putting f’(𝒙)=𝟎 2(2𝑥−1)=0 (2𝑥−1)=0 2𝑥 = 0 + 1 𝒙 = 𝟏/𝟐 Finding f’’(𝒙) f’(𝑥)=2(2𝑥−1) f’(𝑥) = 4𝑥 – 2 f’’(𝑥)= 4 f’’ (𝟏/𝟐) = 4 Since f’’ (𝟏/𝟐) > 0 , 𝑥 = 1/2 is point of local minima Putting 𝑥 = 1/2 , we can calculate minimum value f(𝑥) = (2𝑥−1)^2+3 f(1/2)= (2(1/2)−1)^2+3= (1−1)^2+3= 3 Hence, Minimum value = 3 There is no Maximum value