You are here
Question 1 (i) - Finding approximate value of numbers - Chapter 6 Class 12 Application of Derivatives
Last updated at Dec. 16, 2024 by Teachoo
Finding approximate value of numbers
Chapter 6 Class 12 Application of Derivatives
Concept wise
- Finding rate of change
- To show increasing/decreasing in whole domain
- To show increasing/decreasing in intervals
- Find intervals of increasing/decreasing
- Finding slope of tangent/normal
- Finding point when tangent is parallel/ perpendicular
- Finding equation of tangent/normal when point and curve is given
- Finding equation of tangent/normal when slope and curve are given
-
Finding approximate value of numbers
- Finding approximate value of function
- Finding approximate value- Statement questions
- Finding minimum and maximum values from graph
- Local maxima and minima
- Minima/ maxima (statement questions) - Number questions
- Minima/ maxima (statement questions) - Geometry questions
- Absolute minima/maxima
Transcript
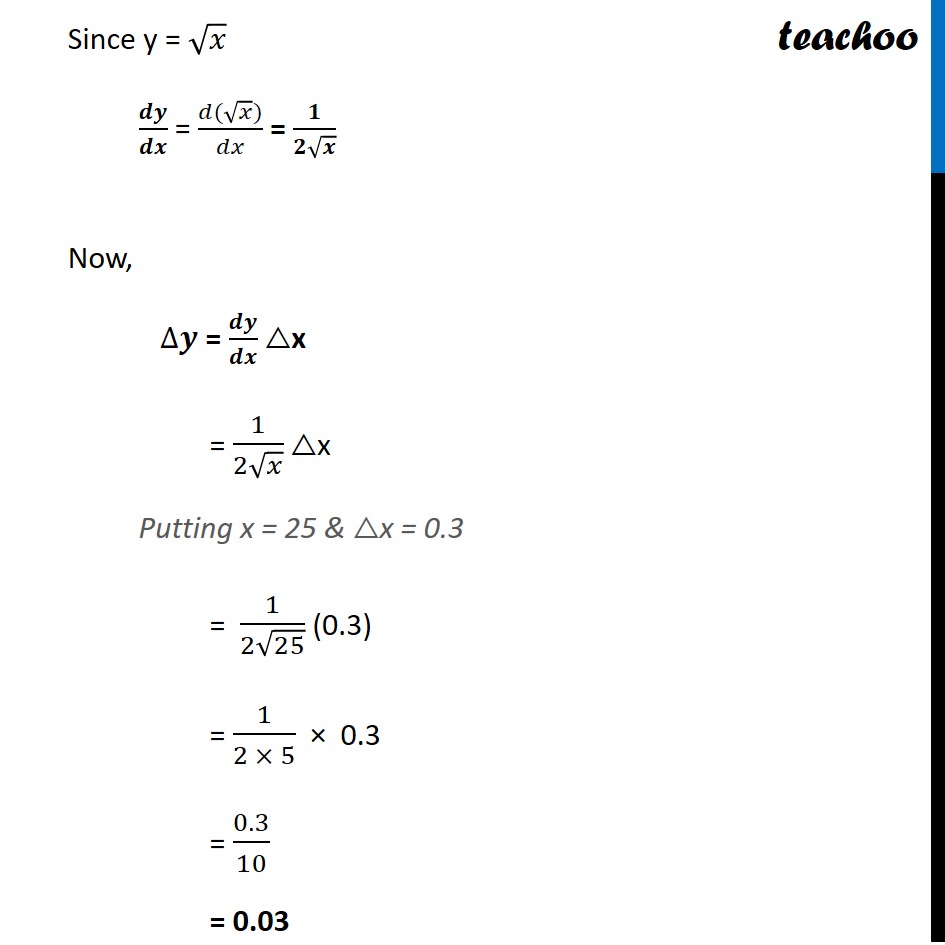
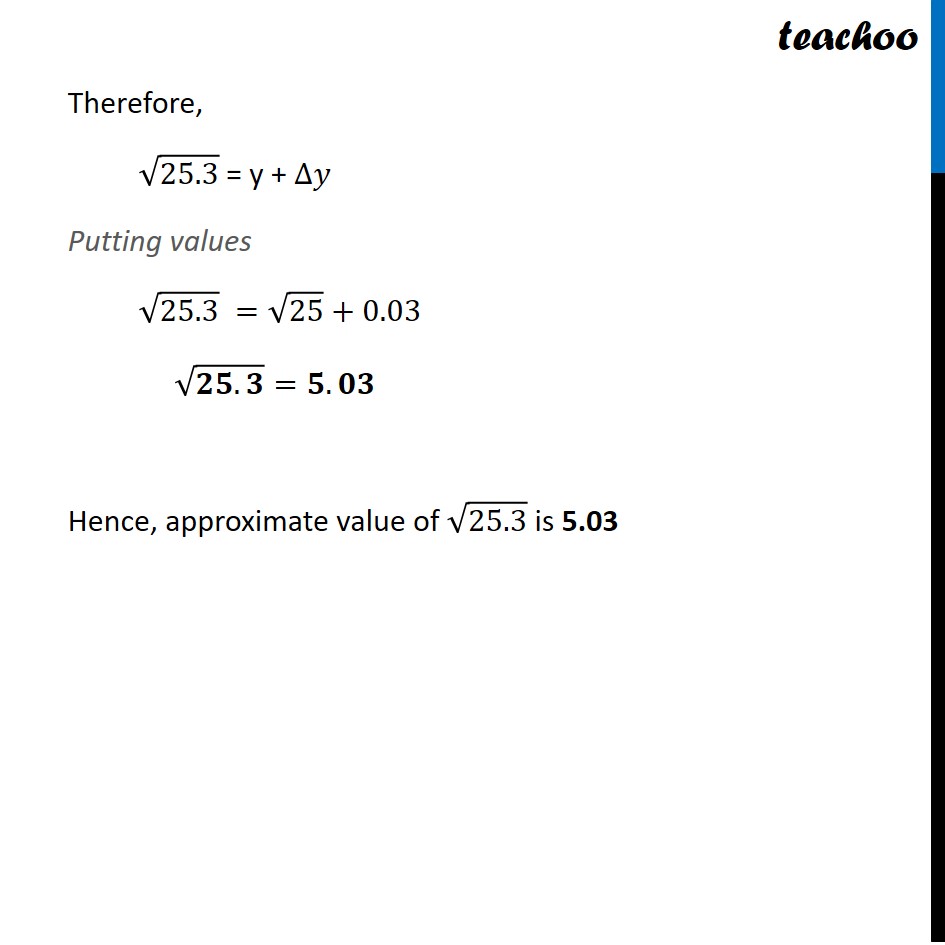
Question 1 Using differentials, find the approximate value of each of the following up to 3 places of decimal. (i) √25.3Let y = √𝒙 Thus, √(𝟐𝟓.𝟑) = y + ∆𝒚 Here, ∆𝒚 = 𝒅𝒚/𝒅𝒙 △x where x = 25 & △x = 0.3 Since y = √𝑥 𝒅𝒚/𝒅𝒙 = (𝑑(√𝑥))/𝑑𝑥 = 𝟏/(𝟐√𝒙) Now, ∆𝒚 = 𝒅𝒚/𝒅𝒙 △x = 1/(2√𝑥) △x Putting x = 25 & △x = 0.3 = 1/(2√25) (0.3) = 1/(2 × 5) × 0.3 = 0.3/10 = 0.03 Therefore, √25.3 = y + ∆𝑦 Putting values √25.3 =√25+0.03 √(𝟐𝟓. 𝟑)=𝟓. 𝟎𝟑 Hence, approximate value of √25.3 is 5.03
