Question 26 (MCQ) - Chapter 6 Class 12 Application of Derivatives (Important Question)

Last updated at April 16, 2024 by Teachoo

Ex 6.3, 26 - Slope of normal to y = 2x2 + 3 sin x at x = 0 is

Ex 6.3,26 - Chapter 6 Class 12 Application of Derivatives - Part 2

Next: Example 23 Important → Go Ad-free

Transcript

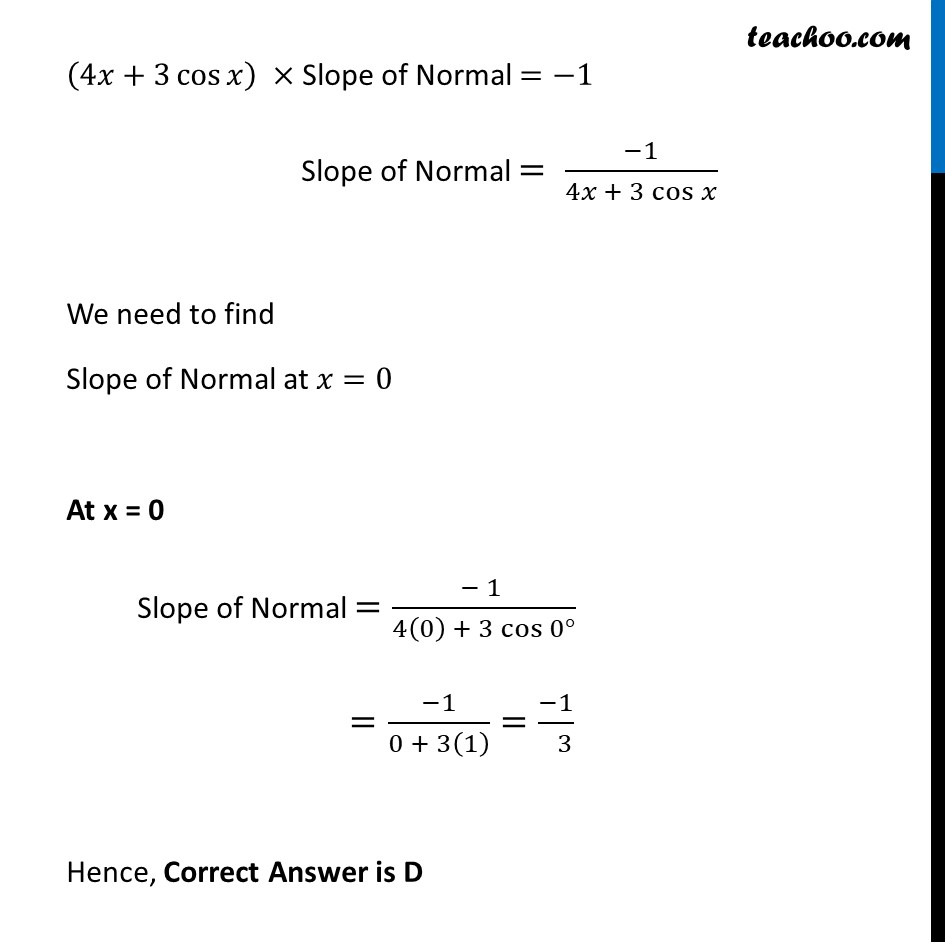
Question 26 The slope of the normal to the curve y = 2x2 + 3 sin x at x = 0 is (A) 3 (B) 1/3 (C) – 3 (D) – 1/3Slope of tangent is 𝑑𝑦/𝑑𝑥 𝑦=2𝑥^2+3 sin⁡𝑥 Differentiating w.r.t. 𝑥 𝑑𝑦/𝑑𝑥=𝑑(2𝑥^2 +3 sin⁡𝑥 )/𝑑𝑥 𝑑𝑦/𝑑𝑥=4𝑥+3 cos⁡𝑥 We know that Slope of tangent × Slope of Normal =−1 (4𝑥+3 cos⁡𝑥 ) × Slope of Normal =−1 Slope of Normal = (−1)/(4𝑥 + 3 cos⁡𝑥 ) We need to find Slope of Normal at 𝑥=0 At x = 0 Slope of Normal =(− 1 )/(4(0) + 3 cos⁡〖0°〗 ) =(−1)/(0 + 3(1) )=(−1)/( 3) Hence, Correct Answer is D

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular