You are here
Misc 13 (MCQ) - Chapter 13 Class 12 Probability
Last updated at Dec. 16, 2024 by Teachoo
Chapter 13 Class 12 Probability
Concept wise
-
Conditional Probability - Values given
- Conditional Probability - Statement
- Multiplication rule of probability
- Independent events
- Basic Probability
- Theorem of total probability
- Bayes theorem
- Random Variable - Defination
- Probability distribution
- Mean or Expectation of random variable
- Variance and Standard Deviation of a Random Variable
- Bernoulli Trials
- Binomial Distribution
Transcript
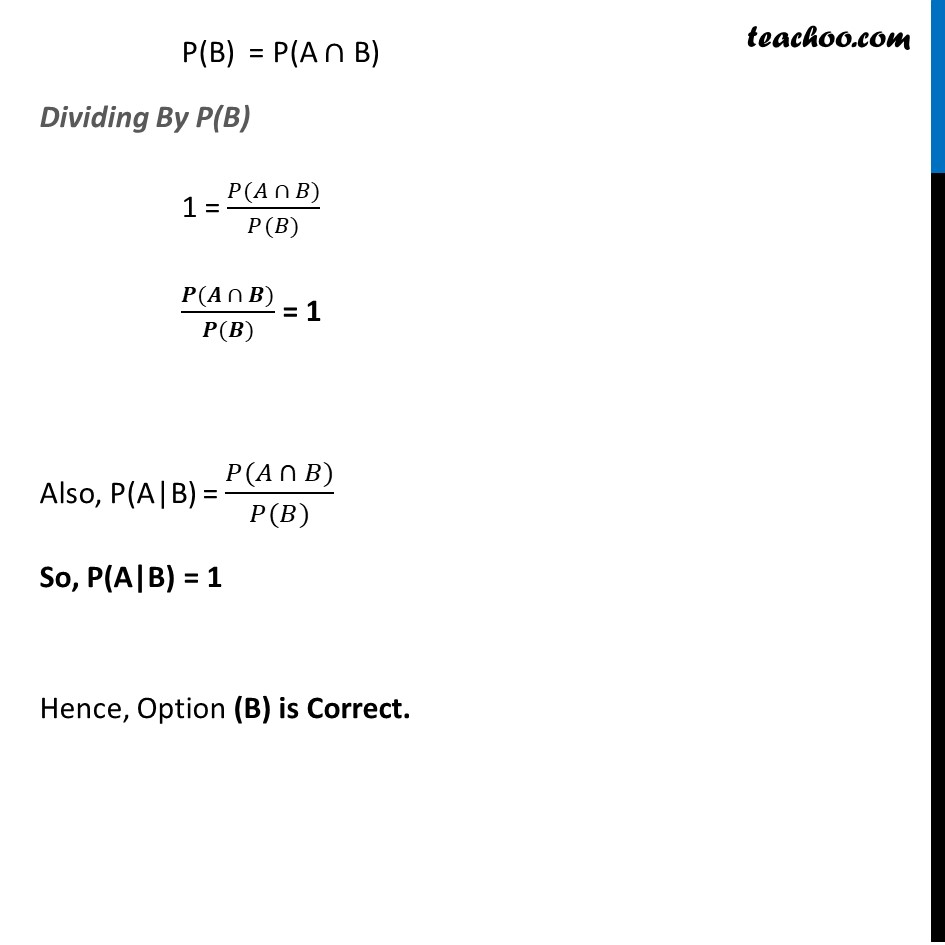
Misc 13 If A and B are any two events such that P(A) + P(B) – P(A and B) = P(A), then (A) P(B|A) = 1 (B) P(A|B) = 1 (C) P(B|A) = 0 (D) P(A|B) = 0 Here P(A and B) = P(A ∩ B) Now, P(A) + P(B) – P(A and B) = P(A) P(A) + P(B) – P(A ∩ B) = P(A) P(B) – P(A ∩ B) = P(A) – P(A) P(B) – P(A ∩ B) = 0 P(B) = P(A ∩ B) P(B) = P(A ∩ B) Dividing By P(B) 1 = (𝑃(𝐴 ∩ 𝐵))/(𝑃(𝐵)) (𝑷(𝑨 ∩ 𝑩))/(𝑷(𝑩)) = 1 Also, P(A|B) = (𝑃(𝐴 ∩ 𝐵))/(𝑃(𝐵)) So, P(A|B) = 1 Hence, Option (B) is Correct.
